Back to main course page

Assignment 11: Relativistic Cosmology

For submission Mon. Nov. 3, Tues. Nov. 4, Wed. Nov. 5.

1. Name a spacetime that has the following properties:

(a) It is uniformly filled with matter that is everywhere at rest.

(b) It is empty of matter but space collapses and then expands everywhere.

(c) It has a special center in the geometry of its space.

(d) It has no matter and no gravitational effects anywhere.

2. (a) What are Einstein's gravitational field equations of 1915? How does Einstein's cosmological constant λ modify them?

(b) Show how the term can be re-interpreted as representing a form of matter in space.

(c) Why is the form of matter odd?

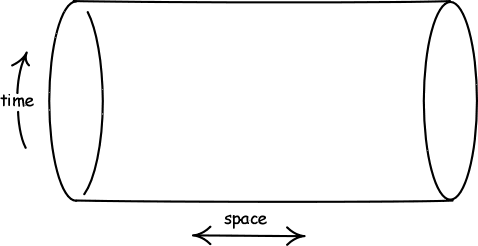
3. Imagine a time-travel, cylinder universe which is empty except for one mass.

(a) Draw in the worldline of the mass when it remains at rest in the space and reconnects with itself.

(b) Draw the worldline of the mass when the mass moves to the right.

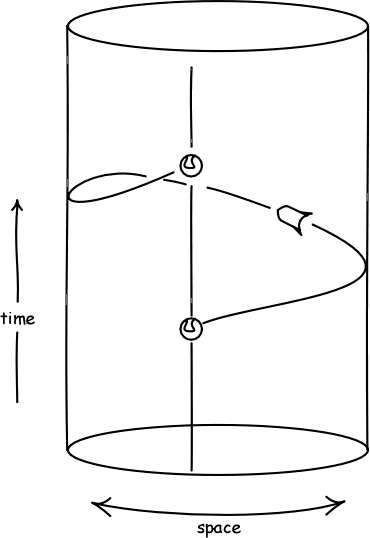
(c) The mass can collide with its future self. The collision is such that the mass gets deflected by just the right amount to come back as the later self of the collision. Draw the worldline that shows this, recalling that aside from collisions the mass moves inertially--i.e. in a straight line in the space.

Hint: Here's a way of resolving collisions in a spacetime diagram. The diagram opposite shows what happens in spacetime when a body A approaches a body B at rest and with equal mass. If the collision is elastic, body A comes to rest and body B moves off the same velocity that A had initially.

For discussion in the recitation.

A. (a) If time travel were possible, the familiar paradox tells us that we could travel back in time, assassinate our grandfather in his youth, thereby precluding our birth. A contradiction ensues, since it now follows both that you traveled back in time and that you did not travel back in time. Good physical theories cannot tolerate contradictions. Does this mean we should abandon any theory that tells us that time travel is possible?

(b) In an old movie, a time traveler enters William Shakespeare's room just at the moment he is writing Hamlet's famous soliloquy. Shakespeare, however, is completely stumped and cannot find the right line. "To be or not to be." the time traveler whispers impatiently in Shakespeare's ear. "An excellent line," Shakespeare exclaims as he dutifully writes it in his manuscript. The puzzle is this: who thought up the line? More generally, is this the same sort of paradox as the "grandfather paradox? Or is there something significantly different about it.

(c) Here's another version of the paradox of (b). A time traveler steals Michelangelo's famous statue of David from its gallery in Florence and transports it back to Michelangelo's workshop in 1501, just as the sculptor is about to start work on the statue. The time traveler kidnaps the sculptor, keeps him trapped for the 3 years needed to sculpt the masterpiece and places the stolen statue in Michelangelo's workshop. When he is released, Michelangelo is too embarrassed to admit that he did not make the statue. Who made the statue?

B. Imagine a Minkowski spacetime wrapped up in one spatial direction. A space traveler synchronizes his clock with one on earth and then leaves earth. The traveler moves inertially eventually coming back to earth without ever changing direction. When the traveler's clock and the earth clock are compared, the traveler's clock will be found to have been slowed by the motion and will read less than the earth clock. Is this a violation of the principle of relativity? Shouldn't the traveler expect the earth clock to have run slower? Note that this version of the "twin" problem is unlike the familiar one in so far as the traveler moves inertially at all times; there is no turning around and thus no acceleration. (Hint: this space has a preferred state of motion! To find it, try drawing in the hypersurfaces of simultaneity of the earth and of the spacetraveler.)