How Did Einstein Think?

John D. Norton
Department of History and Philosophy of Science
and Center for Philosophy of Science
University of Pittsburgh
http://www.pitt.edu/~jdnorton

This page at http://www.pitt.edu/~jdnorton/Goodies

Prepared to accompany
cityLIVE! "everything einstein"
November 15, 2007, 6:30 pm
The New Hazlett Theater, Pittsburgh PA
Panelists: John D. Norton, Walter Isaacson
Moderator: Regina Schulte-Ladbeck

What is it...?

What is it about Einstein that we find so fascinating? One side is that his thought and achievement seems so far above our mundane sphere that we are inspired merely reflecting on him. There is a second side to this fascination. It lies, I believe, in the thought that we are in the end not so far removed from him. While his achievements are transcendent, he was in the end simply human. He ate soup and paid taxes like the rest of us. He tried to keep ahead of the mundane practicalities of life before they submerged him. He didn't wear socks and thought just one soap for washing and shaving was quite enough.

It is that second sense that has always fascinated me. What Einstein did, he did using tools available to all of us. He had no magic wand or secret subscription to Encyclopedia Galactica, where all the truths of nature are written. He used tools and methods available to everyone. He read the same text books and journals available to every scientist of his day. His principal tool was a notepad with a pen and pencil. He read and wrote and calculated and thought; and out poured his extraordinary achievements.

In this regard, Einstein is part of an extraordinary tradition of achievement in science that extends to antiquity. I am equally fascinated by the ancient astronomers. Look around you. How could you figure out that the surface of the Earth is spherical--not just curved, but a surface of constant curvature in all directions? The ancient astronomers did it with nothing more than patient observation, pointed sticks and ingenious thought. Well, perhaps I am a little more fascinated with Einstein than the ancient astronomers.

My purpose here is to say something about how Einstein worked and thought. I've written a lot elsewhere on the details of Einstein's discoveries. Now I want to try to get behind them and understand better the thinking that led Einstein to them.

Einstein's Answer

What did Einstein have to say about his own thinking? We have an answer. Jacques Hadamard was a French mathematician who approached Einstein with questions on Einstein's thought processes when Hadamard was preparing a psychological survey on the internal mental worlds of mathematicians. Einstein's answer is reproduced more fully at right. It is somewhat unrevealing. Einstein struggles as would anyone trying to describe what transpires in their consciousness. For example, to begin with, words and language do not play any role in his thoughts. They must be sought for laboriously later. This is an experience familiar to many of us who find our thoughts racing ahead of our power to express them. It doesn't seem to capture anything distinctive about Einstein's thinking.

Here's a longer extract from Einstein's answer: "(A) The words or the language, as they are written or spoken, do not seem to play any role in my mechanism of thought. The psychical entities which seem to serve as elements in thought are certain signs and more or less clear images which can be "voluntarily" reproduced and combined. There is, of course, a certain connection between those elements and relevant logical concepts. It is also clear that the desire to arrive finally at logically connected concepts is the emotional basis of this rather vague play with the above-mentioned elements. But taken from a psychological viewpoint, this combinatory play seems to be the essential feature in productive thought--before there is any connection with logical construction in words or other kinds of signs which can be communicated to others. (B) The above-mentioned elements are, in my case, of visual and some of muscular type. Conventional words or other signs have to be sought for laboriously only in a secondary stage, when the mentioned associative play is sufficiently established and can be reproduced at will. (C) According to what has been said, the play with the mentioned elements is aimed to be analogous to certain logical connections one is searching for. (D) Visual and motor. In a stage when words intervene at all, they are, in my case, purely auditive, but they interfere only in a secondary stage, as already mentioned. (E) It seems to me that what you call full consciousness is a limit case which can never be fully accomplished. This seems to me connected with the fact called the narrowness of consciousness (Enge des Bewusstseins)" From "A Mathematician's Mind, Testimonial for An Essay on the Psychology of Invention in the Mathematical Field by Jacques S. Hadamard, Princeton University Press, 1945." in Ideas and Opinions.

Perhaps we should heed Einstein's advice:

"If you want to find out anything from the theoretical physicist about the methods they use, I advise you to stick closely to one principle: don't listen to their words, fix your attention on their deeds."
"On the Methods of Theoretical Physics," Herbert Spenser Lecture, Oxford, June 10, 1933.

What can we learn about Einstein's thoughts and methods by looking at his work? I'll sharpen my queries in a series of questions that span from the general and big to the narrow and specific.

Inscrutable Flash of Insight
or
Systematic exploration?

Let us start with the biggest question first. When Einstein made his big discoveries, how did they happen? Did they come in momentary flashes of insight, the product of hidden processes that suddenly flood the mind with their perfected insight? Or did they come through slow labor, much as we might build a tall tower by patiently and systematically piling the bricks one upon another?

Einstein's major discoveries seem to have both in them. Let us consider two discoveries.

Special Relativity Take Einstein's 1905 discovery of the special theory of relativity. This is the theory for which he is best known. It says that moving rods shrink, moving clocks slow and that the speed of light is a fundamental barrier through which nothing can accelerate. The decisive moment in the discovery of the theory came after Einstein had become convinced of two apparently contradictory results. One was the principle of relativity that tells us that uniform motion has no effect on physical systems. The second--soon to be called the light postulate--asserted that the speed of light always came out to be the same number, 186,000 miles per second. How could he have both? If we chase after a beam of light, surely we must judge it slow down? The decisive insight, Einstein later recalled, came after a visit with his friend Michele Besso, when the two discussed his work. Both are possible, Einstein realized, if we give up an assumption about simultaneity. We had assumed that whether two events at different places are simultaneous is an absolute fact. It is not, Einstein now saw. Observers in relative motion will disagree on whether two events are simultaneous. What if those events are used to time how fast light goes, say how long it needs to traverse some measuring rod? Then those differing judgments of simultaneity will make the seemingly impossible, possible. The original observer and the one chasing after light will both judge the light to have the same speed. The rest was mechanical. Einstein needed only 5 to 6 weeks to write what is now often singled out as the greatest scientific paper of the 20th century, "On the Electrodynamics of Moving Bodies." So it was all in that moment? Hardly. The real work lay in the reflections that convinced Einstein of the two apparently contradictory commitments. That work took, by Einstein's own reckoning, seven and more years. From his student days, Einstein had been fascinated by the latest, hottest theory of the moment, Maxwell's electrodynamics. It is a theory of electricity, magnetism and the waves that propagate in these fields; it was the closest the time had come to a theory of everything. One of its most striking results was that light is just a wave of propagating electric and magnetic fields and that it has a single, definite speed, 186,000 miles per second. Maxwell's theory was based on an ether with a preferred state of rest. The problem Einstein found was a tension between this preferred state of rest and the failure of experiments to reveal it. Worse on his reading the theory seemed to predict that no experiment could reveal it. So Einstein pursued the only line of research that seemed promising, attempts to modify Maxwell's theory in a way that would do away with the ether state of rest. It was a long and systematic search that went nowhere. The more he tried, the worse it got. Einstein came to a crisis. All efforts to modify Maxwell's electrodynamics had failed. But how could he keep both the idea that there is no ether state of rest and also the celebrated result of Maxwell's theory that light travels at exactly 186,000 miles per second. Then an observer chasing a light beam would not find the light to slow. These long efforts prepared the ground for Einstein's celebrated visit to Michele Besso.

General Relativity We see this same mix of flash of insight and systematic construction in the case of Einstein's greatest discovery, the general theory of relativity. This is the theory that tells us that gravity is just a curvature of the geometry of spacetime. It tells us that the real geometry of space is not the one Euclid described millennia ago and opens the door for later researchers to posit black holes and other extraordinary pathologies of space and time. The flash came in Einstein's first step. In 1907, while still a patent clerk, he was pondering how one might produce a relativistic theory of gravity and he was not having much success. Then he was struck by the fact that an observer in free fall no longer feels his own weight. He then hit upon what he called "the happiest thought of my life." One can produce gravity in gravity free space merely by reversing the process. Acceleration creates a gravitational field. This is his "principle of equivalence." That thought was the first step. It took seven and more years for Einstein to complete this work in 1915. Its first phase was devoted to systematic constructions that derived directly from Einstein's "happiest thought." In it, acceleration produced a special case of a relativistic gravitational field. Einstein now turned to the task of cataloguing the properties of this special case and generalizing them to arrive at a more general theory. In the special case, clocks are slowed and light is deflected by the gravitational field, which is proportional to the a variable speed of light. Those properties, Einstein supposed, hold in all static gravitational fields and he could arrive at the description of these fields merely by slightly generalizing the ways that the speed of light could vary with position in space. These constructions occupied Einstein's efforts in gravitation theory up to mid 1912. In the year that followed, Einstein made the transition to a spacetime theory in which gravitation is related to the curvature of its geometry. Unlike the case of special relativity, we have been able to reconstruct in some detail the steps and missteps of this decisive advance. We have a series of publications documenting the various stages of the developing theory and even his private notebook calculations, which I have spent a great deal of time in reconstructing. Einstein's explorations went quickly and slowly, straight and meandering. At times they became almost recipe-like. Einstein laid out the requirements his final equations must meet and then systematically searched for equations that satisfied them. While Einstein was launched by a flash of insight, the greatest part of work on the theory was spent in this systematic exploration. That eventually brought his project to completion.

Unfettered Speculation
or
Controlled Flight?

Einstein did have decisive flashes of inspiration. What was their character? Were they just wild moments of unfettered speculation? Or were they controlled? The first fits with the image of Einstein's scientific creativity as resulting from a liberation from reason. The second fits with it as a more profound fulfillment of reason.

My view is that his flights fit much better with the second. In the two cases we have just seen, the moment of inspiration was seeded by earlier work and directly responded to it.

The insight over the relativity of simultaneity came after years of struggle with failed efforts to reconcile the principle of relativity with Maxwell's electrodynamics. He did not so much choose to leap into the unknown as he was pushed by the accumulated pressure of those problems.

Things were similar but not so acute with the "happiest thought of [his] life," the principle of equivalence. It emerged after a series of failed attempts at finding a relativistic theory of gravitation. All the candidates he thought up failed to fit a simple expectation about gravity that he traced back to Galileo: all things big and small must fall with the same acceleration. The virtue of the gravitational field created by acceleration was that it was assured to have exactly this property.

We find the same control in another of Einstein's legendary leaps. In 1905, in the first of his papers of his annus mirabilis, Einstein proposed that light energy might not be distributed over space, as the wave theory supposed. Rather the energy of high frequency radiation was localized into pointlike quanta whose positions in space are independent of one another, rather like the molecules of an ideal gas. The idea was quite extraordinary. The great achievement of nineteenth century physics had been Maxwell's electrodynamics and its proudest moment had come when it had shown that light was merely an electromagnetic wave. As the twentieth century began, no result seemed more secure than the wave character of light. Yet here was Einstein contradicting it. Did a departure this radical result from unfettered speculation that yielded some blinding flash of insight? How else could it arise? We have no autobiographical recollections from Einstein on the moment at which he hit upon this remarkable idea. However we do have plenty of clues from his published papers over how he might have arrived at it. All those clues (surveyed at right) point to "controlled flight."

The Light Quantum While Maxwell's electrodynamics was reigning supreme, Einstein had recognized that it faced a serious problem. The theory was supposed to give an account of heat radiation, the sort of radiation we warm our hands with in front of a roaring fire. Heat energy is really just a jumbled distribution of energy. The energy is distributed randomly over many molecules, if the heat is in a gas, or over many frequencies of light, if we have heat radiation. A standard part of this theory of heat is that the distribution moves towards the most probable distribution, which becomes its equilibrium distribution. What Einstein recognized and laid out clearly in the first part of his 1905 light quantum paper was that this essential equilibrium distribution did not exist in Maxwell's electrodynamics (or, more precisely, that it required an impossible, infinite energy density). It was an early, clear statement of what Ehrenfest later called "the ultraviolet catastrophe." Einstein recalled in his Autobiographical Notes that he had known of these sorts of problem very early. But why react to these problems by doing away with the founding idea of the wave theory of light, that the energy of light is distributed in space? Why not choose a less severe mutilation of the theory? Planck, for example, came to favor the idea that the whole problem could be solved by tinkering with our assumptions about how light interacts with matter. There was no need to change essentially our conception of light or matter individually. Einstein had a specific reason for expecting that this sort of cautious tinkering would fail. When one looks at fluids like air or water, there is no apparent indicator at human scales that they consist of very many molecules. More precisely, that is true as long as one doesn't know what indicators to look for. Einstein did know. In the years leading up to 1905, he had been systematically investigating the macroscopic properties of such systems in order to discern their microscopic constitutions. In that process, he had become very familiar with the macroscopic signatures of particular microscopic constitutions. The simplest signature belonged to ideal gases. That a gas obeys the ideal gas law macroscopically turns out to be an assurance that it consists microscopically of many, spatially localized, independent component molecules. That signature could be used elsewhere. The solutes of dilute solutions exert an osmotic pressure that obeys the ideal gas law; that reveals to us that the solute consists of many, spatially localized components that do not interact with each other. In 1905, Einstein found a form of this same signature in high frequency heat radiation. So he could infer that the energy of heat radiation has the same macroscopic properties as an ideal gas or a dilute solution. The analysis of that signature comprised the core argument of Einstein 1905 light quantum paper; and the inference from it that the energy of heat radiation lives in quanta is presented as his light quantum hypothesis.

Thinking Mathematically
or
Thinking Physically?

What sort of theorist was Einstein? Everyone knows that he brought profound insight in the form of new theories in mathematical physics. If we seek the origin of that thought, should we put the emphasis on the "mathematical" or the "physical"? The distinction between the two is one that Einstein himself drew and reflected upon.

In thinking mathematically, or, as Einstein's sometimes said, formally, one takes the mathematical equations of the theory as a starting point. The hope is that by writing down the simplest mathematical equations that are applicable to the physical system at hand, one arrives at the true laws. The idea is that mathematics has its own inner intelligence, so that once the right mathematics is found, the physical problems melt away. Philosophers will recognize this as a form of Platonism.

In thinking physically, one proceeds quite differently. The starting point is physical. Perhaps it lies in an examination of the results of experiments; or in an insistence on certain founding physical principles, like the principle of relativity of the conservation of energy and momentum; or perhaps it draws on deeper, more visceral ideas of what can and cannot happen in real physical situations.

Einstein's theories sometimes made special mathematical demands on physicists. His great achievement, the general theory of relativity, required physicists to learn what we now call "tensor calculus," which proved something many found formidable. But it was not mathematics that drove Einstein's theoretical successes.

Einstein's enduring achievements in physics were virtually all a product of the earlier part of his life: special relativity, Brownian motion that shows the reality of atoms, the light quantum, general relativity, the "A and B" coefficients paper that grounds lasers. All this was done before he turned 40. Virtually all of these achievements depended upon a very astute form of physical thinking. Einstein then dressed it in mathematical clothing, seeking where ever possible to keep the mathematics as simple as he could.

Just how did Einstein's physical insight work? One part was an keen instinct as to which among the flood of experimental reports were truly revealing. Another was his masterful use of thought experiments. Through them Einstein could cut away the distracting clutter and lay bare a core physical insight in profoundly simple and powerfully convincing form.

Here's an example of a thought experiment from Einstein's work on general relativity already mentioned above. Einstein realized at the start that we needed to think differently about the empty space of special relativity if we were to arrive at an acceptable relativistic theory of gravity. We needed to stop thinking of it as the gravitation free case. It is actually the simplest case of a gravitational spacetime. His claim, the principle of equivalence, was that uniform acceleration in empty space produces a gravitational field.

But how can that be made intelligible? Einstein's approach was simple and ingenious. He had us to imagine a physicist who is drugged and placed inside a box. That box is transported to a distant part of space where it is accelerated uniformly by some agent. The phyicist wakes up. Any object released by the physicist would accelerate to one part of the box. The acceleration would be the same for all objects released, be they small or large in mass. That is the distinctive property of gravity: it accelerates all masses alike. Could the physicist know that the box is accelerated unformly in space and not at rest in a homogenous gravitational field? No, Einstein asserted, there is no way. There is no difference between the two cases. The result of the acceleration is to create a gravitational field inside the box.

Einstein himself recognized this special facility for physical thinking when he wrote his Autobiographical Notes (see right), reporting that this physical insight and intuition outstripped his mathematical instincts. There is, however, a unexpected ending to the story that also surfaces in these remarks. Einstein's general theory of relativity derived essentially from physical thinking. How could he devise a theory of gravity that frees us from our dependence on any special coordinate systems? How could acceleration be deprived of its absoluteness in such a theory? However the theory grew so complicated and difficult that Einstein's physical instincts failed him at the crucial moment. In 1913 he published a defective version of the theory. After nearly three years of hesitation and doubt, he was able to repair the theory by writing down the mathematically simplest equations. They were ones he'd nearly adopted three years before. He had then thought them to be inadmissible for physical reasons. The diagnosis of his earlier error was clear. He had mistakenly trusted his physical insight over mathematical simplicity.

"The fact that I neglected mathematics to a certain extent had its cause not merely in my stronger interest in the natural sciences than in mathematics but also in the following peculiar experience. I saw that mathematics was split up into numerous specialties, each of which could easily absorb the short lifetime granted to us. Consequently, I saw myself in the position of Buridan's ass, which was unable to decide upon any particular bundle of hay. Presumably this was because my intuition was not strong enough in the field of mathematics to differentiate clearly the fundamentally important, that which is really basic, from the rest of the more or less dispensable erudition. Also, my interest in the study of nature was no doubt stronger; and it was not clear to me as a young student that access to a more profound knowledge of the basic principles of physics depends on the most intricate mathematical methods. This dawned upon me only gradually after years of independent scientific work. True enough, physics also was divided into separate fields, each of which was capable of devouring a short lifetime of work without having satisfied the hunger for deeper knowledge. The mass of insufficiently connected experimental data was overwhelming here also. In this field, however, I soon learned to scent out that which might lead to fundamentals and to turn aside from everything else, from the multitude of things that clutter up the mind and divert it from the essentials." Albert Einstein, Autobiographical Notes.

"Can we hope to be guided safely by experience at all when there exist theories (such as classical mechanics) which to a large extent do justice to experience, without getting to the root of the matter? I answer without hesitation that there is, in my opinion, a right way, and that we are capable of finding it. Our experience hitherto justifies us in believing that nature is the realization of the simplest conceivable mathematical ideas. I am convinced that we can discover by means of purely mathematical constructions the concepts and the laws connecting them with each other, which furnish the key to the understanding of natural phenomena." "In a certain sense, therefore, I hold it true that pure thought can grasp reality, as the ancients dreamed." Albert Einstein, "On the Method of Theoretical Physics," The Herbert Spenser lecture, delivered at Oxford, June 10, 1933.

The experience triggered a profound change in Einstein's thinking. He now became convinced that the path to a deeper understanding of the physical world lay in mathematics. Einstein's conversion is expressed most eloquently in his own words from a lecture given in 1933 (left). Here Einstein has adopted a form of Platonism. What makes his adoption of it extraordinary is that he believed it was a lesson learned from his own experience of physical theorizing.

Geometric
or
Algebraic Thinking?

Einstein's work continued to depend on mathematics and the mathematics he employed grew more as time passed. There are different ways of using mathematics to clothe a theory and Einstein adopted a particular stance towards them. The two ways I will describe will be recognized by anyone who has had a high school exposure to mathematics.

One approach is geometrical. The most familiar expression of it is Euclid's geometry. We pursue geometrical structures there by drawing lines and curves and seeing how they intersect. Take a parabola, for example. Does it have mirror symmetry about the vertical axis shown at right as a dotted line? To check, we have to find out whether flipping the figure over the dotted line makes a difference. It does not change the curve at all, so we infer that the parabola does indeed display this mirror symmetry.

Another approach is algebraic. This is a way of doing mathematics that concentrates on writing symbolic expressions and manipulating them. "A fish is 10 inches long plus half of itself. How long is the fish?" We say. "Let x be the length of the fish. Then x = 10 + x/2. Solving, we find x=20. The fish is 20 inches long."

Here's another problem. Take the formula y=x². Is it changed when we replace x by -x? A quick calculation shows that it is not. y=x² becomes y=(-x)² which is just y=x² again since (-x)² = x².

This last problem is purely one of symbolic algebraic manipulation. Yet it is really just the same the problem as the symmetry of the parabola. If x and y are ordinary Cartesian coordinates the y=x² is the formula for a parabola. Replacing x by -x is just the operation of reflection over the y axis. So when the formula y=x² stays the same under this transformation, we have the algebraic equivalent of the demonstration of the mirror symmetry of the parabola.

We see the difference of approach expressed directly in physics. Consider special relativity. Most treatments of the theory rapidly seek to develop the notion of spacetime. Through it one learns a wonderfully simple way to conceive the theory. All of space and time taken together form a single spacetime. Special relativity is really just the theory of this spacetime's geometry. One uses the theory by drawing straight lines and hyperbolas and forming geometric constructions similar to those we learned in elementary geometry classes. The principle of relativity is expressed as a sort of isotropy of the spacetime, somewhat akin the isotropy of a Euclidean space--that all its directions are the same.

That geometrical way of conceiving special relativity is not Einstein's. It was devised by the mathematician Hermann Minkowski shortly after Einstein published his special theory of relativity. Einstein was reluctant to adopt Minkowski's method, thinking it smacked of "superfluous learnedness." It was only well after many others had adopted Minkowski's methods that Einstein capitulated and began to use them. It was a good choice. It proved to be an essential step on the road to general relativity.

Einstein preferred to think of his theory in terms of the coordinates of space and time: x, y, z and t. The essential ideas of the theory were conveyed by the algebraic properties of these quantities, treated as variables in equations. Its basic equations are the Lorentz transformation, which, in Einstein's hands, is a rule for changing the variables used to describe the physical system at hand.

The laws of physics are written as symbolic formulae that include these coordinate variables. The principle of relativity of relativity then became for Einstein an assertion about the algebraic properties of these formulae; that is, the formulae stay the same whenever we carry out the symbolic manipulation of change of variables of the Lorentz transformation.

The emphasis in Einstein's algebraic approach is on variables, not spacetime coordinates, and formulae written using those variable, not geometrical figures in spacetime.

For many purposes, it makes no difference which approach one uses, geometric or algebraic. Sometimes one is more useful or simpler than the other. Very often, both approaches lead us to make exactly the same calculations. We just talk a little differently about them.

However there can be a big difference if we disagree over which approach is more fundamental. We now tend to think of the geometric conception as the more fundamental one and that Einstein's algebraic formulae are merely convenient instruments for getting to the geometrical properties.

There is some evidence that Einstein saw things the other way round. He understood the geometric conception, but took the algebraic formulation to be more fundamental. A simple example illustrates how this difference can matter a lot.

Take a two dimensional Euclidean space with some origin point O. We will consider all the straight lines that pass through it. An elementary fact of Euclidean geometry is that all these lines are exactly the same. None is special geometrically. There is another way to investigate the geometry of a Euclidean space and the lines in it. That is to label all its points with coordinates. We usually use x and y and label the origin point O with the values x=0 and y=0. Then a straight line through the origin O is given algebraically by the formula "y=mx," where m can have any real value.

So consider the sequence of formulae generated as m varies: y=0, y= (1/2)x, y=2x, y=3x, y=4x, ... and so on. As m gets bigger, the straight lines described get closer and closer to a vertical line through O. Our geometric intuitions tell us that this is where the sequence of formulae must be heading. But they do not go there. When m gets to be infinitely big, we get the formula "y=infinity." It not at all clear what that represents. It is not a line passing through O since all lines passing through O must pass through x=0, y=0. These values do not satisfy the formula "y=infinity."

One could take two attitudes to this little oddness. One could think geometrically. One could say that what we are really talking about are just straight lines in Euclidean space. So the limit of the sequence is just a vertical line. What has gone wrong is that algebraic device for representing lines as formulae has "gone bad" in this limiting case. So we shrug the whole thing off as an inadequacy in the algebraic representation.

One might also think algebraically and take the formulae to be fundamental. Then one would say that the set of structures represented themselves go bad in the limit. That is, they are "singular" to use the fancier term when infinities like this appear. That amounts to saying that something odd is afoot as we approach the limit of the sequence.

Of course it is not mandatory to take the geometrical approach as fundamental, that is, the one that is used to correct the algebraic when they disagree. One can imagine circumstances in which it would go the other way. Imagine, for example, that the variable y represents the distance traveled by a particle over time x once it has been ejected from some atomic event at speed m. The formulae y=1/2x, y=x, y=2x, ... represent the cases of the particle being ejected at faster and faster speeds. We could represent these by the corresponding straight lines on a graph. What of the limit of the sequence, y=infinity? It represents an occurrence we might plausibly judge as unreasonable. It is the ejection of the particle with infinite speed so that it instantly is moved "to infinity" or, in other words, is instantly no longer in the space at all. In this scenario, we might well judge the limit of sequence to be a singular process that we do not expect to arise in the world. We would explain away the vertical line in the graph as an oddity of the graph that does not represent anything real.

We would probably now choose to think geometrically. There is clear evidence that Einstein thought algebraically about these cases. This case of straight lines is a simple one I concocted as an illustration. Einstein never spoke of it. There are, however, more complicated cases in which Einstein clearly favored the algebraic representation with its singularities over the regular geometric representation.

One arose in the later 1910s shortly after the birth of relativistic cosmology. Einstein became engaged in a dispute with de Sitter and others over a new cosmological spacetime explored by de Sitter.

In geometrical terms, the de Sitter spacetime is a hyperboloid of revolution in a five dimensional Minkowski spacetime. Fortunately, this somewhat intimidating technical specification is not needed to understand the essential point. De Sitter spacetime is completely homogeneous. Every point is geometrically just like every other. It also has no matter in it.

That can readily come about. To see how, imagine a two dimensional sphere in a three dimensional space. Its surface is homogeneous. Every point is geometrically like every other. De Sitter's hyperboloid is actually a very close geometrical analog of this sphere in the context of relativistic spacetimes.

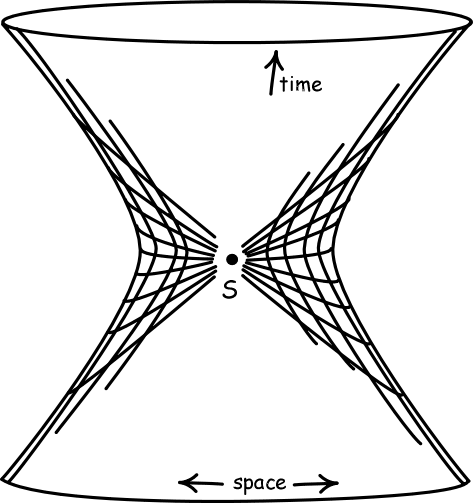As usual, Einstein used coordinate labels to describe the properties of this spacetime. The coordinate system he used, however, had the awkward property of not covering the surface properly. It left some parts out and, worse, "went bad" on an entire spherical surface enclosing the region coordinatized. In the figure at left, the coordinate system "goes bad" at the point marked S where the spatial coordinate lines cross. If the figure included all the three dimensions of space, this point would be the surface of a sphere.

Thinking geometrically, we would just say that there is no pathology on this surface. It is just that our coordinates used to describe the surface at those points are not working well any more. How could it be otherwise, we might wonder, since all points in the spacetime are the same geometrically.

Einstein did not say this. He took the algebraic structures arising from this coordinatization to be fundamental and insisted that there is a singularity in the spacetime on this sphere. He used a computation by the mathematician Hermann Weyl to suggest that the singularity somehow harbored masses that would act like gravitational source masses for the whole spacetime.

What attracted Einstein to this possibility was his fascination with an idea he attributed to Ernst Mach and called "Mach's Principle." The principle required that spacetime structure must be fixed fully by matter. If de Sitter spacetime has well defined spacetime structure but no matter in it, then it violates Mach's Principle. That matter might somehow linger in the singularity was a convenient way for Einstein to rescue Mach's Principle.

For Einstein, the algebra trumped the geometry and he found pathologies where we now think there are none.

In Sum

The transcendent achievement of Einstein required many components. He needed an intellect with singular powers. He needed a dedication to hard work. And he needed a commitment to finding the right answer, no matter how hard the path became.

We can learn just a little more from this glimpse of how Einstein thought. For Einstein had quite particular strengths. They lay in an acute physical intuition that guided him to the fertile physical ideas and revealing experimental results. As long as Einstein used those particular skills to drive his work, he produced one great success after another. He was the right thinker, with the right abilities, in the right place and time.

Then Einstein changed his thinking. He was no longer guided so much by physical intuition as by mathematical simplicity. As that change set in, Einstein's work began to languish. He retreated more and more into a private world in which he spent decades searching for his unified field theory. While he did that, the mainstream of physics moved on to elaborate the quantum theory. Einstein did not follow. His physical instincts told him that this was not the fundamental theory; that was to be found by the mathematical path.

We might lament that Einstein's work took this pathway. What might have happened had he continued to follow his older methods? We cannot know. However I suspect that not much would have emerged. Einstein's physical instincts were the ones needed to develop relativity theory and his other successes.

When the focus of research moved to quantum theory, a different sort of instinct seemed to be required. That was embodied by the Danish physicist Niels Bohr. He had a characteristic tolerance and even delight in contradiction. That characteristic enabled Bohr to theorize successfully in the bewildering and uncertain quantum domain and in a way that Einstein's physical sensibilities found repugnant. Einstein's role changed to that of a senior sage, warning the new generations of the dangers of the path they had chosen.