|
John D. Norton
Maxwell's Demon, Landauer's Principle and
the Thermodynamics of Computation
|
|
home >> research
>> Maxwell's Demon... |
|
|
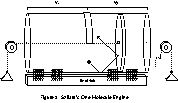
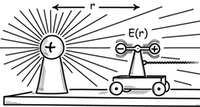
Maxwell's demon is a fictitious, miniscule being imagined by
Maxwell as able to reverse the second law of thermodynamics by
manipulating individual molecules. In a tradition of work initiated
by Szilard in the 1920s, it has become standard to predict the
failure of the demon on information theoretic grounds through a
connection supposed to obtain between information processing and
entropy dissipation. In a study with John Earman, we have suggested
that this account of the demon's failure is either based on question
begging or groundless supposition. |
With John Earman, "Exorcist XIV: The Wrath of
Maxwell's Demon." Studies in the History and Philosophy of Modern
Physics, Part I "From Maxwell to Szilard" 29(1998), pp.435-471; Part
II: "From Szilard to Landauer and Beyond," 30(1999), pp.1-40. Download.
|
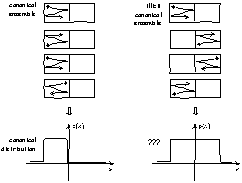 |
The present orthodoxy holds that Maxwell's demon must fail to
reverse the second law of thermodynamics because of a hidden entropy
cost in the erasure of information. The analysis is based on
Landauer's principle, which asserts that the erasure of n bits of
information is accompanied by the passage of least k ln n of entropy
to the surroundings. I argue that Landauer's principle is based on
the formation of illicit canonical ensembles in statistical physics
that give the illusion of the necessity of this entropy cost. I also
urge that, even if the principle were correct, the literarure seeks
to establish that it must defeat all Maxwell demons by the inadquate
means of merely displaying a few suggestive examples.
|
"Eaters of the Lotus: Landauer's Principle and the
Return of Maxwell's Demon." 36 (2005), pp. 375-411. Download
|
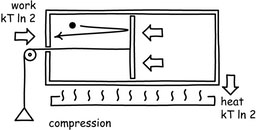 |
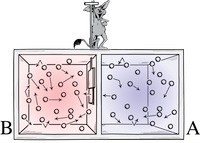
Landauer's Principle asserts that there is an unavoidable cost in
thermodynamic entropy creation when data is erased. It is usually
derived from incorrect assumptions, most notably, that erasure must
compress the phase space of a memory device or that thermodynamic
entropy arises from the probabilistic uncertainty of random data.
Recent work seeks to prove Landauer’s Principle without using these
assumptions. I show that the processes assumed in the proof, and in
the thermodynamics of computation more generally, can be combined to
produce devices that both violate the second law and erase data
without entropy cost, indicating an inconsistency in the theoretical
system. Worse, the standard repertoire of processes selectively
neglects thermal fluctuations. Concrete proposals for how we might
measure dissipationlessly and expand single molecule gases
reversibly are shown to be fatally disrupted by fluctuations. |
"Waiting for Landauer," Studies in History and
Philosophy of Modern Physics, 42(2011), pp. 184-198. Download.
For my reply to Ladyman and Robertson's reply to this paper, see
"Author's Reply to 'Landauer Defended'," Studies in History and
Philosophy of Modern Physics, 44 (2013), p.
272. Download.
See also Goodies pages:
When a Good
Theory meets a Bad Idealization: The Failure of the Thermodynamics
of Computation.
No Go Result
for the Thermodynamics of Computation
For the latest and best developed version of the "no go" result, see
"All Shook Up..." below. |
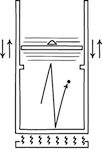 |
Entropy creation in excess of that tracked by Landauer's principle
is needed to overcome fluctuations in molecular scale computation.
This paper is a short account of the "no go" result reported in "Waiting
for Landauer." |
"The End of the Thermodynamics of Computation: A No
Go Result."Philosophy of Science. 80,
(2013), pp. 1182-1192. Download.
For the latest and best developed version of the "no go" result, see
"All Shook Up..." below. |
 |
Brownian computers are supposed to illustrate how logically
reversible mathematical operations can be computed by physical
processes that are thermodynamically reversible or nearly so. In
fact, they are thermodynamically irreversible processes that are the
analog of an uncontrolled expansion of a gas into a vacuum. |
"Brownian Computation is Thermodynamically
Irreversible." Foundations of Physics. 43
(2013), pp 1384-1410.Download.
"On Brownian Computation" International Journal of Modern
Physics: Conference Series. 33 (2014), pp. 1460366-1 to
1460366-6. download. |
 |
The most successful exorcism of Maxwell’s demon is Smoluchowski’s
1912 observation that thermal fluctuations would likely disrupt the
operation of any molecular scale demonic machine.
Information-theoretic exorcisms fail since these same thermal
fluctuations invalidate the molecular scale manipulations upon which
the thermodynamics of computation is based. A new argument
concerning conservation of phase space volume shows that all
Maxwell’s demons must fail. |
"All Shook Up: Fluctuations, Maxwell's Demon and the
Thermodynamics of Computation." Entropy 2013, 15,
4432-4483. Download.
For a short extension of the exorcism to quantum theory, see ""The
Simplest Exorcism of Maxwell's Demon: The Quantum Version." Download. |
 |
Efforts to exorcise Maxwell's demon have focused on the
information processing a demon supposedly must do. There is a much
simpler exorcism. If the demon and thermal system combined are a
Hamiltonian system, then the intended operation of the demon must
compress the overall phase space, in violation of Liouville's
theorem. |
The
Simplest Exorcism of Maxwell's Demon
No Information Needed
In Goodies pages. Based on Section 4 of "All Shook Up..." |
 |
The idea of a thermodynamically reversible process is central to
thermodynamics. Yet essentially all descriptions of them over nearly
two centuries are internally contradictory. They consist of
equilibrium states, which are by definition unchanging in time; yet
still they still change in time. I review the history and offer a
solution. |
"The Impossible Process: Thermodynamic
Reversibility," Studies in History and Philosophy of Modern
Physics, 55(2016), pp. 43-61. Download |
 |
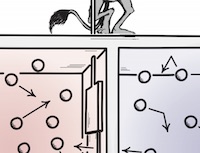
Thermodynamically reversible processes cannot be completed in
systems at molecular scales. They are fatally disrupted by
fluctuations. This paper reviews the general result and computes two
cases in detail. |
"Thermodynamically Reversible Processes in
Statistical Physics." American Journal of Physics, 85
(2017), pp. 135-145. Download. |
 |
The received view is that a Maxwell's demon must fail to reverse
the second law of thermodynamics for reasons to do with information
and computation. This received view has failed, I argue, and our
continuing preoccupation with it has distracted us from a simpler
and more secure exorcism that merely uses the Liouville theorem of
statistical physics. I extend this exorcism to the quantum case. |
"Maxwell's Demon Does not Compute." In Michael E.
Cuffaro and Samuel C. Fletcher, eds., Physical Perspectives on
Computation, Computational Perspectives on Physics.
Cambridge: Cambridge University Press. 2018. pp. 240-256. Download.
|
 |
Narrative conventions in a thought experiment allow thought
experimenters great latitude in deciding which processes are typical
and bear generalization and which can be idealized away as
incidental. This latitude is abused in the worst thought experiment
in science. |
"The Worst Thought Experiment," The Routledge
Companion to Thought Experiments. Eds. Michael T. Stuart,
James Robert Brown, and Yiftach Fehige. London: Routledge, 2018. pp.
454-68. Download. |

|
Landauer's principle mistakenly associates thermodynamic entropy
creation in a computating device with the logic of the computation
implemented. The mistake derives from a neglect of the dynamical
character of the probability W in Boltzmann's celebrated "S = k ln
W." |
"A Hot Mess," Inference: International Review
of Science. Vol. 4, Issue 3. Draft |
 |
In 1824, Sadi Carnot proposed the strange, internally
contradictory notion of a thermodynamically reversible process as
the most efficient in the context of dissipative heat engines. They
are analogous to the reversible geometrical movements that his
father, Lazare, had earlier found to be the most efficient in
ordinary, mechanically dissipative machines. |
"How Analogy Helped Create the New Science of
Thermodynamics"
Download draft. |

|
Both Wayne Myrvold and I have written pieces, relating to Landauer's principle, in which fluctuations are alluded to in their titles. We explain that the pieces are mutually compatible. |
(With Wayne Myrvold) "On Norton’s '...Shook...' and Myrvold’s 'Shakin’ ...' " Philosophy of Physics, Vol. 1, Issue 1, Article 5. Download. |

|
A purely thermodynamic argument precludes dissipationless erasure. Gibbs' "- k p log p" entropy formula, properly applied, does not assign an information entropy to pre-erasure states. Suppressing fluctuations remains the principal source of dissipation in molecular scale processes. |
"The Simply Uninformed Thermodynamics of Erasure," Philosophy of Physics3(1): 3,1-20 Download. |

|
Nearly a century of efforts have failed to find a novel connection of foundational importance between thermodynamic entropy and notions of information and computation. This paper is a short summary of how these efforts have failed. It includes an account of how the Landauer principle conflates dynamic with static probabilities.
Too good, to be true is a utopian work published by Thomas Lupton in 1580. |
"Too Good to be True: Entropy, Information, Computation and the Uninformed Thermodynamics of Erasure," Download.
|
|
|















