
| HPS 2814 | Einstein | Spring 2023 |
Back to Schedule.
Back to course Documents.
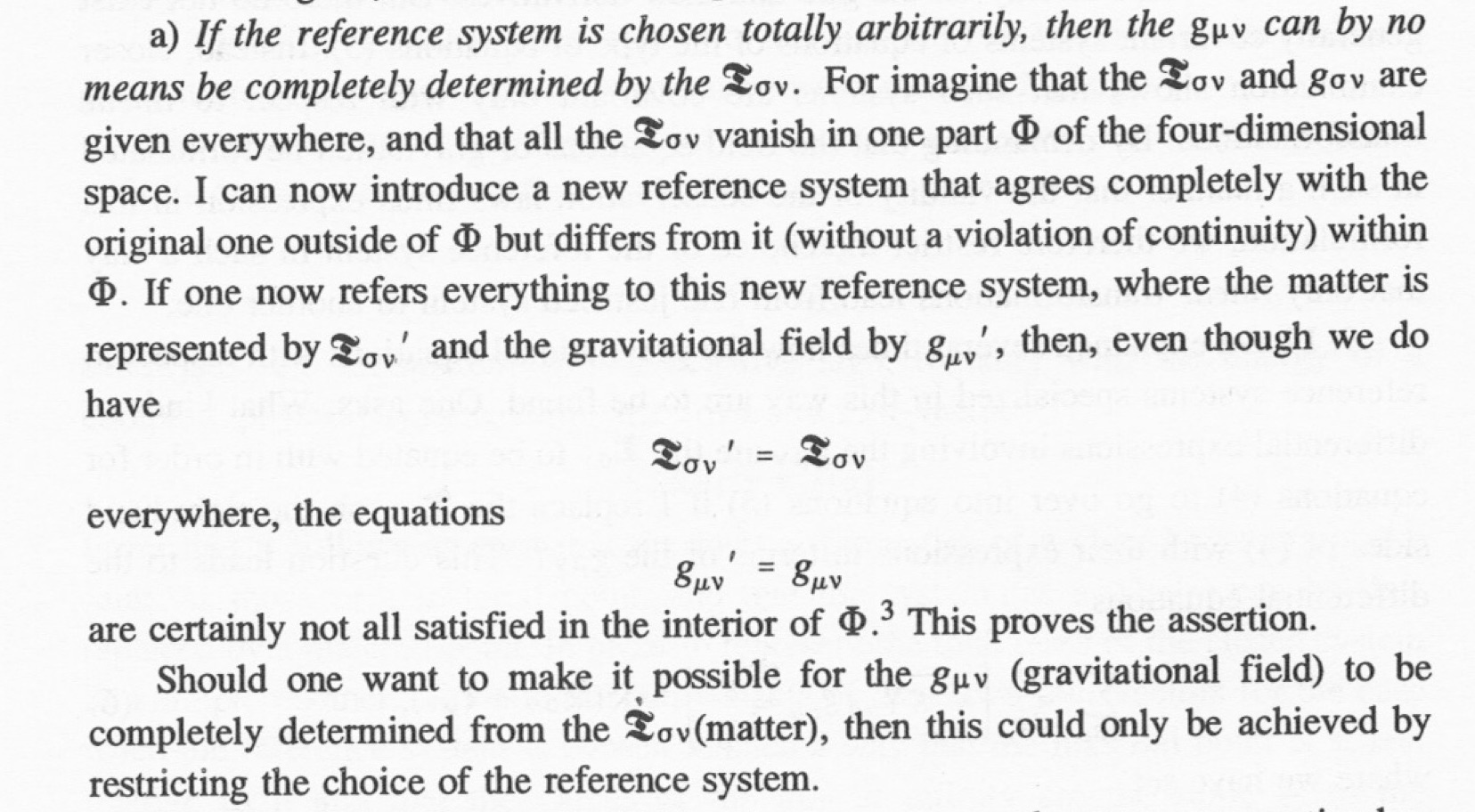
Einstein's Hole Argument
Background reading:
John D. Norton, "The
Hole Argument," Stanford Encyclopedia of Philosophy.
John Stachel, "The
Hole Argument and Some Physical and Philosophical Implications,"
Living Reviews in Relativity.
The Four Published Versions
A. Einstein, "On the Foundations of the Generalized Theory of Relativity and the Theory of Gravitation," Physikalische Zeitschrift 15(1914), pp. 176-180.


A. Einstein, "Comments" on "Outline of a Generalized Theory of Relativity and of a Theory of Gravitation," Zeitschrift fuer Mathematik und Physik, 62(1914), pp. 260-61.

A. Einstein and M. Grossmann, "Covariance Properties of the Field Equations of the Theory of Gravitation Based on the General Theory of Relativity," Zeitschrift fuer Mathematik und Physik, 62(1914), pp. 215-225.
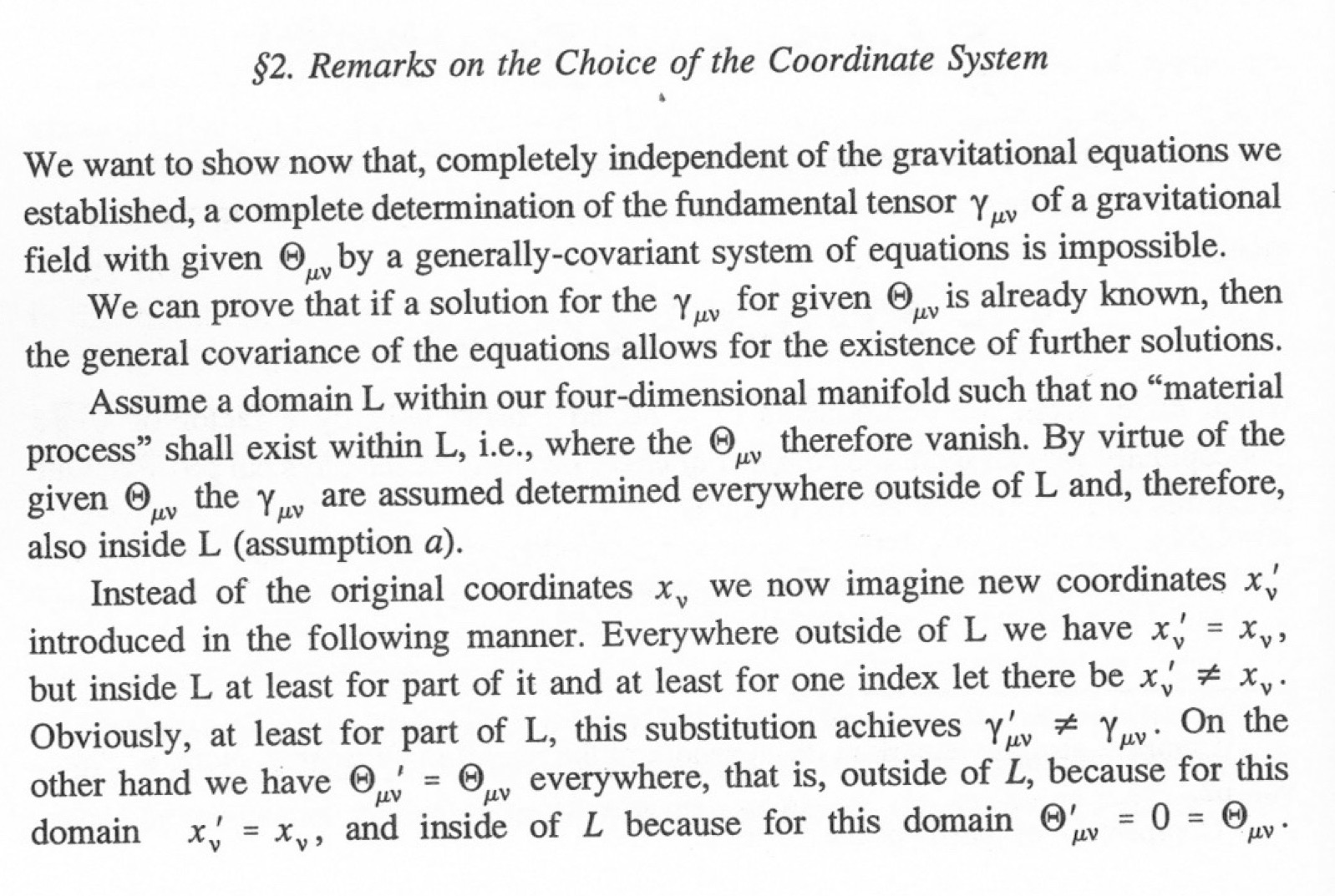

A. Einstein, "The Formal Foundation of the General Theory of Relativity," Koeniglich Preussische Akademie der Wissenschaften (Berlin). Sitzungsberichte. (1914), pp. 1030-1085.

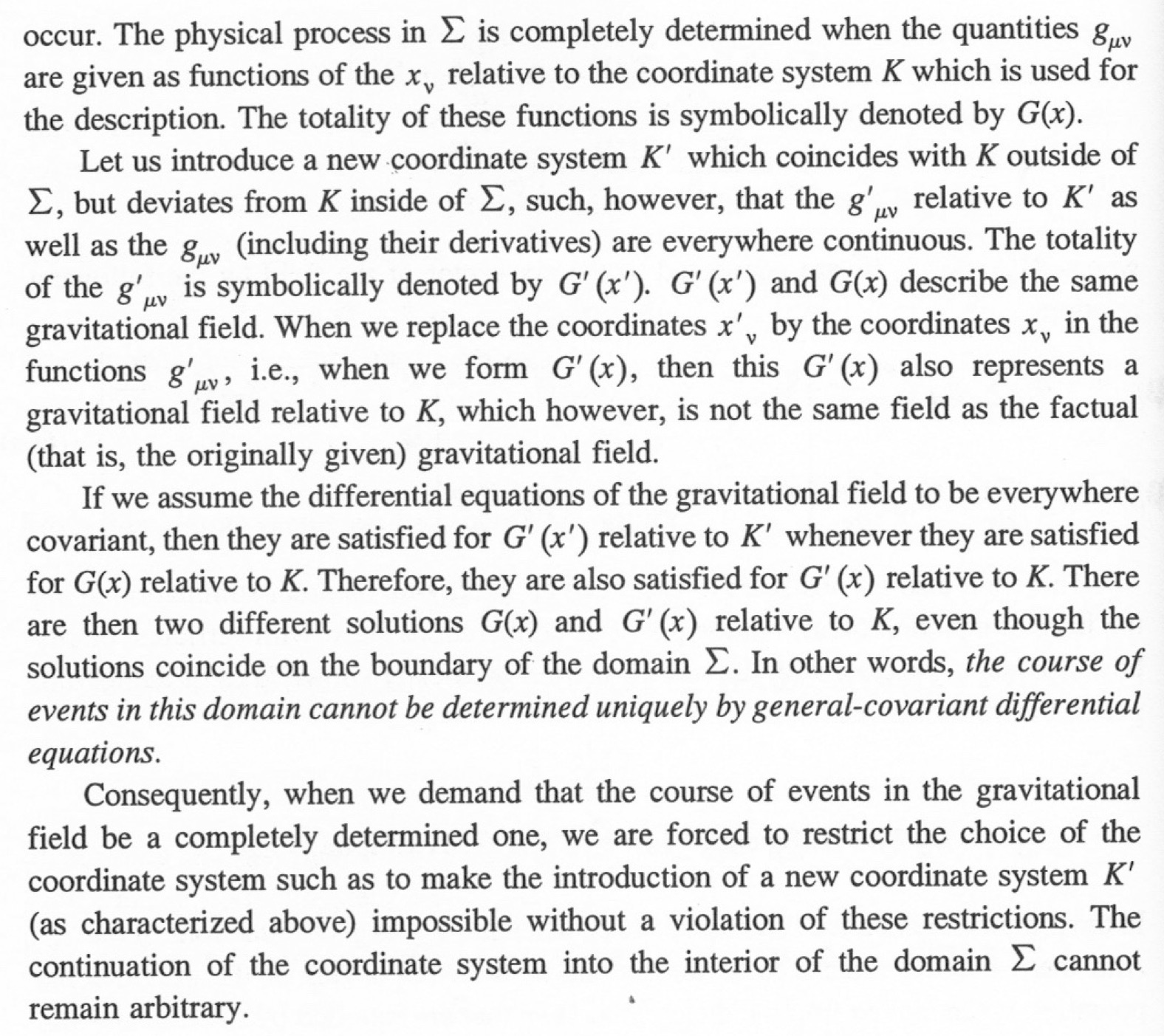
Einstein's Point-Coincidence Argument
The Published Version
A. Einstein, "The Foundation of the General Theory of Relativity," Annalen der Physik, 49 (1916).
It is clear
that a physical theory that satisfies this postulate [of general
covariance] will also be suitable for the general postulate of relativity.
For the sum of all substitutions in any case includes thoses
which correspond to all relative motions of three-dimensional systems of
co-ordinates. That this requirement of general co-variance, which takes
away from space and time the last remnant of physical objectivity, is a
natural one, will be seen from the following reflection. All our
space-time verifications invariably amount to a determination of
space-time coincidences. If, for example, events consisted merely in the
motion of material points, then ultimately nothing would be observable but
the meetings of two or more of these points. Moreover, the results of our
measurements are nothing but verifications of such meetings of the
material points of our measuring instruments with other material points,
coincidences between the hands of a clock and points on the clock-dial,
and observed point-events happening at the same place at the same time.
The introduction of a system of reference serves no other purpose than to
facilitate the description of the totality of such coincidences. We allot
to the universe four space-time variables x1, x2, x3,x4,
in such a way that for every point-event there is a corresponding system
of values of the variables x1 . . . x4. To two
coincident point-events there corresponds one system of values of the
variables x1 . . . x4, i.e., coincidence is
characterized by the identity of the co-ordinates. If, in place of the
variables x1, x2, x3,x4, we
introduce functions of them, x'1, x'2, x'3,x'4,
as a new system of co-ordinates, so that the systems of values are made to
correspond to one another without ambiguity, the equality of all four
co-ordinates in the new system will also serve as an expression for the
space-time coincidence of the two point-events. As all our physical
experience can be ultimately reduced to such coincidences, there is no
immediate reason for preferring certain systems of coordinates to others,
that is to say, we arrive at the requirement of general covariance.
In Correspondence
Einstein to Michele Besso, 3 January 1916 (translation from John Stachel, "The Hole Argument...")
Everything in the hole argument was correct up to the final conclusion.It has no physical content if, with respect to the same coordinate system K, two different solutions G (x) and ′ G (x ) exist, To imagine two solutions simultaneously on the same manifold has no meaning, and indeed the system K has no physical reality. The hole argument is replaced by the following consideration. Nothing is physically real but the totality of space-time point coincidences. If, for example, all physical events were to be built up from the motions of material points alone, then the meetings of these points, i.e., the points of intersection of their world lines, would be the only real things, i.e., observable in principle. These points of intersection naturally are preserved during alltransformations (and no new ones occur) if only certain uniqueness conditions are observed. It is therefore most natural to demand of the laws that they determine no more than the totality of space-time coincidences. From what has been said, this is already attained through the use of generally covariant equations.
Einstein to Paul Ehrenfest, 26 December 1915 (My translation).
In §12 of
my work of last year, everything is correct (in the first three
paragraphs) up to the italics at the end of the third paragraph. One can
deduce no contradiction at all with the uniqueness of occurrences from the
fact that both systems G(x) and G'(x), related to the same reference
system, satisfy the conditions of the grav. field. The apparent force of
this consideration is lost immediately if one considers that
(1) the reference system signifies nothing real
(2) that the (simultaneous) realization of two different g-systems
(betters said, two different grav. fields) in the same region of the
continuum is impossible according to the nature of the theory.
In the place of §12 steps the following consideration. The reality of the
world-occurrence (in opposition to that dependent on the choice of
reference system) subsists in spacetime coincidences. [Footnote:
and in nothing else!] For example the intersection points of two different
world lines are real as is the assertion that they do not
intersect one another. Those assertions, which refer to physical reality,
are not lost then through any (unambiguous) coordinate transformation. If
two systems of gμν (or [more] gen.[erally],
variables used for describing the world) are so constituted, that one can
obtrain the second from the first merely through a space-time
transformation, then they refer to exactly the same thing. For they have
all timespace coincidences in common, i.e. all that is observable. This
consideration shows immediately how natural is the requirement of general
covariance.
Background reading
Marco Giovanelli, “Erich Kretschmann as a proto-logical-empiricist:
Adventures and misadventures of the point-coincidence argument,” Studies
in History and Philosophy of Modern Physics, 44: 115–134. Preprint
at http://philsci-archive.pitt.edu/10158.