Assignment 6: Spacetime and Four Dimensional Spaces

For submission Tuesday, February 13, Wednesday February 14

1. In an ordinary space, a straight line is the shortest distance between two points (a "geodesic"). Imagine that there are several roads that can be driven from Pittsburgh to Philadelphia and you know that only one of the them is a perfect straight line.You drive them all. How could you use your car's odometer to determine which is the straight line path? (A car's odometer is sometimes called a "tripmeter" and measures the distance the car has traveled.)

2. In a Minkowski spacetime, an inertial trajectory connecting two events is the curve ofgreatest elapsed proper time. A wristwatch carried by a traveler measures the proper time elapsed along some timelike curve in a Minkowski spacetime. Many travelers, one of them moving inertially, set out from event A to event B in a Minkowski spacetime. How can the readings on their wristwatches be used to determine which is the inertially moving traveler?

3. How do the processes described in 2. relate to the Twin Effect ("Paradox") in Spacetime.

For discussion in the recitation

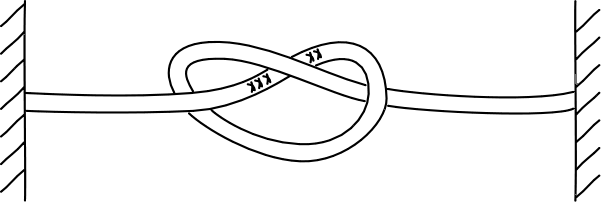
A. Consider the two challenges in "What is a four dimensional spacetime like?" The second is to show that there are no knots in a four dimensional space. Use the techniques described to show that if the knot shown were in a four dimensional space, the knot could be untied without detaching the ends of the rope from the walls.

knot

Hint: consider the section of the rope marked "XXXXX." What if it were lifted into the fourth dimension?

B. An equilateral triangle is a plane figure bounded by three lines of equal length. It is drawn by taking a line AB and a point C not on AB. The points A and B are connected to C with straight lines. C is selected so that all three lines AB, AC and BC are equal in length.

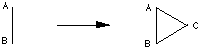
A regular tetrahedron is is a three dimensional solid bounded by four equilateral triangles. It is drawn by taking an equilateral triangle ABC and a fourth point D. The points A, B and C are connected to D by straight lines. D is selected so that each of the triangles ABC, ABD, BCD and ACD are equilateral.

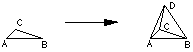
Continuing in this pattern, what does a four dimensional tetrahedron look like? How is it constructed? Draw one.

(For the brave to tackle outside the recitation: Compute the area and volume of an equilateral triangle and a regular tetrahedron. Continue to compute the four dimensional volume of the figure drawn in B. Warning: This is a hard problem. I have not found a simple way of doing it!)