| HPS 0410 | Einstein for Everyone | Spring 2007 |
Back to main course page
For submission Tuesday March 20/ Wednesday March 21.
1. In the spacetime surrounding the sun:
(a) How is curvature of a space-time sheet manifested? What sorts of experiments might we do to detect it?
(b) How is curvature of a space-space sheet manifested? What sorts of experiments might we do to detect it?
2. (a) What is the essential idea of Einstein's gravitational field equations?
(b) Why is it plausible that the Minkowski spacetime of special relativity conforms to them in case the spacetime's matter density is everywhere zero?
(c) Does this mean that a Minkowski spacetime is the only possibility where the matter density is zero? Why not?
3.The essence of Einstein's new theory of gravity is to depict gravitational effects as resulting from a curvature of spacetime. Why is the equality of inertial and gravitational mass in Newton's theory important to this depiction?
For discussion in the recitation.
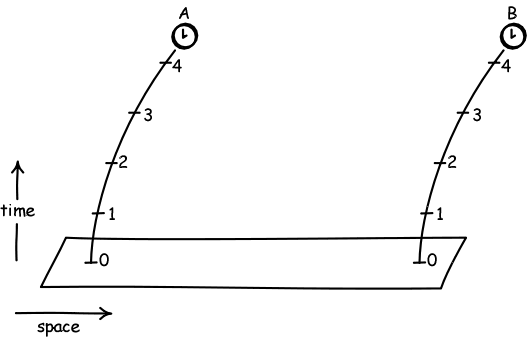
A. Einstein first hit upon the idea that gravitation slows clocks through a thought experiment conducted fully within a Minkowski spacetime of special relativity. He imagined an observer with two clocks all enclosed within a box and accelerating in a Minkowski spacetime. The clocks run at different rates, according to their position in the box. Einstein's principle of equivalence told him that the inertial field appearing in the box was nothing other than a special form of a gravitational field, which he deemed responsible for the slowing.
The relative slowing of the clocks can be recovered fully from the spacetime geometry of a Minkowski spacetime. Here is a spacetime diagram of two clocks accelerating. Draw in hypersurfaces of simultaneity for observers located with the clocks and moving with them. Show that the B-clock observer judges the A-clock to run o run slower; and the A-clock observer judges the B-clock to run faster.

B. Einstein regarded his general theory of relativity as explaining the otherwise unexplained coincidence of the equality of inertial and gravitational mass in Newtonian theory. To what extent was this like Einstein's special theory explaining why all efforts to detect the ether state of rest had failed?
C. Einstein regarded his principle of equivalence as extending the relativity of motion to uniform acceleration. For, he said, the observer in the box described in A. need not interpret the effects in the box as due to the box's motion. They could instead be interpreted as effects of a gravitational field seen by an observer at rest. How much is the principle of equivalence like the relativity of inertial motion?