Back to main course page

Assignment 8: General Relativity

For submission

1.The effect of geodesic deviation can be used to detect curvature in spacetime.

(a) The simplest case is the gravitation free Minkowski spacetime. Consider four objects arranged at equal distances apart in a straight line in Minkowski spacetime and initially at rest. Draw a spacetime diagram of their ensuing worldlines. Use the notion of geodesic deviation to conclude that the sheet of the spacetime that they are exploring is flat.

(b) Now imagine that the same four bodies are momentarily at rest, high above the surface of a planet, such as our earth, all lined up at the same altitude. They are released and begin to fall towards the planet. Draw a spacetime diagram of the ensuing worldlines. Use the notion of geodesic deviation to conclude that the sheet of spacetime they are exploring is curved.

2. (a) What is the essential idea of Einstein's gravitational field equations?

(b) Why is it plausible that the Minkowski spacetime of special relativity conforms to them in case the spacetime's matter density is everywhere zero?

(c) Does this mean that a Minkowski spacetime is the only possibility where the matter density is zero? Why not?

3.(a) What consequence does the equality of inertial and gravitational mass of Newtonian theory have for bodies in free fall?

(b) How is this consequence important to Einstein's new theory of gravity, which depicts gravitational effects as resulting from a curvature of spacetime?

For discussion in the recitation.

A. According to general relativity, there is noticeable curvature in the space-time sheets of spacetime in the vicinity of the earth. That curvature is manifested as gravitational effects. General relativity also tells us that the geometry of space above the surface of the earth has a very, very slight curvature as well. That would be manifested as a curvature in a "space-space" sheet of spacetime.

How could it be measured, assuming that precise enough measurements could be made?

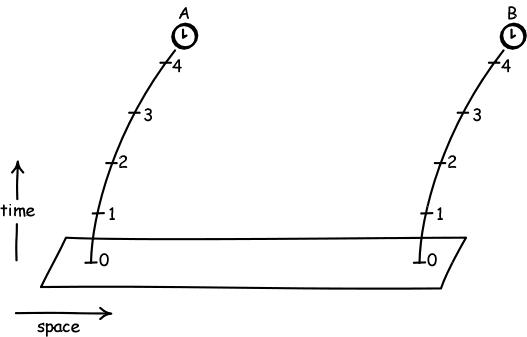
B. Einstein first hit upon the idea that gravitation slows clocks through a thought experiment conducted fully within a Minkowski spacetime of special relativity. He imagined an observer with two clocks all enclosed within a box and accelerating uniformly in a Minkowski spacetime. He then showed that, according to special relativity, the clocks run at different rates, according to their position in the box. The farther forward they are in the direction of the acceleration, the faster they run. Einstein's principle of equivalence then added the assertion that the inertial field appearing in the box was nothing other than a special form of a gravitational field. So he concluded that clocks run at different rates according to their altitude in a gravitational field. The higher clocks run faster and the lower ones slower.

The relative slowing of the clocks can be recovered fully from the spacetime geometry of a Minkowski spacetime. Here is a spacetime diagram of two clocks accelerating. The acceleration is in the direction from the A clock to the B clock. Draw in hypersurfaces of simultaneity for observers located with the clocks and moving with them. Show that the B-clock observer judges the A-clock to run slower; and the A-clock observer judges the B-clock to run faster.

C. Einstein took a radically new approach to gravity by declaring it to coincide with a curvature of spacetime. However, as we have seen in the chapter, the same thing can be done with Newtonian gravitation theory, so that all its gravitational effects can be associated with a curvature in some parts of spacetime. So what is new with Einstein's proposal?

D. You can take a flat sheet of paper and wrap it into a cylinder, so that its rightmost edge coincides with its leftmost edge. That operation does not affect the intrinsic flatness of the paper. One can do the same thing in imagination with a cubical chunk of Minkowski spacetime to create a very odd, new spacetime. Take the chunk's rightmost edge and declare that it coincides with its leftmost edge. That means that anyone traveling past the surface marking rightmost edge of this space would simply pop back at the surface marking the leftmost edge. Use geodesic deviation to convince yourself that the wrapping up of this spacetime has not changed the flatness of the spacetime.

E. What are the three classic tests of general relativity? How good are they? Are there other ways to explain the observations upon which they depend? If so, why should we prefer the explanation provided by general relativity?