
| HPS 0410 | Einstein for Everyone |
Back to main course page
John
D. Norton
Department of History and Philosophy of Science
University of Pittsburgh
After this chapter was
written, I undertook a more detailed study of Einstein's treatment of
spacetime singularities. The result is
"Einstein against Singularities: Analysis versus Geometry," Philosophy
of Physics 2(1): 13, 1–73. Download from journal
or here.
The existence of black holes now seems so familiar that it is hard to imagine that even recently the mere possibility of black holes was seriously doubted. While it seems reasonable to hope that a serviceable theory of quantum gravity might no longer harbor spacetime singularities, present work in general relativity treats spacetime singularities as routine features arising in common spacetimes. The earlier view was that they are pathologies of the theory that could not be realized in nature. We saw a glimpse of this in Eddington's remark that a big bang origin of the universe is "repugnant to me."
In a similar vein, Einstein regarded black holes as lying outside proper physics. His antipathy to them was quite strong. In the modern literature, the singularity at the center of a black hole is the locus of great concern. Einstein's analysis did not extend that far. He already found the event horizon at the Schwarzschild radius to be troublesome. Where we now find the spacetime to be quite regular at the event horizon, he identified it as singular, on the basis of pathologies in the particular coordinate description he used for the spacetime. He even argued that physical processes would prevent gravitational collapse proceeding far enough to form a black hole.
After this chapter was written, I undertook a more extensive examination of Einstein's treatment of spacetime singularities. It includes his treatment of black holes and also a singularity he identified in a de Sitter spacetime. See "Einstein against Singularities: Analysis versus Geometry."
| In 1939, Einstein published a paper in which he argued that physical processes would prevent the formation of black holes. His argument was straightforward and not too hard to follow. What is hard to understand is why its conclusion could be seen to preclude the formation of black holes in all generality. | Albert Einstein, "On a Stationary System With Spherical Symmetry Consisting of Many Gravitating Masses," The Annals of Mathematics, 40, (Oct., 1939), pp. 922-936. |

Einstein's argument started by considering a mass in a circular orbit in a Schwarzschild spacetime in a region outside the event horizon. In order to maintain its circular orbit, the mass needs to move very fast. It must do this for the same reason that our planet earth must move quickly in its orbit so that it does not fall into the sun. What Einstein found is that as these orbits get closer to the event horizon, the speed necessary for stability increases until it approaches a limiting orbit that is at a radial coordinate distance r of r = (2+√3) ≈ 3.73 times the coordinate distance rs of the event horizon from the center of the spacetime. The closer the mass' orbit is to this limiting orbit, the closer its speed is to the speed of light. Since, according to relativity theory, ordinary masses cannot move at the speed of light, this limiting orbit is the unrealized limit for the orbital motion of all these masses.
Einstein then considered a swarm of masses all moving in circular orbits above what would be the event horizon. He assumed them to be moving in many directions such that they form a spherical cloud or perhaps a collection of spherical shells around the same central point. Since these masses have a spherically symmetric distribution, they can be source masses for a Schwarzschild spacetime. These masses, if they were to proceed to collapse onto each other, would produce a spacetime with an event horizon that would be inside the innermost shell of the masses, prior to their collapse.
Presumably Einstein imagined cosmic matter collecting under its own gravity and forming the huge swirling configurations that we see in galaxies. As a computational convenience, he added the assumption that their distribution is spherical since then he can conclude that the spacetime structure is that of a Schwarzschild spacetime.
 |
Einstein now argued that his earlier result precludes the masses collapsing and forming an event horizon. He summarized his conclusion as: | The "Schwarzschild singularity" Einstein named here is not the singularity at the center of a black hole. Rather it refers to the event horizon. In the coordinate systems Einstein used to describe the Schwarzschild spacetime, a quantity becomes singular at the event horizon. We now recognize that these singularities are merely artifacts of the particular coordinate systems used by Einstein and not physical pathologies. It remains an open question in history of science why Einstein regarded these coordinate singularities as serious physical pathologies. See below for further discussion. |
"The essential result of this investigation is a clear understanding as to why the "Schwarzschild singularities" do not exist in physical reality. Although the theory given here treats only clusters whose particles move along circular paths it does not seem to be subject to reasonable doubt that more general cases will have analogous results. The "Schwarzschild singularity" does not appear for the reason that matter cannot be concentrated arbitrarily. And this is due to the fact that otherwise the constituting particles would reach the velocity of light."
Einstein's optimism that this mechanism would reappear in any other process is clearly mistaken. We have empirical evidence of that bodies can fall past the event horizons of black holes and merge with the black holes. In January 2020, the LIGO observatory reported finding two cases, ten days apart, of neutron star-black hole mergers. (GW200105, GW200115)

From
the LIGO website, https://www.ligo.caltech.edu/image/ligo20210629d
LIGO image caption: "...image from a simulation consistent with GW200115
GW signal and black hole - neutron star coalescence."
While Einstein's conclusion was clearly hasty, we should not judge him too severely. General relativity does allow these processes of neutron-black hole mergers. However the computations that trace out the details are very difficult and not possible with the simple pen and paper resources used by Einstein in 1939. Present day analyses of these mergers are carried out in massive, computer driven, numerical simulations, such as here.
| There is a deeper reason for Einstein's reluctance to accept the physical possibilities of black holes. He harbored a strong aversion to singularities in his theories. Four years before the paper above, Einstein published a paper with a collaborator, Nathan Rosen. It touched on singularities in general relativity. There they expressed clearly the view that singularities are untenable in a physical theory (p. 73) | Albert Einstein and Nathan Rosen, "The Particle Problem in the General Theory of Relativity," Physical Review, 48 (1935), pp. 73-77. |
"For a singularity brings so much arbitrariness into the theory that it actually nullifies its laws. ... Every field theory, in our opinion, must therefore adhere to the fundamental principle that singularities of the field are to be excluded."
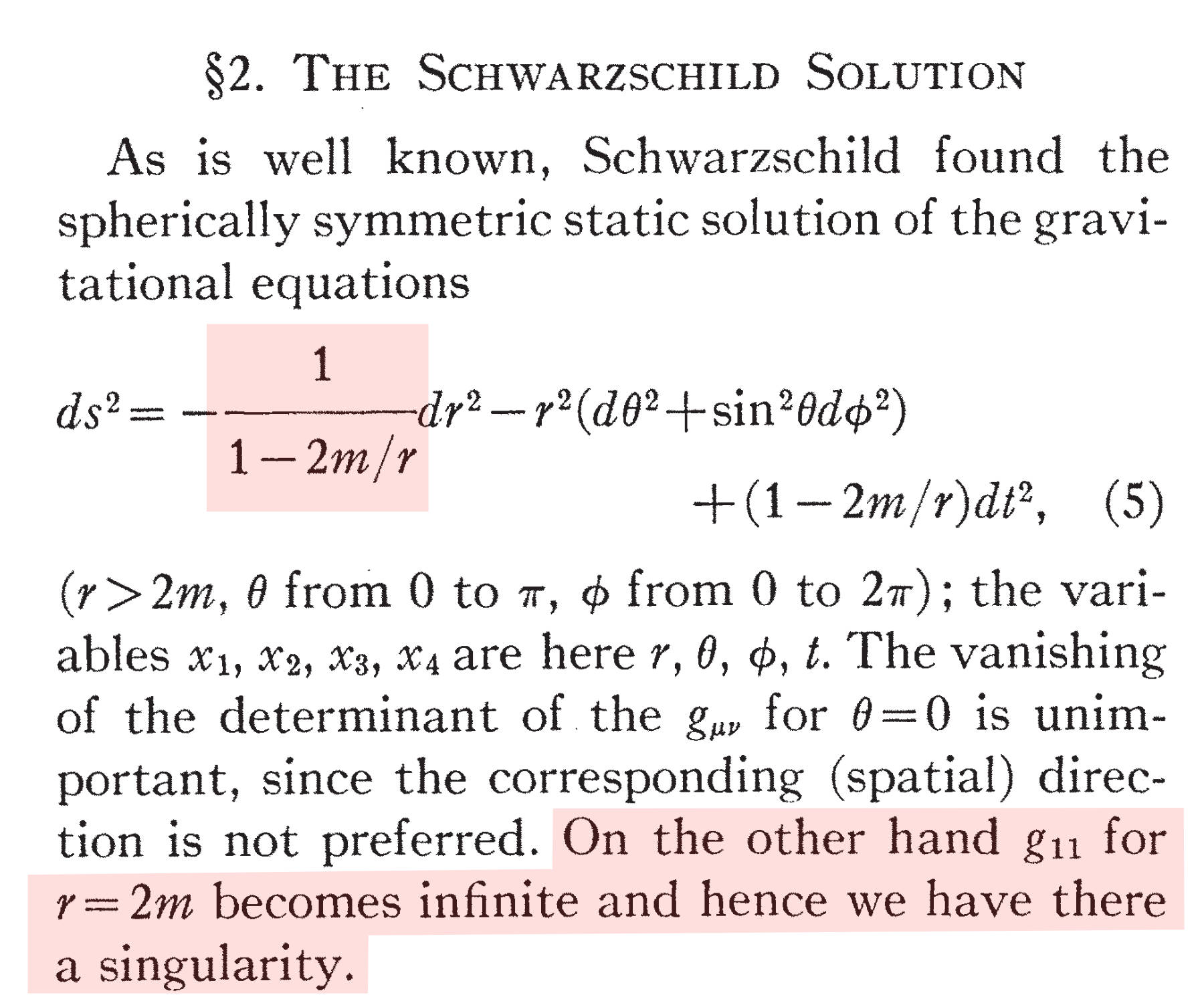
There was a further complication. It is now part of any introduction to black holes that there is nothing pathological in the local spacetime geometry at the event horizon. The point most commonly made is that the spacetime curvature at the event horizon remains finite. It may even be quite small if the event horizon is large, because the mass parameter is large. That, however, was not Einstein's view. He located a singularity at the event horizon, or, as it is sometimes called, the "Schwarzschild radius." In his 1939 paper, Einstein called it the "Schwarzschild singularity." This was the singularity that Einstein and Rosen deplored.
We may now be able to understand why Einstein would deplore ineliminable singularities in spacetime theories, such as the singularity at the center of a black hole. What is harder to understand is why Einstein and Rosen characterized the event horizon as a singularity that should be excluded from physical theories. The reason seems to lie in the particular methods Einstein employed in his theorizing
In this text, the discussion was carried out in what is now called a "geometrical" mode. Structures in spacetime are identified by their geometry: lines, planes, spheres and so on. For example, a circle is presented directly as a figure, much as Euclid would have done.
Einstein however proceeded in an "algebraic" mode. That means that he would describe the figure in terms of variables, like x and y. Einstein's unit circle would have been the formula:
x2 + y2 = 1
Here Einstein is following Descartes' analytic method. His variables x and y are Cartesian coordinates.
It is possible to use many different coordinate systems. We do not have to use the Cartesian coordinates above. A popular choice for a circle are radial coordinates. The radial coordinate r gives the distance of a point from some arbitrarily chosen origin; and the angular coordinate θ determines its direction:
The formula for a unit circle is now:
r = 1
In the end, it does not matter if we treat the circle
geometrically, as did Euclid, or algebraically with either of the formulae
above. The results will be the same.
There is trap for the unwary in using such algebraic formula. Points everywhere in the plane can be identified by giving the unique pair of coordinates r, θ for the point. That is true for all points, except the origin, where r = 0. What value of θ should we assign to it? Since θ is meaningful only in giving the direction in space of a point from the origin, it cannot be applied to the origin. We might assign all values of θ to the origin, or none at all.
As long as we realize that this is the origin of coordinates, we are unlikely to be confused by this problem. If however we just treat r and θ as variables, then we might be very worried that in some circumstances we are unable to assign a value to θ. The remedy is to recognize that there is no problem with the space. This indefiniteness is just an oddity of the coordinate system and not the geometry of the space. A common diagnosis is that the coordinate system "goes bad" at the origin.
Einstein and Rosen's condemnation of the event horizon as singular seems to be tied up with their use of a coordinate system that "goes bad" at the event horizon. They attribute a problem to the spacetime whereas it is merely an eliminable artefact of the particular coordinate system they used. It seems hard to believe now that this was behind Einstein and Rosen's thinking. Perhaps further historical studies will provide a different explanation. For now, however, it seems to be the only possibility.
A spacetime requires four coordinates to identify events. Commonly we have one time coordinate and three space coordinates, such as t, x, y and z. For spacetimes like a Schwarzschild spacetime, it is more convenient to label the events with so called "spherical coordinates." They are a time coordinate t, a radial coordinate r, whose values propagate out equally in all directions from the central spatial point, and two angle coordinates, θ and φ. Using these coordinates, here is how Einstein and Rosen represented the Schwarzschild spacetime in their 1935 paper (for a black hole of mass m in suitable units of mass and setting c=1):
Here is how these coordinates are assigned to events around the event horizon. (The figure does not show the θ coordinate.)
The time coordinate t runs up and down in the figure. The radial coordinate r starts at the event horizon or Schwarzschild radius of r = 2m and proceeds outward. The φ coordinate gives the angular position around the event horizon. These coordinates are not shown within the event horizon since they do not develop continuously past the event horizon.
So far, we cannot see how this coordinate system "goes bad." To that end, we will first suppress further the φ coordinate and just show a two dimensional sheet of spacetime for some fixed φ coordinate value, such as φ = 0. It looks like this:
This appears benign, but only because the figure does not show all the pertinent structure of the Schwarzschild spacetime. It is tempting to imagine that differences in the time coordinate correspond to differences in proper time such as may be read by a clock. That is not the case. Equal differences in the t coordinate in two parts of the spacetime will in general not correspond to two equal increments of proper time.
Consider, for example the equal increments of the time coordinate "dt" shown by the blue marks in the figure:
To find the proper time associated with them, a correction factor must be applied. It is given in Einstein and Rosen's expression for ds2 above. If there is a coordinate difference of dt, then the proper time elapsed is given by:
√(1 - 2m/r) dt
The problem is that the factor √(1 - 2m/r) gets arbitrarily close to zero as the blue marks in the figure approach the Schwarzschild radius at r = 2m; and it is zero at the Schwarzschild radius.
√(1 - 2m/r) → √(1 - 1) = 0
That means that the blue mark at the event horizon corresponds to no difference in time at all. That is, at the event horizon, the t coordinate assigns all values of t to the same event. The coordinate system has "gone bad" in the same way that the radial coordinate system described above went bad at the origin.
There is another problem for small differences in the radial coordinate "dr." The same difference in the radial coordinate r will correspond to different proper distances in space, such as would be measured by a physical ruler. Once again, Einstein and Rosen's formula above supplies the correction factor. The coordinate difference dr must be multiplied by the factor 1 / √(1 - 2m/r) to recover the proper distance. That is, the distance is
1 / √(1 - 2m/r) dr
This factor is the (square root of the) "g11" factor that so concerned Einstein and Rosen in the passage quoted above. Their worry was that this factor 1 / √(1 - 2m/r) takes on an infinite value at the Schwarzschild radius r = 2m:
1 / √(1 - 2m/r) = 1 / √(1 - 1) = 1 / √0 = 1 / 0 = ∞
They were right that this factor takes an infinite value. However that by itself does not establish that there is a pathology in the spacetime itself. As this factor grows larger, the coordinate differential dr, represented by the blue patches in the figure above, can be made smaller at a faster rate, so that the spatial lengths assigned to the blue marks remain finite as the event horizon at r = 2m is approached.
Here is the explicit calculation of this convergence.
In sum, Einstein and Rosen are right to identify a problem in their formula. However they are wrong to identify it as a singularity in the spacetime structure itself. Rather, the odd effects just recounted derive from the unfortunate choice of spacetime coordinates that they have made. As we shall see, with another, more careful choice of spacetime coordinates, these anomalies disappear.
In the case of the circle above, we could describe the same circle in different coordinate systems without changing any of its geometric properties. The same can be done for a Schwarzschild spacetime. We can transform to a new coordinate system in which the pathologies bothering Einstein and Rosen do not appear.
The mathematical details are given here for the curious.
The transformation involves only Einstein and Rosen's t and r coordinates. They are replaced by the new coordinates T and X. The angle coordinates θ and φ are unaffected. When the new coordinate system is explored, we find that Einstein and Rosen's original coordinate system, for the region outside the event horizon, corresponds only to the wedge shown in the figure below:
The upward pointing diagonal corresponds to the event horizon and, crucially, the coordinate system passes through it without any pathologies such as were found in Einstein and Rosen's system. This new coordinate system shows that the region outside the event horizon can be extended through the event horizon to its interior without problems.
A convenient feature of this new coordinate system is that lightlike trajectories once again always propagate along lines at 45 degrees to the perpendicular. This once again makes it easier for us to see which events can affect and be affected by others. These lines are shown here:
We saw above that Einstein and Rosen's t coordinate assigned different values to the same event at the event horizon, r = 2m. If we plot the surfaces of constant t for Einstein and Rosen's coordinate system within the new coordinate system, we do indeed find that they converge to one event at the event horizon.
The figure shows us that this t coordinate does behave like the angle coordinate in our earlier representation of a circle.
Just as the angle coordinate theta "goes bad" at the origin, so also does the t coordinate "go bad" at the event horizon.
Einstein and Rosen's "r" coordinate assigns the same value to events that are the same spatial distance from the event horizon. In the new coordinate system, curves of constant r are now represented by hyperbolas:
As the value of the r coordinate approaches that of the event horizon at r = 2m, the hyperbolas approach arbitrarily closely to the wedge that includes the event horizon. At r = 2m, they coincide with the wedge.
Copyright John D. Norton. October 29, 2020. February 5, March 25, April 11, 2022. March 26, 2024. August 7, 2024.