
| HPS 0410 | Einstein for Everyone |
John
D. Norton
Department of History and Philosophy of Science
University of Pittsburgh
For related material, see
my
"Little boxes: A
simple implementation of the Greenberger, Horne, and Zeilinger result
for spatial degrees of freedom," American Journal of Physics,
79(2)(2011), pp. 182-188.
The Einstein of this chapter is a little removed from the Einstein of popular imagination. That Einstein is the first of the modern physicists of the 20th century. He is the the genius of 1905 who established the reality of atoms, laid out special relativity and E=mc2, and made the audacious proposal of the light quantum. This same Einstein went on to conceive a theory of gravity unlike anything seen before and to reawaken the science of cosmology.

In his later years, a different Einstein emerged. The mainstream of physics followed the course of the quantum theory of the mid 1920's. Einstein recognized that this new quantum theory enjoyed remarkable empirical successes, so that it clearly had something very right. However he did not believe that future fundamental physics should be to build upon it. Rather he thought the way ahead was to develop the geometrical approach of general relativity into an all encompassing "unified field theory" within which the results of the new quantum theory would be derived. While he had contributed to its development, Einstein became the most prominent critic of the new quantum theory.

That Einstein was uncomfortable with quantum theory attracted much attention and there have been many accounts of his reservations, some trying to locate their deeper sources. However these different accounts may vary, there is no doubt of Einstein's principal objection. He believed that the quantum wave function of some system, the ψ-function, was not a complete description of the system. Rather, it provided some sort of statistical summary of the properties of many like systems. (The term "ψ-function" is just an old fashioned term for the quantum wave. ψ is the Greek letter "psi.")
An example--NOT Einstein's--will make this a little clearer. Consider the air in the room. As far as ordinary measurements are concerned, the air forms a continuous fluid. When sound propagates in air, waves of compression and rarefaction move through the air. We can arrive at a powerful theory of air and sound solely using the representation of air as a continuous fluid that harbors pressure waves.

We now know that this theory is incomplete. Air is made up of very many, very tiny molecules. The familiar pressure waves that we use to represent sound waves really represent the average positions of the molecules that comprise the air. If we could zoom in on just a small part of the sound wave, we would see something like this (where the figure is exaggerating the granularity of air):
The perfectly regular, nicely rounded pressure waves can be so uniform only because they smooth away all the bumps of the individual atoms. They do however provide a serviceable theory of air and sound waves for many many practical purposes. But they are ultimately an incomplete picture of any particular sound wave. Many different distributions of molecules can be smoothed to give the same wave. So if we are given one wave, we cannot know which particular distribution of air molecules lies behind it. It could be one of very many.
or
or
or ...
Eventually the differences between them will matter. In this example, if we use only the pressure wave picture, it will never be possible to trace out the trajectory of a single molecule, even though the complete mechanical description of the system assigns a definite trajectory to each of the very many molecules.
Einstein's attitude to the quantum wave was analogous. The ψ-function is not a complete description of any particular system. It is a description of the average of many similar systems. For many purposes, this will suffice, but ultimately it will fail. He wrote:
 Albert Einstein, "Remarks Concerning the Essays Brought Together in this Co-operative Volume," (1949) in, P. A. Schilpp, ed., Albert Einstein-Philosopher Scientist. 2nd ed. New York: Tudor Publishing, 1951, pp. 671-72. |
"Within the
framework of statistical quantum theory there is no such thing as a
complete description of the individual system. More cautiously it
might be put as follows: The attempt to conceive the
quantum-theoretical description as the complete description of the
individual systems leads to unnatural theoretical interpretations,
which become immediately unnecessary if one accepts the
interpretation that the description refers to ensembles of systems
and not to individual systems... Assuming the success of efforts to accomplish a complete physical description, the statistical quantum theory would, within the framework of future physics, take an approximately analogous position to the statistical mechanics within the framework of classical mechanics. I am rather firmly convinced that the development of theoretical physics will be of this type; but the path will be lengthy and difficult. " |
If the quantum wave is not a complete description of the physical system, then Einstein has a ready explanation of the probabilities that have now entered into physics in quantum measurement processes: they are merely expressions of our ignorance. If an atom has a probability of one half of radioactive decay over an hour, then all that really means is that its wave function describes an ensemble of many different atomic systems, half of which decay in an hour. Whether one particular atom in the ensemble will decay in one hour is definitely determinable.
However we will not be able to discern which atoms will decay if all we know is the quantum wave associated with it. Whether it decays or not depends upon properties of that system that have been smoothed away by the quantum wave and thus are unknown to us. It is our ignorance of these smoothed away properties that makes a probabilistic assertion the best we can do.

The alternative to this view of incompleteness was to accept that the quantum wave is a complete description of the system. Then the probabilities of different measurement outcomes reflect an ineliminable underdetermination in the world. Figuratively speaking, the decision as to which outcome is realized lies outside the physical system. The physics tells us that any of several outcomes is possible. Einstein referred to this situation in his oft repeated quip that he could not believe that God plays dice. The remark seems to have been made frequently, but mostly in conversation. Here is how he put it, when it was written:
| To Max Born, December 4, 1926. In Born, Born
Einstein Letters, 91. Quoted from Alice Calaprice, ed., The Expanded Quotable Einstein. Princeton University Press, 2000. p.245. |
"Quantum
mechanics is very worthy of regard. But an inner voice tells me that
this not yet the right track. The theory yields much, but it hardly
brings us closer to the Old One's secrets. I, in any case, am
convinced that He does not play dice. " |
| To Cornelius Lanczos, March 21, 1942, Einstein
Archive, 15-294. As translated in Albert Einstein: The Human Side. H. Dukas and B. Hoffmann, eds., Princeton: Princeeton Univeristy Press, 1979, p. 68. |
" " |
A note of caution is needed. The analogy to pressure waves in air is my analogy. It suggests that Einstein somehow imagined a real, point-like particle hiding behind the quantum wave, a picture not so removed from the Bohm hidden variable theory. Perhaps Einstein did entertain a picture like this in his earlier speculations. However what is quite distinctive about his mature statements of the incompleteness of quantum theory is that they are extremely cautious in describing the reality that may be hidden the statistical wave. Einstein remains as uncommitted on the question as he can possibly be.
We do know, however, where Einstein hoped to find the
theory that would ultimately complete and even replace quantum theory.
After he completed his general theory of relativity in the 1910s, Einstein
embarked on the program of extending it to cover electromagnetism. The
general theory of relativity had shown that gravity could be incorporated
into the geometry of spacetime if we allowed for a curved geometry. The
hope was that further generalizations of the geometry of spacetime would
allow a geometrical treatment of electricity and magnetism. This was the
goal of his famed "unified field theory." In
the process, Einstein hoped, a fuller account of quantum processes might
emerge.

We can have some idea of what his unified field theorizing looked like in 1935 (the year of the "EPR" paper to be discussed below). As we saw in an earlier chapter, then he posited with his EPR co-author Nathan Rosen that massive, uncharged particles might be accounted for in a purely spacetime geometric manner as Einstein-Rosen bridges.
The idea was that we normally think of a collection of uncharged, massive particles as lumps of stuff in space:
These particles were really not lumps of stuff that are distinct from the spacetime geometry itself. These particles were, in the Einstein-Rosen picture, really bridge structures connecting two spacetimes:
The proposal was quite tentative and incomplete. It did not recover any of the distinctively quantum properties of particles. That recovery, Einstein hoped, would eventually come with further exploration. This was not an enduring theory for Einstein, but a passing suggestion that seems to have faded.
Einstein pursued this project for decades, up to his death. However, the final results were inconclusive. As he dug himself deeper into these investigations, the mainstream of physics turned in other directions. While Einstein was struggling to understand how to unify two forces, gravity and electromagnetism and somehow pick up quantum properties along the way, physics had discovered two more fundamental forces, the weak and strong nuclear forces. And while Einstein focussed on the geometrical approach that proved so fruitful in the 1910s, quantum physicists were dealing with a new theory in which the idea of an observer independent reality was becoming elusive.

Einstein was relentlessly consistent in his principal complaint concerning quantum theory: it could not be a complete theory. And he was correspondingly single-minded in the principal argument he used in his efforts to establish this incompleteness. The argument depended essentially on a highly non-classical element of quantum theory that Schroedinger in the 1930s called "entanglement." (He called it "Verschränkung", in the same paper in which he presented his cat paradox.)
We can create two particles in an entangled state when some local event ejects particles in opposite directions in space, such that the properties of the two particles are correlated. If their total momentum sums to zero, for example, then, if one has a positive momentum, the other must have a momentum of the same magnitude but aligned in the opposite direction. When two particles become entangled, a complete account of the properties of one of them is not possible if it does not include the other system; and this will be true no matter how far apart the two systems may be spatially.
Entanglement can be illustrated if we consider the property of position in space of a quantum particle. If there is just one particle, we have already seen how the position property is discerned. The particle will in general be represented by a wave spread in space. We measure the position of the particle and this triggers a collapse to just one point in space.
In this simplest case, the particle wave is spread over a
small interval of space. Slightly more complicated situations are
possible. The particle may be spread into discontinuous regions of space.
For example, the wave may have two lobes and
we might measure just whether the particle is in the left or right lobe.
The measurement operation has the effect of collapsing the wave to one of
its two lobes with a probability determined by the magnitude of the two
lobes. (In the figure, the two lobes are of equal magnitude, so collapse
to each is equally probable.)
How do we get two lobes like this? It is the situation that would arise if
we confined the particle to a box that had two
disconnected chambers. The particle wave is non-vanishing only
inside the two chambers but is zero everywhere else. We do not perform a
measurement that discerns the exact position of the particle. Rather, we
merely measure whether the particle is in the left or right chamber. The
measurement will collapse the wave to one or other of its two lobes.
Now take the case of two boxes, A
and B, each with its own particle. As before the particles are
spread over the two chambers. Drawing their wave functions is a little
more complicated and this complication will be origin of entanglement. A
picture of the A particle wave in its A space and a picture of the B
particle wave in its B space by themselves omits essential information
about how the two particles are correlated.
In fullest quantum account, we do not have two waves, one for each
particle. There is only one wave corresponding to the two particles and
that one wave resides not in ordinary three dimensional space, but in the
six dimensional configuration space of the two particles. This
six dimensional space has three axes for the possible positions of the A
particle; and another three axes for the possible spatial positions of the
B particle. Picking one point in the space specifies a position for both
the A and the B particle.
The resulting six dimensional space is impossible to draw easily. However we get the essential idea if we idealize each particle as living in a one dimensional space: a one dimensional A space and a one dimensional B space. The corresponding configuration space is a two dimensional space. One of its dimensions is A space; and the other is B space. Each point in this two dimensional space gives us one spatial coordinate for the A particle and one spatial coordinate for the B particle. The wave that represents both particles is a wave in the two dimensional configuration space.
Here is one way that the two particle system wave can be distributed in this AB space:
The wave is zero everywhere except for two lobes. There is
a lobe in the region that corresponds to the left chamber "L" of box A and
the left chapter "L" of box B; and there is a second lobe in the region of
the the right chamber "R" of box A and the right chamber "R" of box B. If
we measure the position of the A particle,
the wave will collapse to one or other lobe. For concreteness, let us say
it is the first lobe; the A particle will now definitely be in the left
chamber. That same collapse will automatically induce collapse of the B
particle to its left lobe and thereby confer on the B particle the
property of definitely being in the left chamber.
That is the remarkable outcome. As a result of a
measurement on the A particle, the B particle has acquired a more definite
position, even though the two particles may be widely
separated in space.
The A particle may be in a box on my table. The B particle may be in a box
on a space station on Mars. This is entanglement. The properties of the B
particle are simply not separate from those of the A particle no matter
how far apart they may be in space. We measured the A particle that
resides on earth; and the B particle on Mars was affected. If you are
learning of entanglement for the first time and you are not amazed
by this result, you should go back and re-read the last few paragraphs.
The case just analyzed is the case of the left-right positions of the two particles perfectly correlated: that is, a "left" for the A particle always goes with a "left" for the B particle; and a "right" for the A particle always goes with a "right" for the B particles.
Other cases are possible. Here is the wave for the case of
two perfectly anti-correlated particles. You
should reflect on the figure until you are convinced that on measurement a
"left" on particle A always goes with a "right" on particle B; and
conversely.
| The difference
between correlated and anti-correlated particles cannot be
represented in the A or B spaces of the two particles individually.
They can only be represented in their joint configuration spaces.
That is why the use of configuration space is so essential. It lets
us represent otherwise elusive physical properties. It is easy to underestimate the importance of the transition from ordinary space to a configuration space in quantum theory. It is not just a redescription of things already apparent in ordinary space descriptions. It is a new element in the theory that enables the distinctively quantum effect of entanglement to be expressed. |
Those of you who have seen entanglement discussed
elsewhere will probably have seen it expressed differently, as an impossibility
of factoring the common wave function into the product of a
separate A and a separate B wave. This is the same idea as expressed
here in the figures. If we just take one lobe of the common wave
function--the left lobe, say, it can be formed by multiplying
together the left lobes of the individual wave functions. Undoing
the multiplication is just factoring the one lobe into the two
separate waves. When we have the fully entangled state with both left and right lobes present, we can no longer represent the combined wave as a simple product of two waves, one from each of the A and B spaces. |
| The earliest fully developed and published version of Einstein's argument against the completeness of quantum mechanics appeared in a 1935 article co-authored with Boris Podolsky and Nathan Rosen and universally known by the initials of its authors, "EPR." | A. Einstein, B. Podolsky, and N. Rosen, "Can Quantum-Mechanical Description of Physical Reality Be Considered Complete?" Physical Review, 47 (1935), pp. 777–780. |

The argument of the paper depends essentially on exploiting entanglement. If one has two entangled systems, one can perform measurements on one of the systems and thereby learn the properties of the other. We have seen how this would work in my example above of a particle distributed over two chambers. The key idea is that the measurement we perform on the first system will not disturb the second, so that whatever property we learn of the second system must be one it possessed prior to our making the measurement.
This is why entanglement is such a powerful idea. We can allow that a measurement on the first particle will disturb the first particle. However EPR insist that a measurement on the first particle will not disturb the second particle, which could be removed many light years from the first in space.
The argument is then completed by noting that we could have measured many different properties of the first system and, as a result, discovered many properties of the second--many more than an assumption of completeness would allow.
This can be seen in the illustration
EPR give of their general argument. We imagine two particles that
are entangled in such a way that their momenta and position coordinates
are equal but opposite in sign. The simplest way to create such an
entangled pair is through an atomic event that ejects two particles of the
same type in opposite directions.
Assume the event is such that the total momentum of the pair is zero.
Then, if the first particle has momentum +10 units, the other must have
momentum -10 units; and so on for all other possible values. Similarly,
the symmetry of the pair assures us that if the first particle has moved
to a position +100 units of distance from the creation event, then the
other must be at -100 units of distance; and so on for all other possible
positions.
It follows that we can discover the properties of the second particle at will and without disturbing it, merely by performing measurements on the first particle. We could, for example, discern the second particle's momentum by measuring the momentum of the first particle.
Or we could find the position at some moment of the second particle by measuring the position of the first.
We do not actually need to perform any of the measurements to be assured that the second particle possesses the properties mentioned. The mere possibility of the measurements is enough to assure us that the properties are really there. That is, we do not need to know the momentum and position of the second particle to be assured that it has a definite momentum and position.
We conclude that the second particle must possess both a definite position and a definite momentum. The wave representing the second particle, however, will in general assign neither definite position nor definite momentum to it. Therefore, EPR conclude, the quantum wave is an incomplete description.
Are you sensing something familiar about the EPR argument but cannot quite place it? What was distinctive about Einstein's early work was his uncanny ability to find ways of revealing the hidden, inner structure of matter from its measureable properties. We saw this in Einstein's work of 1905 in statistical physics. ("Atoms and the Quantum.") There he looked at the measurable properties of sugar solutions--their viscosity and rates of diffusion--to infer to the size of molecules. The analysis of Brownian motion also led him to an estimate the the size of molecules. And, most revolutionary, he recognized the signature of atoms in the measurable thermodynamic properties of heat radiation. That led him to his light quantum hypothesis. In the EPR argument, we see something analogous. Einstein once again wants to reveal the hidden properties of quantum particles and he finds a way based on measurement to get at them. This time the measurements are on remote entangled systems.
The discussion above summarizes the EPR argument. However it does not fully expose the assumptions that it makes. For the argument to succeed, there are two assumptions needed and both have been subject to quite intense scrutiny in the literature.
The first is separability. EPR tacitly assume that two systems widely separated in space have independent existences, so that the state of one can be specified fully without consideration of the second.

23 October 1892 issue of Fliegende
Blätter https://commons.wikimedia.org/wiki/File:Kaninchen_und_Ente.svg
Is this an image of a duck or a rabbit? The question provides a
very crude illustration of the idea of nonseparability. It is
both rabbit and duck. Neither exist independently. Their existences are so
fully integrated that, if we take away one, we automatically take away the
other. One cannot be without the other.
The second assumption is locality. EPR assume that a measurement here cannot affect a system there if here and there are spacelike separated, that is, any influence propagating from one place to the other must proceed faster than light.

Radio News January 1924 p. 869
The idea of locality is illustrated by radio
transmission. A radio set in one place can only communicate with another
elsewhere by sending radiowaves that propagate at the speed of light. A
faster signal is precluded by relativity theory.
Both assumptions are disavowed by standard quantum mechanics. Entangled states violate separability and measurement collapse is instantaneous. The admissibility and persuasiveness of the EPR argument depend essentially on the extent to which one accepts these two assumptions. One might discard them just because quantum theory, our most successful theory of matter, does not adhere to them. Or one might adopt them precisely because one senses this is the beginning of the escape from the deeper woes of the measurement problem.
The EPR paper did clearly state one of its premises that is closely connected with these last two ideas. It is the "criterion of reality" that takes a definite stance on a central issue in philosophy: how do we know what is real and what is not:
Criterion of reality
"If, without in any way disturbing a system, we can predict with certainty
(i.e. with probability equal to unity) the value of a physical quantity,
then there exists an element of physical reality corresponding to this
physical quantity."
The EPR paper is the best-known expression of Einstein's argument against the completeness of quantum theory. The logic of the paper is a little more tangled than the sketch just given. There is clear evidence that Einstein felt the tangles unnecessary, attributing them to his co-author. He wrote shortly afterwards to Schroedinger of his concern (June 19, 1935):
"For reasons of
language this [paper] was written by Podolsky after several discussions.
Still, it did not come out as well as I had originally wanted; rather, the
essential thing was, so to speak, smothered by the formalism
[Gelehrsamkeit]. (Translation from
http://plato.stanford.edu/entries/qt-epr/)
"
Einstein's own later statements of the essential argument were much briefer and clearer. Here is version from his article "Physics and Reality" (Journal of the Franklin Institute, 221, 1936).
"Consider a
mechanical system consisting of two partial systems A and B which interact
with each other only during a limited time. Let the ψ function before
their interaction be given. Then the Schrödinger equation will furnish the
ψ function after the interaction has taken place. Let us now determine the
physical state of the partial system A as completely as possible by
measurements. Then quantum mechanics allows us to determine the ψ function
of the partial system B from the measurements made, and from the ψ
function of the total system. This determination, however, gives a result
which depends upon which of the physical quantities (observables) of A
have been measured (for instance, coordinates or momenta). Since there can
be only one physical state of B after the interaction which cannot
reasonably be considered to depend on the particular measurement we
perform on the system A separated from B it may be concluded that the ψ
function is not unambiguously coordinated to the physical state. This
coordination of several ψ functions to the same physical state of system B
shows again that the ψ function cannot be interpreted as a (complete)
description of a physical state of a single system. Here also the
coordination of the ψ function to an ensemble of systems eliminates every
difficulty.*
[Footnote] *A measurement on A, for example, thus involves a
transition to a narrower ensemble of systems. The latter (hence also its ψ
function) depends upon the point of view according to which this reduction
of the ensemble of systems is carried out.
"
Here's the version given in Einstein's Autobiographical Notes, written over a decade after the EPR paper. It is worth quoting at length since it surely represents Einstein's most considered view, expressed in the way he thought most fitting.
"There is to be a system that at the time t of our observation consists of two component systems S1 and S2, which at this time are spatially separated and (in the sense of the classical physics) interact with each other but slightly. The total system is to be described completely in terms of quantum mechanics by a known ψ-function, say ψ12. All quantum theoreticians now agree upon the following. If I make a complete measurement of S1, I obtain from the results of the measurement and from ψ12 an entirely definite ψ-function ψ2 of the system S2. The character of ψ2 then depends upon what kind of measurement I perform on S1.
Now it appears to me that one may speak of the real state of the partial system S2. To begin with, before performing the measurement on S1, we know even less of this real state than we know of a system described by the ψ-function. But on one assumption we should, in my opinion, insist without qualification: the real state of the system S2 is independent of any manipulation of the system S1, which is spatially separated from the former. According to the type of measurement I perform on S1, I get, however, a very different ψ2 for the second partial system (φ2, φ21, . . . ). Now, however, the real state of S2 must be independent of what happens to S1. For the same real state of S2 it is possible therefore to find (depending on one's choice of the measurement performed on S1) different types of ψ-function. (One can escape from this conclusion only by either assuming that the measurement of S1 (telepathically) changes the real state of S2 or by denying altogether that spatially separated entities possess independent real states. Both alternatives appear to me entirely unacceptable.)
If now the physicists A and B accept this reasoning as valid, then B will have to give up his position that the ψ-function constitutes a complete description of a real state. For in this case it would be impossible that two different types of ψ-functions could be assigned to the identical state of S2.
The statistical character of the present theory would then follow necessarily from the incompleteness of the description of the systems in quantum mechanics, and there would no longer exist any ground for the assumption that a future foundation of physics must be based upon statistics.
It is my opinion that the contemporary quantum theory
represents an optimal formulation of the relationships, given certain
fixed basic concepts, which by and large have been taken from classical
mechanics. I believe, however, that this theory offers no useful point of
departure for future development...
"
One remark is especially noteworthy since it makes clear the importance of locality and separability in Einstein's argument. He canvasses two possible escapes from his conclusion of the incompleteness of quantum theory. They are "measurement of S1 (telepathically) changes the real state of S2"--that corresponds to a violation of locality. The second is "denying altogether that spatially separated entities possess independent real states"--that is the violation of separability.
In plumbing the depths of Einstein's objections to quantum theory, his concern to preserve separability seems to be the deepest and most fundamental. Certainly separability is logically prior to locality. One cannot require systems here to interact locally with systems there unless one can already distinguish systems here from systems there. That distinction requires separability.
Here's one of Einstein's remarks on the question from a paper "Quantum Mechanics and Reality," Dialectica, 2 (1948), pp. 320-24:
"Without such an
assumption of the mutually independent existence ... of spatially distant
things, as assumption which originates in everyday thought, physical
thought in the sense familiar to us would not be possible. Nor does one
see how physical laws could be formulated and tested without such a clean
separation.
"
Translation from Don Howard, "Einstein on Locality and Separability," in Studies in History and Philosophy of Science, 16 (1985), pp. 171-201 on .
In all this, Einstein was defending a minority view in the physics community. The task of responding to Einstein was taken up by Niels Bohr. The debate in which they engaged was surely one of the monumental debates of the 20th century. Here were two titans of modern physics with quite opposed positions, struggling to establish their view of the meaning of the quantum.
| The great difficulty in following the debate, however, is that its canonical history has been written by Bohr in his contribution to the Schilpp Einstein volume. There one finds a story of a far-sighted Bohr, who recognizes the profound philosophical re-orientation brought by quantum theory; and a reactionary, recalcitrant Einstein unable to accommodate the novelty. Einstein's view was, we would expect, somewhat different. Unfortunately Einstein gave no extended, published account of his perspective on the debate. In private correspondence, he was quite disparaging of Bohr, calling him a "talmudic philosopher [who] doesn't give a hoot for 'reality,' which he regards as a hobgoblin of the naive..." (Einstein to Schroedinger, June 19, 1935. Translation from Don Howard, "Einstein on Locality and Separability," in Studies in History and Philosophy of Science, 16 (1985), pp. 171-201 on p. 178.) | Niels Bohr, "Discussions with Einstein on
Epistemological Problems in Atomic Physics" in, P. A. Schilpp, ed.,
Albert Einstein-Philosopher Scientist. 2nd ed. New York: Tudor
Publishing, 1951. Available online here. |
 |
It is easier to report on Bohr's views than to justify them. So let me attempt just to report and a few expressions of my own hesitations. The point of view advocated by Bohr was labeled "complementarity" by Bohr and its starting point was an insistence that we must describe experiments in classical terms: |
| "...it is
decisive to recognise that, however far the phenomena transcend
the scope of classical physical explanation, the account of all
evidence must be expressed in classical terms. The argument is
simply that by the word "experiment" we refer to a situation where
we can tell others what we have done and what we have learned and
that, therefore, the account of the experimental arrangement and of
the results of the observations must be expressed in unambiguous
language with suitable application of the terminology of classical
physics. " |
The "must be" seems to me excessive and
unwarranted. Somehow, in a way he does not describe, Bohr is able to
preclude the description of my sensing a flash of light as "I sensed
a photon" where photon is a term whose meaning is given by quantum
theory. Classical terminology is peculiarily well-adapted to ordinary sized objects since it arises in a theory designed to describe them. So it is easy to continue to use classical terms when we describe quantum experiments with ordinary sized objects. We should not confuse that comfort with our having no alternative. |
This led Bohr immediately to what seems to be the central idea:
"This crucial point,
which was to become a main theme of the discussions reported in the
following, implies the impossibility of any sharp separation between the
behaviour of atomic objects and the interaction with the measuring
instruments which serve to define the conditions under which the phenomena
appear. In fact, the individuality of the typical quantum effects finds
its proper expression in the circumstance that any attempt of subdividing
the phenomena will demand a change in the experimental arrangement
introducing new possibilities of interaction between objects and measuring
instruments which in principle cannot be controlled. Consequently,
evidence obtained under different experimental conditions cannot be
comprehended within a single picture, but must be regarded as
complementary in the sense that only the totality of the phenomena
exhausts the possible information about the objects.
"
| The main theme was then illustrated vividly and
effectively with a series of descriptions of the various measurement
devices, described in a "semi-serious" realistic style, in order to
make clear that performing one measurement precludes the performing
of another. For example, a position
measurement on a propagating particle might employ a slit
firmly bolted to the bench through which the particle passes. We
then know the exact height of the particle when it passes through
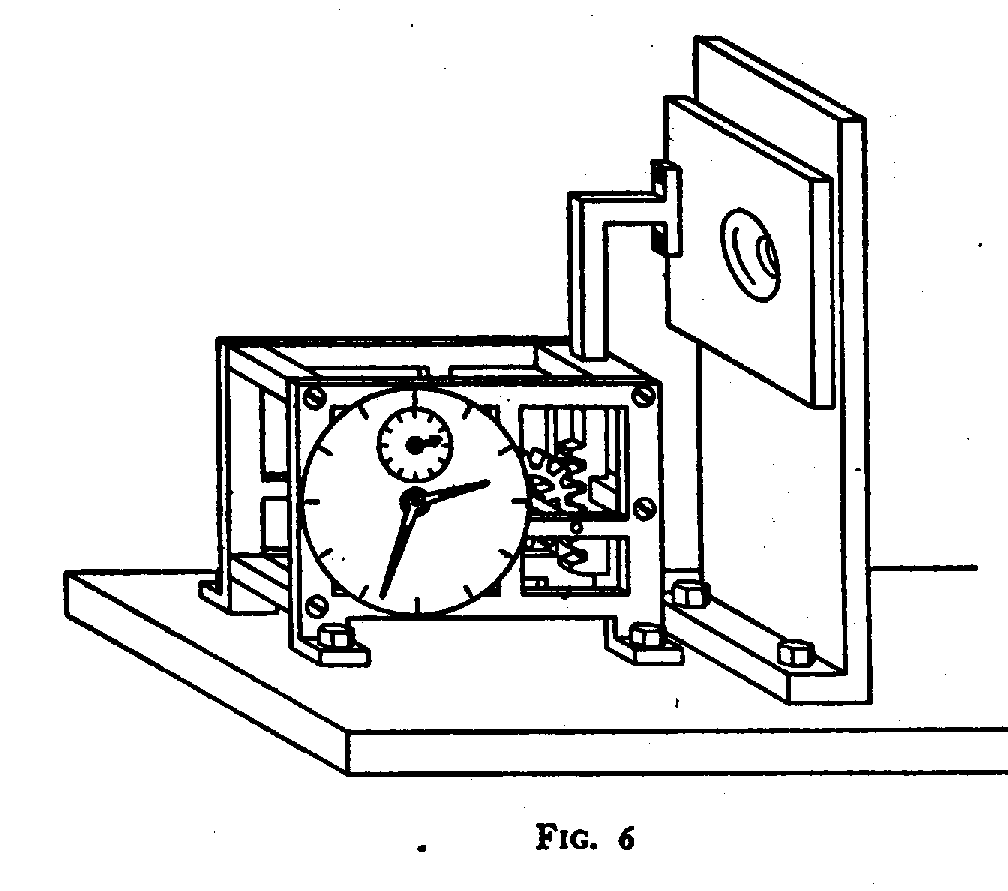
the slit. The images illustrating Bohr's arguments opposite and below are reproduced from Bohr's narrative in his "Conversations with Einstein ..." They are so familiar that any present-day philosopher of physics recognizes them immediately as coming from Bohr's narrative. Any philosopher of physics with some understanding of perspective drawings will also recognize that the figures were not draw correctly in the sense that the parallels lines in the three-dimensional objects are not represented by lines in the drawing that converge onto a distant vanishing point. Instead they are drawn parallel in the drawing as they would be in an isometric, engineering drawing. The effect is that they appear slightly distorted to an eye used to looking at perspective drawings. |
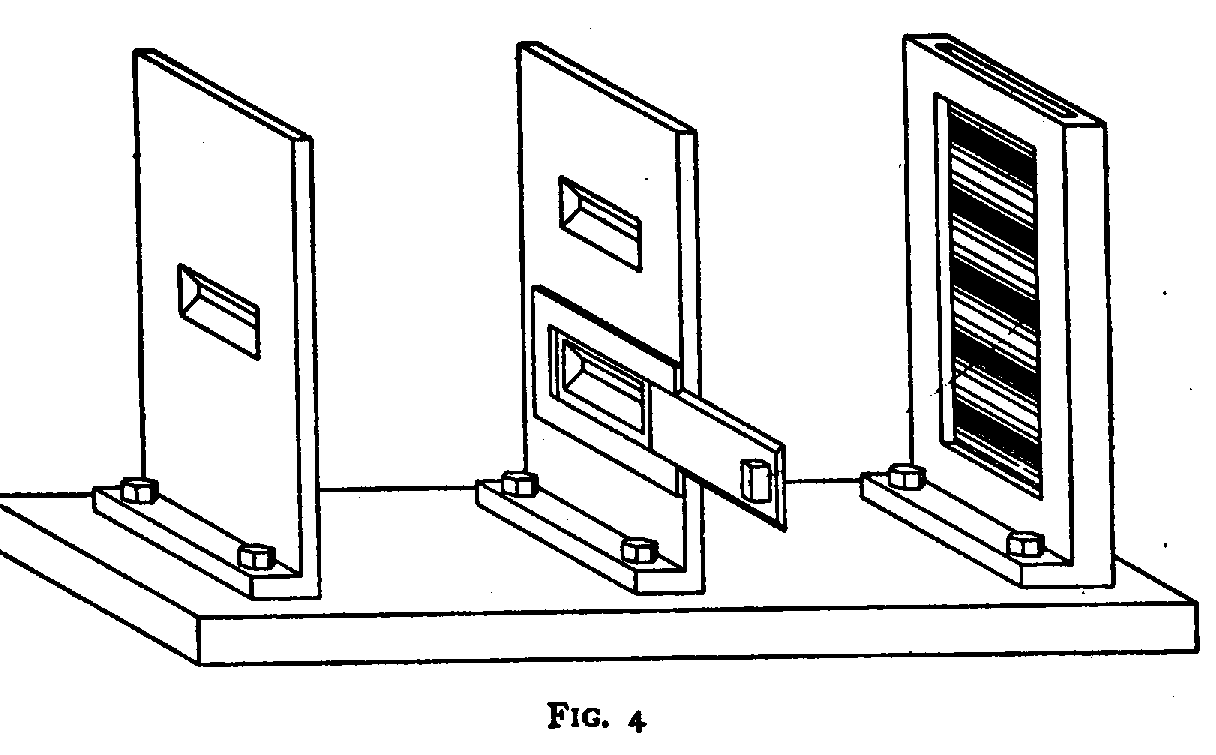 |
|
|
A momentum measurement,
however, would require a movable slit, whose recoil under the
passage of the particle would let us determine the size of a
momentum transfer to or from the particle. That essential
moveability of the slit precluded the fixed slit arrangement of the
position measurement. The mutual exclusivity of the two arrangements of measurement apparatus is reflected in the complementarity of the quantities of position and momentum. |
Bohr then developed further examples, included the celebrated Einstein "photon in a box" thought experiment.
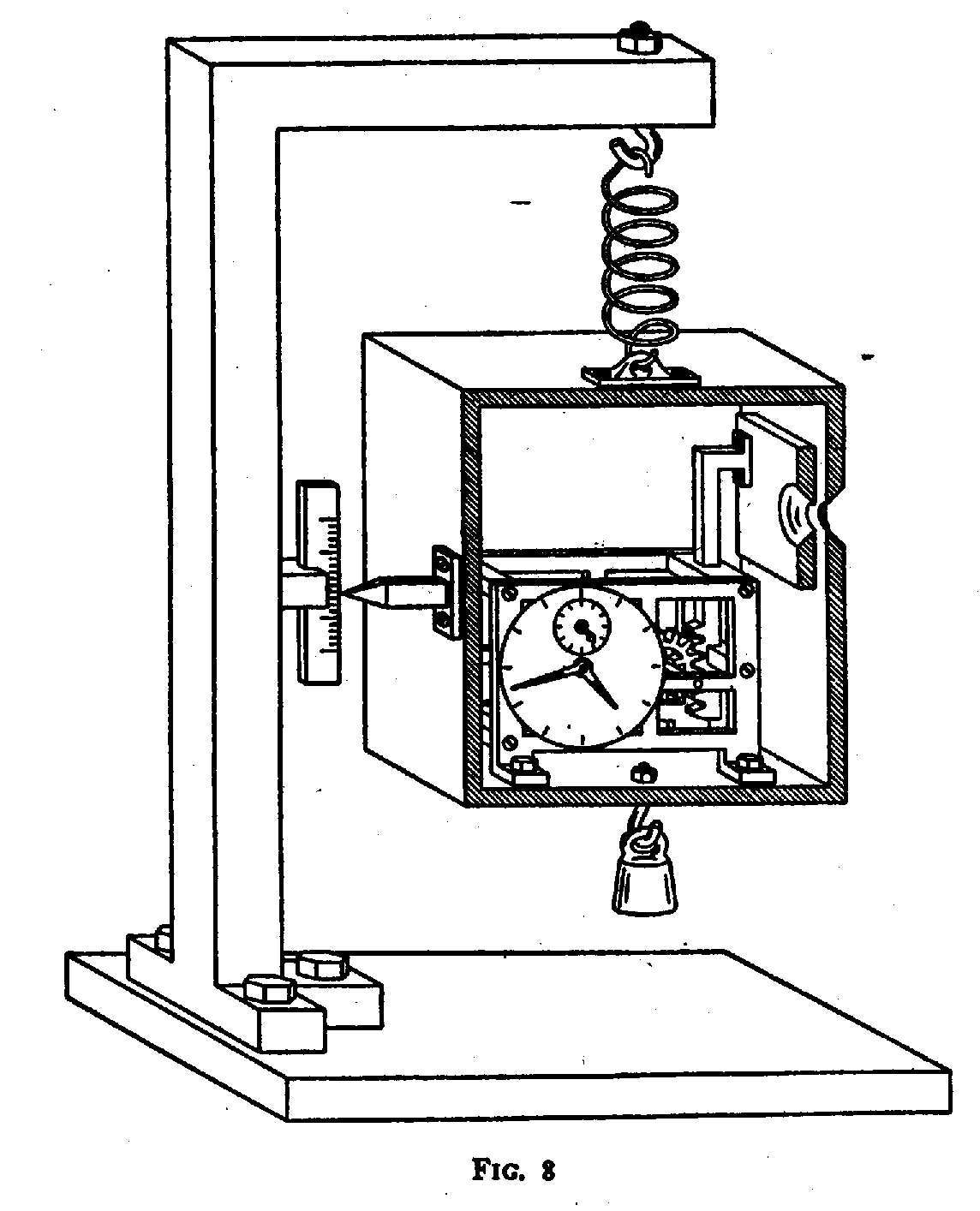
Overall, on Bohr's account so far, Einstein's approach was decisively defeated in the resulting analyses. Needless to say, that did not seem to be Einstein's view. You can read the details of Bohr's discussion in his text and, for a suggestion on Einstein's side, see Don Howard, "Revisiting the Einstein-Bohr Dialogue."
All this display of realistic measuring devices was a prelude to Bohr's response to the EPR argument. In giving it, he quoted from an earlier text he'd written that captured his central response
"From our point of
new we now see that the wording of the above-mentioned criterion of
physical reality proposed by Einstein, Podolsky, and Rosen contains an
ambiguity as regards the meaning of the expression ' without in any way
disturbing a system.' Of course there is in a case like that just
considered no question of a mechanical disturbance of the system under
investigation during the last critical stage of the measuring procedure.
But even at this stage there is essentially the question of an
influence on the very conditions which define the possible types of
predictions regarding the future behaviour of the system. Since
these conditions constitute an inherent element of the description of any
phenomenon to which the term "physical reality" can be properly attached,
we see that the argumentation of the mentioned authors does not justify
their conclusion that quantum-mechanical description is essentially
incomplete. On the contrary, this description, as appears from the
preceding discussion, may be characterised as a rational utilisation of
all possibilities of unambiguous interpretation of measurements,
compatible with the finite and uncontrollable interaction between the
objects and the measuring instruments in the field of quantum theory. In
fact, it is only the mutual exclusion of any two experimental procedures,
permitting the unambiguous definition of complementary physical
quantities, which provides room for new physical laws, the coexistence of
which might at first sight appear irreconcilable with the basic principles
of science. It is just this entirely new situation as regards the
description of physical phenomena that the notion of complementarity
aims at characterising.
"
| This explanation of the purported failure of the EPR argument is not easy to comprehend on a first reading. One expects it to become clearer on re-reading. My experience is that this does not happen and I have been unable to find a cogent interpretation of the text. Whether we should persevere or not remains an issue that divides the philosophy of physics community. One part remains convinced that Bohr's insights were profound, but poorly expressed, and we should keep seeking their deeper insights. Another holds that Bohr had vivid thoughts that he believed, mistakenly, solved foundational problems; but these thoughts were incoherent and the opacity of Bohr's writing is simply a result of that incoherence. |  I
belong to the second group that finds Bohr's thought opaque. My best
efforts find Bohr advocating a kind of ultra-empiricism that
entangles epistemology (how we know things) with ontology (what
things are). The idea is that what something is, is inseparable from
how we actually happened to find out about it. I
belong to the second group that finds Bohr's thought opaque. My best
efforts find Bohr advocating a kind of ultra-empiricism that
entangles epistemology (how we know things) with ontology (what
things are). The idea is that what something is, is inseparable from
how we actually happened to find out about it. The EPR argument requires us to imagine two different measurements that we might perform on the first system; and from their possible outcomes we infer to the properties of the second. EPR presume that it is possible to know what would happen were two different measurements performed on the system. Bohr's ultra-empiricism asserts that the two systems would not be the same system if different measurements were performed on them. For what the system is, involves essentially which measurement is performed on it. What EPR think of as one system, explored by different measurements, is, for Bohr's ultra-empiricism, two different systems. It follows that EPR are mistaken in imagining that the two measurements could be performed on the very same system. The first steps of the EPR argument are blocked. While this seems to be Bohr's argument, it is opaque to me why Bohr thought this ultra-empiricism is compatible with quantum theory. It amounts to a denial that quantum theory supports what philosophers call "counterfactuals"--statements of what would have happened were, contrary to actual fact, some other conditions to be the case. Quantum theory clearly supports counterfactuals. When we have a spread out quantum wave representing some particle, standard algorithms in the theory tell us what would happen were we to perform this measurement, or, instead of it, had we performed that measurement. Generally the description of what would happen is expressed in terms of the probabilities of various outcomes. But there is no difficulty in recovering the result. Thus, there seems no problem as far as quantum theory is concerned when EPR assert what would happen were this measurement or another incompatible measurement to be performed. |
Einstein's direct response to Bohr's analysis in the same volume was terse, even severe:
"...it must seem a
mistake to permit theoretical description to be directly dependent upon
acts of empirical assertions, as it seems to me to be intended [for
example] in Bohr's principle of complementarity, the sharp formulation of
which, moreover, I have been unable to achieve despite much effort which I
have expended on it. From my point of view [such] statements or
measurements can occur only as special instances, viz., parts, of physical
description, to which I cannot ascribe any exceptional position above the
rest.
"
Not many scholars have the distinction of being told in print by Einstein that he has been unable to discern precisely what they are asserting "despite much effort"!
While the final outcome of the debate remains controversial in the philosophy of physics literature, I can state my own view. In his debate with Bohr, Einstein won. Einstein's argument is clear and powerful. Bohr's claims are either obscure or indefensible.
One can win a battle, but lose the war. And that is what happened. Einstein won the debate with Bohr, in my view. In his debate with quantum theory, Einstein lost and unequivocally so.
The reason for his loss did not emerge during his lifetime. They came in the decade after his death through the work of John S. Bell. The story of Bell's work and the flood of work it inspired is too large a topic to treat adequately in this short section. We can see only some preliminary fragments here.

John S. Bell
https://commons.wikimedia.org/wiki/File:John_bell_2.png
What Bell noticed was a lacuna in Einstein's argument. Einstein correctly noted that measurements on a system would enable the prediction of outcomes of measurements on another system entangled with it. By imagining different possible measurements on the first system, one then merely used the computations of the quantum theory to determine the outcomes of the measurements on the second system.
| Einstein presumed that all these possible outcomes reflected properties possessed by the second system. That meant that all possible measurements would end up revealing a single set of possessed properties. What Bell showed was that this last assumption failed. If one assumed that the computations of quantum theory correctly predicted the outcomes of measurement, then there was no single set of hidden properties consistent with all possible measurements. Or, more precisely, if one assumed separability and locality, then there was no such set. | The situation is not so removed from
the familiar parable of the blind men and the elephant, but with an
essential twist. In it, several blind men feel different parts of
the elephant, each imagining a very different animal on the basis of
the limited portion they sensed. We, however, recognize that each part they describe can be fitted together to describe the one familiar animal. In the quantum case, however, each of the different measurements yields results that cannot be fitted together to describe a single independent reality. So it is as if the blind men report parts that cannot be integrated consistently into one animal. 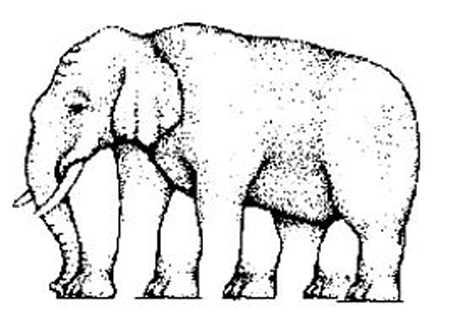 |
Bell's arguments cannot be developed here. They go beyond the ideas developed above. But they do so only in the technical details, not in matters of basic principle. To begin, Bell set his analysis in the context of a version of the EPR argument laid out by David Bohm. That version was devoted specifically to the measurement of the angular momentum of particles.
Where ordinary, linear momentum measures the conserved quantity of motion associated with motion in a straight line, angular momentum measures a corresponding conserved quantity associated with rotation. In macroscopic bodies, it is associated with their rotational motion. Particles, such as electrons, also carry an intrinsic angular momentum, but it is no longer associated with a rotational motion of the electron. Nevertheless, in spite of the danger of misunderstanding, the quantity is still called "spin."
The reformulation in terms of spin greatly simplifies the analysis since all electrons carry the same spin in magnitude. Where they differ is just in the axial direction of the spin. We can create an entangled pair of electrons in a "singlet state" with total angular momentum of zero. If one has a spin axis that points "up," then, since the total angular momentum sums to zero, the other electron has a spin axis that points "down." As before, measuring the "up-down" spin on one electron and finding "up" allows us to determine what the measured spin will be on the other electron. It is "down."
We could instead have measured the "left-right" spin on the first electron. If the first electron is found to have spin "right," then the second must have spin "left."
Bell then assumed that entangled systems do have properties that conform with Einstein's expectations of separability and locality. Whatever spin properties are revealed by these measurements, he assumed, they are truly held by both electrons. The complication is that some of the measurement outcomes are only delimited by quantum theory through the probabilities of which spin states will show up in the measurements. For example, if we measure the spin "up-down" state of an electron that truly has spin "right," then there is a probability of 0.5 that the measurement returns spin "up"; and a probability of 0.5 that it returns spin "down."
It follows that measurements aimed at discerning the hidden spin states of the electrons give scattered results, conforming with these probabilities. In general, we cannot be sure which ones will appear in any one measurement. However, in many repeated experiments, definite trends will emerge. They will take the form of correlations between the outcomes returned by measurements on each of the two entangled particles. What Bell showed is that a characteristic parameter of these correlations will always lie in a small interval of values. The assertion that they lie in this interval is the Bell inequality. In later treatments, this interval spanned -2 to +2.
The parameter of Bell's inequality can also be determined by assuming that the measurements conform to the predictions of the quantum theory. The result of that calculation is that the parameter is greater than 2 for the particular case treated. It follows that a theory conforming to Einstein's expectations cannot yield the same predictions as quantum theory. Since we have high confidence in quantum theory's predictions, this was taken as a demonstration of the failure of Einstein's assumptions.
The photo above of John S. Bell shows him standing in front of a blackboard on which the relevant quantities are written, as the detail of the photo below shows. That is, the "Einstein" absolute value is 2 or less, but the "QM" (quantum mechanical) value is 2√2≅2.82.
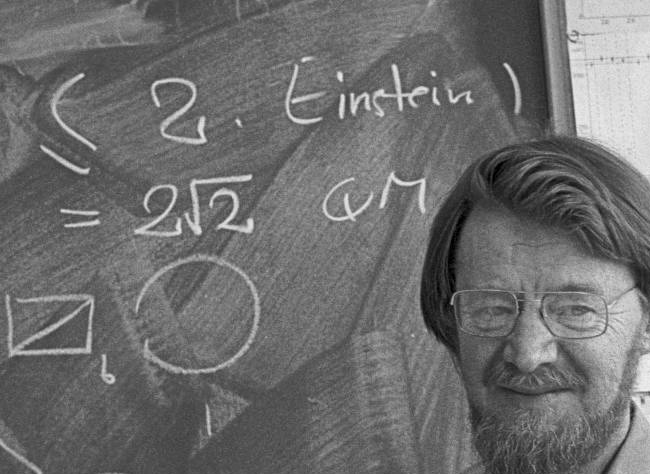
There was a loophole. Was it possible that the predictions of quantum theory were incorrect on this parameter? Later experiments, such as reported by Alain Aspect in 1981, affirmed that the quantum predictions were correct. The loophole was closed.
The final outcome is that the EPR argument for the incompleteness of quantum theory fails. Whatever reality lies behind quantum processes does not conform to the presumptions of the EPR argument. We cannot keep both separability and locality. Something has to be given up.
For more see Abner Shimony, "Bell's Theorem", Stanford Encyclopedia of Philosophy.
An appreciation of Bell's arguments requires requires
comfort with the quantity, spin. It is possible, however, to see how the
EPR argument fails using only the simple notions of waves and wave
collapse that we've developed so far. For such an account see my
"Little boxes: A
simple implementation of the Greenberger, Horne, and Zeilinger result
for spatial degrees of freedom," American Journal of Physics,
79(2)(2011), pp. 182-188.
So what should our verdict be of Einstein's recalcitrance in the face of the new quantum theory? Here is my view. Einstein was wrong in his suppositions of separability and locality in the quantum domain. In his time, they were entirely reasonable demands and it was very hard to see then that they would fail. That they do fail is the lesson we have now learned.
However, in my view, he was not wrong to resist the foundational accounts that surrounded quantum theory in his final decades. He was quite right to protest that no account of the quantum domain could so glibly give up the notion of reality as they did. All was not well then in our accounts of the quantum domain; and all is not well now. The clearest indication of the trouble is the persistence of the measurement problem. It shows us that there is something quite unresolved in the foundations of quantum theory.
In the early years of the theory, as new empirical and theoretical advances came in rapid succession, it was easy to overlook these problems. It is not hard to image the pressures faced by any critic of a new, rising theory. Any new theory has small problems that will be resolved soon enough, we can imagine Einstein hearing from the theory's proponents. These problem are not usually reasons for grave concern. Why should one doubt a theory will such a prodigious record of success? Why hold up real progress with quibbles? Is it not expedient to suspend criticism?

It took a thinker of strong character and principle to stand up to the pressures of this expedient view. That thinker was Einstein and he had little company in his hesitations. The photo above of Einstein leaving (I think) the Institute for Advanced Study in Princeton is a poignant image of a man walking alone. He wrote to Schroedinger on May 31, 1928, at the very start:
"The Heisenberg-Bohr tranquilizing philosophy--or religion?--is delicately contrived that, for the time being, it provides a gentle pillow for the true believer from which he cannot very easily be aroused. So let him lie there."
Quoted from Arthur Fine, The Shaky Game.University of Chicago Press, 1988, p.18.
While we now may not agree with the nature of Einstein's positive complaints concerning the newly emerging quantum theory, it is now abundantly clear that something was not and is not right with the theory. In hindsight that we see that Einstein's resistance was appropriate and should be celebrated. I can see that clearly now, but I doubt that I would have had the clarity and character to see it in 1928. I do not have the insight and principle of Einstein.
Copyright John D. Norton. March 27, April 11, April 20, 30, 201; January 30, 2013. November 18, 2020. February 6, April 21, 2022. December 2, 2024