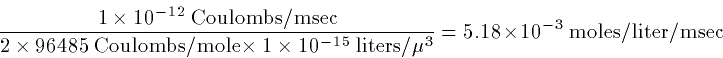

Next: Exploring the Hodgkin Huxley
Up: Calcium dynamics and IK-AHP
Previous: Calcium dependent potassium
Many modelers define the conductances, etc in absolute terms, such as
a capacitance of, say, 0.29 nF. Most of the time, I will define my
units in terms of ``size per unit area,'' but some of the models I
describe (in particular, the big cortical mix and match model) will be
in absolute numbers. A modeler who does that is making an implicit
assumption about the size of the cell. For example, in the above
capacitance example, if I assume a capacitance value of 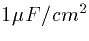 then 0.29 nF corresponds to a cell with a total membrane area of
29000
then 0.29 nF corresponds to a cell with a total membrane area of
29000 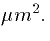 Given that typical conductances are in units of
mS/cm2 then typical absolute conductances would be of the order of
microsiemens, currents are in nanoamps, capacitance in nanofarads. In
McCormick's and Huguenard's model (J. Neurophys 68:1373, 1384) sodium
has a conductance of 12
Given that typical conductances are in units of
mS/cm2 then typical absolute conductances would be of the order of
microsiemens, currents are in nanoamps, capacitance in nanofarads. In
McCormick's and Huguenard's model (J. Neurophys 68:1373, 1384) sodium
has a conductance of 12 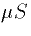 for the 29000
for the 29000 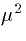 cell
which translates into 41 mS/cm2. (Make sure you can do this
calculation - keep in mind that
cell
which translates into 41 mS/cm2. (Make sure you can do this
calculation - keep in mind that 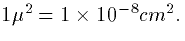
Since currents are in nanofarads, let's see what the conversion factors
are for the influx of calcium. Recall that a farad is a coulomb per
second. The Faraday constant, F has units of coulombs per
mole. Concetration is moles per liter, so that we need to know the
volume in which the calcium is relevant. Volume is area times depth,
so if we take a depth of 100 nM under our spherical cell, we can
figure out the volume. Thus, with a
linear uptake in cacium, the
calcium concentration is satisfies:
![\begin{displaymath}
\frac{d[Ca_i]}{dt} = -k I_{Ca} /(d\cdot A) - \beta [Ca_i]\end{displaymath}](img61.gif)
where 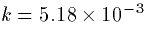 to convert current (nanoamperes), time
(milliseconds) and volume (cubic microns) to concentration in
moles/liter. (To see this, note
to convert current (nanoamperes), time
(milliseconds) and volume (cubic microns) to concentration in
moles/liter. (To see this, note
Thus
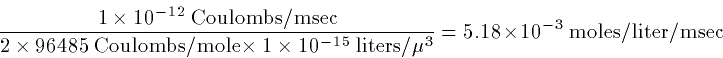
)


Next: Exploring the Hodgkin Huxley
Up: Calcium dynamics and IK-AHP
Previous: Calcium dependent potassium
G. Bard Ermentrout
1/29/1998
![\begin{displaymath}
\frac{d[Ca_i]}{dt} = -k I_{Ca} /(d\cdot A) - \beta [Ca_i]\end{displaymath}](img61.gif)
![\begin{displaymath}
\frac{d[Ca_i]}{dt} = -k I_{Ca} /(d\cdot A) - \beta [Ca_i]\end{displaymath}](img61.gif)