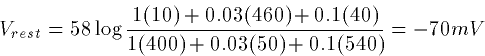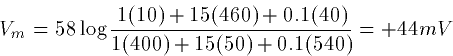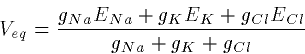

Next: Ion concentrations and equilibrium
Up: Ionic basis of the
Previous: Nernst Equation
In the presence of several different ions, the equilibrium of the cell
depends on the relative permeability of the ions. For this, we use
the Goldman-Hodgkin-Katz equation:
| ![\begin{displaymath}
V_{rest} = \frac{RT}{F} \ln \frac{P_K [K^+]_{out} +P_{Na} [N...
...{in}}{P_K [K^+]_{in} +P_{Na} [Na^+]_{in}
+ P_{Cl} [Cl^-]_{out}}\end{displaymath}](img7.gif) |
(2) |
Permeability of an ion is dependent on a number of factors such as the
size of the ion, its mobility, etc.
During rest in the squid giant axon, the permeabilities have the ratio
PK:PNa:PCl = 1:0.03:0.1 so that
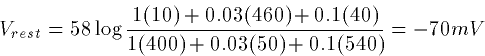
Since PK dominates, this is close to EK.
During an action potential the ratio is PK:PNa:PCl =1:15:.1
so that
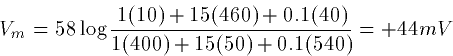
Later on we will approximate the GHK equations by a linearized
version:
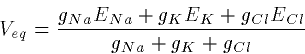
where the conductances g are proportional to the permeabilities.
HOMEWORK
- 1.
- Suppose the external potassium in a mammalian cell is increased by a
factor of 10. What is the new value of EK?
- 2.
- At
 a cell contains 80 mM sodium inside and has only
100 mM sodium outside. What is the equilibrium potential for sodium?
a cell contains 80 mM sodium inside and has only
100 mM sodium outside. What is the equilibrium potential for sodium?
- 3.
- Using the same permeabilities for the mammalian cell as were
used for the squid axon, compute Vrest,Vm using the table
below.


Next: Ion concentrations and equilibrium
Up: Ionic basis of the
Previous: Nernst Equation
G. Bard Ermentrout
1/10/1998
![\begin{displaymath}
V_{rest} = \frac{RT}{F} \ln \frac{P_K [K^+]_{out} +P_{Na} [N...
...{in}}{P_K [K^+]_{in} +P_{Na} [Na^+]_{in}
+ P_{Cl} [Cl^-]_{out}}\end{displaymath}](img7.gif)
![\begin{displaymath}
V_{rest} = \frac{RT}{F} \ln \frac{P_K [K^+]_{out} +P_{Na} [N...
...{in}}{P_K [K^+]_{in} +P_{Na} [Na^+]_{in}
+ P_{Cl} [Cl^-]_{out}}\end{displaymath}](img7.gif)