NMDA

An important receptor found in cortical pyramidal neurons is the NMDA receptor. They are quite slow with rise times of about 20 msec and decay times of 25 to 120 msec. A fairly good simple model for NMDA is

$$I_{NMDA} = \bar{g}_{NMDA} B(V) s(t) (V-V_{NMDA})$$

where s(t) obeys first order dynamics. There is an important difference between NMDA and AMPA. The conductance depends in a complex fashion on the postsynaptic potential via the term B(V). This voltage dependent conductance depends on the level of external magnesium ions. Here is a physiological correlate of the Hebb rule that both pre- and postsynaptic cells must be coincidently active. The voltage dependence is mediated by magnesium ions which normally block the NMDA receptors. Thus, the postsynaptic cell must be sufficiently depolarized to knock out the magnesium ions. This is modeled in Jahr and Stevens, J. Neuroscience 10, 1830-1837:

$$B(V) = \frac{1}{1+ e^{-.062V}[Mg^{2+}]/3.57}$$

By blocking the magnesium, it is possible to eliminate the voltage dependence and measure the kinetic parameters. A simple first order model is

$$\frac{ds}{dt} = \alpha [T] (1-s) - \beta s$$

as in the AMPA current, but , 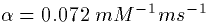$\alpha=0.072 \ mM^{-1} ms^{-1}$,$\beta=0.0066 \ ms^{-1}$ and $V_{NMDA}=0\ mV.$