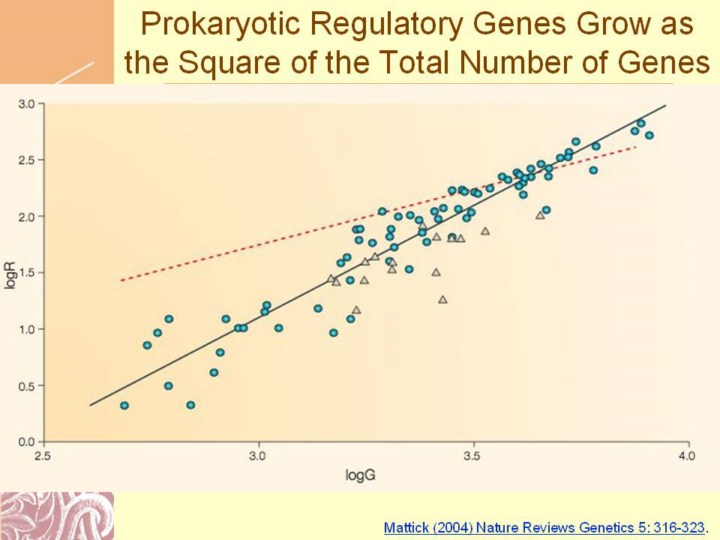Mattick (2004) Nature Reviews Genetics 5: 316-323

Double-logarithmic plot of the number of genes that encode regulatory proteins (R) against the total number of genes (G) for bacteria (circles) and archaea (triangles).The log�log distribution is well described by a straight line with slope 1.96 �0.15 (95% confidence interval), corresponding to a quadratic relationship between regulator number and total gene number (note that if R = ANx, a log transformation yields logR = xlogN + logA, in which the slope of the resulting line is equal to the exponent x). Dashed lines show the best linear fit to the data. Modified from REFS 18,19.