Infinite Sets

John D. Norton
Department of History and Philosophy of Science
University of Pittsburgh
http://www.pitt.edu/~jdnorton

The paradoxes of the infinitely large pose a foundational problem: can we really accept their results. Is there not something wrong with an infinite hotel that can always make room for more guests? Should we not draw the line at infinities of bodies whose interactions in ordinary collision mechanics lead to violations of the laws of conservation of energy and momentum? Perhaps there is a contradiction lurking in the paradoxes. That view was long held. The more recent view, as we shall now see, is just to accept that they are counter-intuitive, but not inherently contradictory; and they point to a new way to think about infinities.

Potential and Actual Infinities

The oldest response to these paradoxes was to take a severe option that can be traced back as far as Aristotle. According to it there is something wrong with the very idea of a completed infinity. The notion should be excised from our analyses.

Since something in the neighborhood of infinity seems useful in our thinking, Aristotle distinguished two senses of infinity: the potential and the actual. We have seen earlier how Aristotle employed the distinction in his response to Zeno's paradoxes of the dichotomy and the Achilles.

A potential infinity is characterized by its incompleteness. It manifests in systems in which an extension is always possible. Consider, for example, a sphere in ordinary space:

Potentially, a sphere can always be made larger. There is no largest sphere. The idea of an actually infinite sphere is literally nonsense. That is, it is a term "infinite sphere" that refers to nothing. For the surface of infinite sphere would have to consists of points infinitely far away from its center. But there are no such point "at infinity." Every sphere in a Euclidean space is some finite distance from a center point. The infinity is merely a potential infinity: there is no largest radius. For any radius we pick, say 10, 100, 1000, ..., there is always a larger one, 11, 101, 1001, ... Infinity is not a place picked out by the nonsensical notion of "infinite radius," where we somehow imagine that very, very distant points live.

Here is a fancier case in which avoiding actual infinities is the right thing to do. I have argued that the "thermodynamic" limit in statistical physics should be treated as arising from potential infinities of components. If it is understood in terms of actual infinities, the limit produces undesired, pathological behavior in thermal systems. John D. Norton, "Approximation and Idealization: Why the Difference Matters" Philosophy of Science, 79 (2012), pp. 207-232.

This last example shows that an aversion to actual infinities is not always mistaken. To insist that there are infinite spheres in a Euclidean space directly contracts the property of a Euclidean space that every point in it is some finite distance from any nominated starting point. The appropriate attitude is selective. Sometimes we should limit ourselves to potential infinities. Sometimes we should not. The troublesome view is on that issues a blanket prohibition on actual infinities.

Potential Infinities in Euclid's Elements

There is a striking example of a most effective and useful branch of mathematics that employed only potential infinities. This is the geometry codified by Euclid in the 4th century BC. His Elements of Geometry was the definitive treatise in geometry for millennia. It provided Newton with the mathematics he needed to formulate his celebrated system of the world.

Here is the title page of the first English edition (1582) of Euclid's Elements, translated from the Greek:

The foundation of Euclid's geometry lay in his five postulates. They asserted what exists in the world of geometry. The rest of geometry is to be constructed from what these five postulates say exists. Here is how the first two postulates appeared in this first English edition. (This early edition does not use the not standard term of "postulate.")

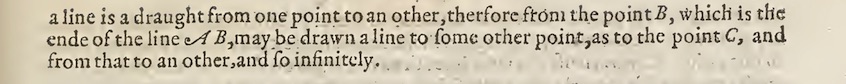
These postulates contain a similar concession to possible infinity, as opposed to an actual infinity. The first postulate says from a later translation:

1. Let the following be postulated: to draw a straight line from any point to any point.

English from Heath translation, Cambridge Univ. Press, 1908, p. 195-96.

Euclid's wording is, unfortunately sufficiently ambiguous that this postulate might allow for an actually infinite line to be produced. I incline against this possibility since an actually infinite line essentially never appears in the full 12 books of Euclid's Elements. The term infinite appears about a dozen times in one translation. However the translation gives the corresponding Greek, which is "apeiron." I understand it mean "unbounded" not the actual infinity connoted by the modern use of the word "infinite." When appended to a line, I read it just to convey that the line can always be extended indefinitely. Greek text of J.L. Heiberg, translation Richard Fitzpatrick, http://farside.ph.utexas.edu/Books/Euclid/Elements.pdf

What is key here is what is not said. We would now want to say "There are infinitely long straight lines." Euclid instead just says that we can take any interval, finite in length, and produce it. Here I assume that "produce" means just "add any finite length to." If that is so, the extension can be continued indefinitely. However, no matter how many finite extensions we apply, since there are only finitely many of them, we do not arrive at a completed infinity, which would be the infinite straight line.

Perhaps Euclid limited his treatise to potential infinities through a concern for the trouble that actual infinities might cause. Or perhaps he just did not need actual infinities. In any case, the restriction did not harm the position of Euclid's Elements as the definitive treatise in geometry for thousands of years after him.

Potential and Actual Infinities in Sets of Numbers

We can follow the Aristotelian spirit and apply the same thinking to sets of numbers. There are many possible finite collections of numbers. We might have

These collections manifest a potential infinity. We can keep writing down new collections. No matter how many we write down, we can always add more. Each of these collections is extendable. We can always add more numbers to them; and then more again. This notion of potential infinity is, we are to suppose, sufficient to handle responsibly all the problems that arise concerning infinities.

Where a potential infinity is always incomplete, an actual infinity is complete. The totality of all the numbers is an actual infinity. They are all there. There is no new number that can be added to them.

All the paradoxes of the infinitely large depend on entities with completed infinities. If they are prohibited, then the paradoxes are gone. While this prohibition solves the problem, the general principle in solving paradoxes is to do the least harm: do not prohibit any more than really needs to be prohibited. This prohibition against actual infinities has been judged to go too far in the mainstream of mathematical thought, as we shall now see.

While this is a majority view in mathematics, there is still a minority finitist tradition that avoids actual infinities. We saw an example of its thinking in the question of the truth of propositions in arithmetic and its relation to supertasks.

Two Ways to Compare the Sizes of Sets

There is a less severe way of responding to the paradoxes of the infinitely large. There are, we shall see, two ways in which we compare the sizes of sets. Each by itself is unproblematic. However if we apply both to the same situation they can give different results. If we do this without realizing that we are using two different ways for comparing, we end up with conflicts that appear paradoxical. Once we realize that incautious application of both ways can lead to confusion, it becomes easier to see how we can treat infinities without immediately immersing ourselves in paradoxes.

Comparison by inclusion

If we have two sets such that one is properly included in the other, that is, one is a proper subset of the other, then the first is smaller. The "proper" means that every element of the first set is in the second but the second has some elements not in the first.

if we picture sets as areas, proper inclusion arises when the smaller set occupies a smaller area in the figure than the larger set.

Under this criterion, the set of even numbers is smaller than the set of numbers:

This sense of comparison is familiar and extends to antiquity. Euclid began his Elements by listing "axioms." These are propositions whose truth is so obvious that no there is no room for challenge. They are self-evident truths. They are the solid starting point of 12 books on geometry. They include such straightforward observations as:

Axiom 9. "The whole is greater than its part, and equal to the sum of all its parts."

Here how this axiom appeared in the first English edition (1582) of Euclid's Elements:

Comparison by inclusion, more precisely

Inclusion as a way of determining the sizes of sets is limited in its application since it requires that suitable subset relations hold before the considerations of inclusion can apply.

It is almost irresistible to proclaim: "But (1,3} and {2,4} both have two members and they can be put in one-to-one correspondence." True! But these considerations are those that arise in the second way of comparing set sizes, by cardinality.

For example, it is easy to see that the singleton set {1} is smaller than the set {1, 2}, since {1} is a proper subset of {1, 2}. We would like to say, however, that {1, 3} and (2, 4} have the same size. However, since neither is a subset of the other, considerations of inclusion fall silent. The problem remains when we deal with infinite sets. We would like to say that the set of odd numbers:

However, we cannot since there are no suitable subset relations holding between them.

The following definitions allow us to proceed with slightly greater precision and avoid the problems just noted. Both conditions can only be applied in cases in which the two sets stand in suitable subset relations.

A set A is no larger than a set B under the criterion of inclusion, just if A is a subset of B.

The awkward "no larger than" condition allows the definition to be formulated in terms of subsets, as opposed to proper subsets. That leaves open the possibility that A and B are same size. Leaving this possibility open enables us to give a definition of what it is for two sets to have the same size.

Sets A and B have the same size under the criterion of inclusion, just if A is no larger than B and B is no larger than A

To see how this works, take the most general case of two sets A and B, such that A and B have a non-empty intersection. In a diagram, they appear as:

The condition for sameness of size has two parts. The first requires that A is no larger than B; that is A is a subset of B. That means that the part of A that does not intersect with B is empty:

The second condition requires that the B is no larger than A; that is, that B is a subset of A. That means that the part of B that does not intersection with A is empty.

Self-test puzzle. Apply these conditions to the four sets:
A = {1, 2, 3, 4, 5, 6, 7, 8, 9}
B = natural numbers less than 10
C = {2, 4, 6, 8}
D = {0, 1, 2, 3, 4}

Answer

After these restrictions, it follows that sets A and B are the same set and thus have the same size.

A set A is smaller than a set B under the criterion of inclusion, just if A is a proper subset of B.

These seem such natural ways of comparing the sizes of sets that, at first, it seems hard to imagine that there might be anything to conflict with them.

Comparison by cardinality

"Cardinality" is a term of art in mathematics that will be explained shortly. Loosely speaking, it is a number that gives the size of a set. For finite sets, the notion is familiar. The four-membered set {u, v, x, y} has cardinality 4 or the cardinal number 4.

A second way of comparing the sizes of sets is equally familiar. Most of us do not know how many socks we have. However we are fairly sure that we have as many left socks as right socks. Or that is true at least for those of us who neatly store their stocks rolled together as a matched pair of left and right socks. (As a useful fiction, I am imagining that we mark each pair of socks as left and right.)

The criterion used is that the two sets of socks--left and right--are the same size just if we can map them one-to-one to each other. They are equinumerous.

This idea of the one-to-one mapping is key to the whole analysis. We will be using it again and again. Here is the more formal treatment of it.

Self-test puzzle: Are the mappings in this table really one-to-one? How would you show that they are?

Under this criterion, there are as many numbers as there are even numbers; and as many numbers as there are square numbers.

In general, any infinite subset of the natural numbers is equinumerous with the natural numbers and can be mapped one-to-one to them in this way.

If we think of subsets as areas, as in the figure above, then this one-to-one mapping appears as an expansion of the smaller area such that it fills the larger area exactly:

The effect of the expansion is to induce a one-to-one mapping from points in the smaller area to points in the larger area.

This criterion of one-to-one correspondence is a robust means of comparing the sizes of sets. It can be used with finite sets and with infinite sets. When two sets are equinumerous in this sense, we say that they have the same cardinality; or that their size is given by the corresponding cardinal number. This is a key definition for what is to come:

Two sets have the same cardinality if their members can be placed in a one-to-one correspondence. They have the same cardinal number.

In the case of finite sets, this notion is just the familiar notion of the size of the set. For example, the four-membered sets {u, v, x, y} and {1, 2, 3, 4} can be placed in a one-to-one correspondence. They have the same cardinality and the cardinal number 4.

If the members of two sets cannot be placed in such a one-to-one correspondence, then one has a larger cardinality and the other a smaller cardinality.

A smaller cardinality set can be placed in one-to-one correspondence with a proper subset of a larger cardinality set, but not vice versa.

In terms of the representation of sets by areas, the smaller cardinality area can be mapped one-to-one onto a proper subset of the larger cardinality area:

Self-test puzzle: This condition of what it is to be smaller in cardinality is a definition, which means we choose otherwise. What are the alternatives? Are any appealing?

However, there is no expansion of the smaller cardinality area such that it can fill the larger cardinality area fully:

When they disagree

The two criteria agree, as long as we are dealing with finite sets. That makes it easy to treat them as interchangeable. You can check you have the same number of left and right socks by pairing them; or you can count how many left socks you have and how many right socks you have and check if the two counts are the same. Either procedure works and should give the same result, assuming that you have finitely many socks.

They come apart when they are applied to infinite sets. This troubled Leibniz, the great 17th century thinker. He would not compromise the Euclidean axiom: "the whole is greater than the part." He considered the case of numbers and their squares. He recognized correctly that the numbers 1, 2, 3, ... are equinumerous with the square numbers 1²=1, 2²=4, 3²=9, ... He found this equality to be absurd:

As quoted in Mark van Atten. "A note on Leibniz’ argument against infinite wholes." British Journal for the History of Philosophy, 19 (2011), pp.121-129.

"Therefore there are as many numbers as there are square numbers, that is, the number of numbers is equal to the number of squares, the whole to the part, which is absurd."

Stern action was needed to eliminate the absurdity. He concluded that numbers do not form a proper whole, so that the equality in number of the set of numbers and set of square numbers is escaped. This escape is a version of the Aristotelian retreat from actual infinities to potential infinities.

Georg Cantor's Transfinite Arithmetic

"No one should be able to drive us out of the paradise that Cantor created for us." "Aus dem Paradies, das Cantor uns geschaffen, soll uns niemand vertrieben können." p. 170 in David Hilbert, "Über das Unendliche" [On the infinite], Mathematische Annalen 95 (1926): 161–190.

Until the 19th century, comparisons of the sizes of sets was carried out almost exclusively by inclusion. Georg Cantor took up the investigation of the comparisons of the sizes of sets using the criterion of one-to-one correspondence. What resulted was an opening of a whole new realm of mathematics. It is so wonderful in its scope that David Hilbert called it Cantor's "paradise." (Alas, Hilbert's positive reaction was not universal. Cantor faced considerable opposition to his ideas and suffered greatly for it.)

A major result is that not all infinities are alike. Some are bigger than others; and there are always greater infinities to be had. He classified the sizes of the infinities by transfinite numbers, using the Hebrew letter "aleph":

Sets as Numerous as the Natural Numbers

Cardinal Numbers

The set of natural numbers, {1, 2, 3, 4,...} has the cardinality of the first of Cantor's numbers, ℵ₀. ("Aleph-null") This is its cardinal number, which conveys to us its size. Merely saying "infinity" in Cantor's theory is imprecise. They are many infinities. By specifying ℵ₀, we specify which infinity applies.

All of the infinite subsets of the natural numbers have this same cardinality. They include the square numbers, the cubes, ..., prime numbers, multiples of 2, multiples of 10, and so on for many more.

These sets are often called "countably infinite" or "denumerably infinite." That just means that the sets are of a size that we can, metaphorically, count off or number their members by using the natural numbers, 1, 2, 3, ... (The terms "countable" and "denumerable" may also be applied to finite sets, since they can also have their members counted,. There does not seem to be a universal agreement on whether this application to finite sets is a proper use of the terms. This is just a question of how we choose our definitions.)

A cardinal number like ℵ₀ is used in the same way as we use natural numbers to convey the size of a set that is finite in size. The cardinality of the set {1, 2, 3, 4} is 4. That is, its cardinal number is 4. We use ℵ₀ in the same way as we use "4." Here is an example:

What is the size of the set {1, 2, 3, 4}? Answer: "4"
Analogously:
What is the size of the set of natural numbers, {1, 2, 3, ...} Answer: ℵ₀.

Everything in Cantor's theory applies to finite sets as well. For example, the sets {1, 2, 3, 4} and {5, 6, 7, 8} can be placed in a one-to-one correspondence. Therefore they have the same cardinality, 4:

Nothing unexpected happens, however, in the application to finite sets. This finite set cannot be placed into a one-to-one correspondence with any of its proper subsets, such as {5,6}. This last set is smaller in cardinality and has the cardinal number 2.

The integers ℤ

New cases arise when we consider sets of numbers that are larger than the natural numbers by the criterion of inclusion, but are of the same cardinality. Here are two cases. The integers are are all the natural numbers, zero, and the negatives of the natural numbers:

Self-test puzzle: can you find other ways to map the two sets one-to-one?

It should not be a surprise that the natural numbers ℕ and the integers ℤ can be put in a one-to-one correspondence. Here's one way to do it?

Even number in ℕ → -(even number/2) in ℤ
so 4 → -(4/2) = -2
6 → -(6/3) = -3
etc.

Odd number in ℕ → (odd number-1)/2 in ℤ
so 1 → (1-1)/2 = 0
3 → (3-1)/2 = 1
etc.

Cantor introduces ℵ₀

The paper is Georg Cantor, "Beiträge zur Begründung der transfiniten Mengenlehre," Mathematische Annalen, 46 (1896), pp. 481-512.

Here is the original paper from 1896 in which Cantor declares that sets of the size of the natural numbers are given a cardinal number called ℵ₀. He uses the German spelling for "aleph," which is "alef."

The rationals ℚ ...

The more interesting case is the set of all rational numbers ℚ. These are all the numbers that can be written as a ratio of integers, excluding division by zero. They include the obvious fractions like 1/2, -1/2, 0 and 2/3. Also included are improper fractions like 13/7 and 101/5.

At first it seems that there must be many more rational numbers than natural numbers or integers. For if we lay out the natural numbers or the integers on a number line, they have gaps between the numbers:

The rational numbers, however, are dense on the number line. That is, if we pick any two rational numbers:

and so on indefinitely. Each of the gaps between the natural numbers is filled with infinitely many rational numbers.

The rule used here to generate new rational numbers to fill in any gap is this: for any two rationals R₁ and R₂, their average (R₁ + R₂)/2 lies between them and is also a rational number.

For example, if R₁ = 37/4 R₂ = 65/3, then
(R1 + R2)/2 = (3x37 + 4x65)/(3x4) =371/12

Self-test. This is one way to prove density. Is there another way that does not take the average of R₁ and R₂? The geometric mean? What else?

This lets us prove by reductio that there can be no finite sized gaps in the set of rational numbers. For suppose for purposes of reductio there is a gap between say R₁ and R₂, which would mean that there are no rationals between them. Yet (R₁ + R₂)/2 is a rational number that lies between them.

We might try to picture this density if we represent rational numbers by circles on the number line. There is a big gap between 1 and 2. Then a smaller gap between 1 and 1.5 and between 1.5 and 2. Each gap is filled by more rational numbers, so that none remain:

Thus, the rational numbers seem to be so numerous that they escape one-to-one correspondence with the natural numbers. Or at least that is a plausible first guess.

... are equinumerous with the naturals ℕ

Cantor showed otherwise. It turns out that there is a simple scheme that allows us to place the natural numbers in a one-to-one correspondence with the natural numbers. The trick is easiest to see if we start with the positive rationals only. We lay them out in an infinite two-dimensional grid. We can then trace through the entire grid by following the diagonal paths shown. We tag each rational number as we pass it with a natural number. it follows that the positive rationals have the same cardinality as the natural numbers, Cantor's, ℵ₀.

The natural scheme shown has duplications. 1/1, 2/2, 3/3, ... are all the same rational number 1. We could just ignore the duplications, for then the one-to-one mapping would be between a proper subset of the natural numbers and the rational numbers. That is enough to establish that the natural numbers and the positive rational numbers are equinumerous. A slightly more elegant approach is shown in the figure. When we come to a duplicated rational number, we just jump over it and do not tag it.

Self test: can you see how do to this simple exercise?

This procedure sets up the one-to-one mapping between the natural numbers ℕ and the positive rationals. It is then a simple exercise to extend this one-to-one mapping to the full set of rationals ℚ that includes zero and all the negative rationals.

Self-test puzzle: This proof method uses the function 2^m3ⁿ. Could we use other functions? What about m/n is mapped to mxn, so that 2/3 is mapped to 2x3=6?

Answer

This method is the standard method used to display a one-to-one correspondence of the natural numbers and the positive rational numbers. A much faster way to get the same result is to write each positive rational number as m/n. Each is then mapped to 2^m3ⁿ. We know from the unique factorization of composite numbers in arithmetic, that each m/n is thereby mapped to a unique natural number. A one-to-one correspondence is thereby displayed between the natural numbers and a subset of the natural numbers.

1/1 → 2¹3¹ = 6
1/2 → 2¹3² = 18
2/1 → 2²3¹ = 12
etc.

Sets more Numerous than the Natural Numbers. ℝ

It is surprising that the set of rational numbers turns out to be equinumerous with the set of natural numbers. We might well wonder about another set of numbers that is also dense on the real line. These are the real numbers, ℝ. Rational numbers can be expressed as terminating decimals

If we now add into the set all the numbers (positive and negative) with non-repeating decimal expansions, that is, the irrational numbers, we have the set of real numbers:

√2 = 1.4142135623730950488…
π = 3.14159265358979323846264338327950288419716939937510...

The real numbers are also dense on the real line. Between any two real numbers there is always another one; and so on indefinitely.

The demonstration uses a rule similar to the one used with rational numbers. For any two real numbers R₁ and R₂, their average (R₁ + R₂)/2 lies between them and is also a real number. Since the rational numbers are already dense on the number line, we need further argumentation to show that the irrational numbers added are also dense on the number line. The averaging argument does not show it. We can take two irrational numbers and find that their average is a natural number. Consider (10+π) and (10-π). They are both irrational numbers, but their average is 10. To see that the irrational numbers are dense on the number line, take any two, different rational numbers, r₁ and r₂, it is easy to see that there are infinitely many in irrational numbers between them. For example, consider 1.1 and 1.2. The irrational 1.1235687389578... (assumed non-repeating) is one of infinitely many irrational numbers lying between them.

It turns out, however, that the real numbers are more numerous in cardinality than the set of natural numbers. That they are more numerous is shown by one of the most celebrated arguments in Cantor's set theory, "diagonalization." Cantor's argument is a reductio argument. He assumes for purposes of reductio that there is a one-to-one mapping between the natural numbers and a subset of the real numbers lying in the interval (0,1). (If he can show that this subset of real numbers is already too big to be put in one-to-one correspondence with the natural numbers, then the same must true of the bigger set of all real numbers.)

Let us assume that we have found such a one-to-one correspondence. It might look like this:

1 ↔ 0 . 1 4 1 5 9 2 6 ...
2 ↔ 0 . 5 3 5 8 9 7 9 ...
3 ↔ 0 . 3 2 3 8 4 6 2 ...
4 ↔ 0 . 6 4 3 3 8 3 2 ...
5 ↔ 0 . 7 9 5 0 2 8 8 ...
6 ↔ 0 . 4 1 9 7 1 6 9 ...
7 ↔ 0 . 3 9 9 3 7 5 1 ...
...

We consider the digits along the shaded diagonal. These digits form another real number:

Self-test puzzle. Is this increment by one rule the only one we could use?

All we need now to complete the reductio is to find any real number that differs from this one in every digit. We might replace a 1 by 2, a 2 by a 3, ..., a 9 by a 0. then we have

This new number cannot appear anywhere in the one-to-one correspondence assumed:

It cannot correspond to 1, since it differs from real corresponding to 1 in the first digit.
It cannot correspond to 2, since it differs from real corresponding to 2 in the second digit.
It cannot correspond to 3, since it differs from real corresponding to 3 in the third digit.
and so on for all the digits.

That is, we have shown that this assignment of real numbers to natural numbers is not one-to-one. The real number we have constructed is missing from the correspondence. There is nothing special about the particular correspondence suggested. No matter which correspondence we might propose, the diagonalization construction will always produce a real number that eludes it.

The reductio is complete. The assumption that we can have a one-to-one correspondence between the natural numbers and real numbers produces a contradiction. The real numbers form an infinite set larger than the infinite set of the natural numbers. It is natural to assume that this larger set carries Cantor's next cardinal number ℵ₁. However deciding just which sets ought to carry this next cardinal number proves to be a messy matter and will be discussed below under the heading of the "continuum hypothesis."

Since the real numbers form a continuum, such as the continuum of a line in geometry, it is often called "continuum- sized." In contrast, sets of the size of the natural numbers or smaller are called "countable," since we can enumerate their elements with ordinary counting numbers 1, 2, 3, ... Sets of the size of the real numbers, however, would require something as big as the real numbers if an enumeration is to succeed.

The method of diagonalization is one that will return many times. It is an important mode of proof when we explore infinite systems. Its core idea is to turn something against itself and thereby to establish a contradiction.

Cantor's Discovery of Proof by Diagonalization

The paper is Georg Cantor (1891). "Ueber eine elementare Frage der Mannigfaltigkeitslehre" Jahresbericht der Deutschen Mathematiker-Vereinigung. 1, pp. 75–78.

Here is the short paper in which Cantor announces, with obvious satisfaction, that he has discovered a proof of great simplicity ("grosse Einfachheit") that shows that some sets are larger in cardinality than others.

Equinumerous Intervals of Real Numbers.

There are many distinct sets equinumerous with the real numbers. Here are just a few of them.

Consider the closed interval of real numbers [0,1] and [0,2]. That is, all the real numbers lying between 0 and 1, including 0 and 1; and all the real numbers lying between 0 and 2, including 0 and 2. They are equinumerous and of continuum size.

Self-test puzzle: Does this method of proof also enable us to show that all rational numbers between 0 and 1 are equinumerous with all rational numbers between 0 and 2?

The simple proof is to note that each real number r in [0,1] stands in a one-to-one correspondence with each real number 2r in [0,2]. That is, to find out what real number is assigned to any real number, r, just double it:

There are many more examples. Another example concerns the open set of real numbers (0,1), that is, all real numbers between 0 and 1, not including 0 and 1. It can be placed in a one-to-one correspondence with (0,∞), that is all real numbers greater than 0. The correspondence can be brought about in many ways. A simple algebraic rule suffices:

That is,
r=0.01 is mapped to 0.01/(1-0.01) = 0.01/0.99 = .010101....
r = 0.5 is mapped to 0.5/(1 - 0.5) = 1
r= 0.99 is mapped to 0.99/(1-0.99) = 0.99/0.01 = 99

Self-test puzzle: Convince yourself that the map has the following assignments:
1/2 → 1
2/3 → 2
3/4 → 3
4/5 → 4
...
n/(n+1) → n

The geometrical representation lays out the values of r on the right hand, vertical side of the unit square shown in the figure below. A ray from the top left hand corner maps the various values of r to their corresponding values of r/(1/r) on an indefinitely extended horizontal line, aligned with the base of the square:

That this figure represents the mapping r → r/(1-r) follows from a consideration of similar triangles. The figure below redraws a portion of the above geometrical representation. The triangles ABC and CDE are similar. Hence the ratio of the first triangle's sides, AB:BC equals the corresponding ratio of the second triangle's sides, DE:CD. That is
AB:BC = 1/(1-r) = DE:CD = [ r/(1-r) ]/r = 1/(1-r)

The function implemented is not the simple algebraic function just given, but a trigonometric function. r in (0,1) is mapped to tan(rπ/2)

A more traditional way to display the correspondence is with the geometric figure below. It shows how to map a unit interval on the quarter circle to the infinite line of all positive real numbers.

Self-test puzzle: These two geometric representations show two ways that the real numbers in (0,1) can be mapped one-to-one to the real numbers in (0,∞). Can you find more ways to realize the mapping?

These mappings are richer than what is needed to establish mere one-to-one correspondence. They also preserve the order the two sets of numbers mapped. Here order means that "less than" relations are preserved in the mapping. That means that that a richer equivalence is actually being demonstrated. It is not just cardinal equivalence, but what is called "ordinal" (or order preserving) equivalence. This richer concept is not explored here.

The Mapping in the Port Royal Logic

The mapping between finite and infinite intervals of real numbers has a rich history. An early form of it is found in Arnaud and Nicole's La Logique ou l'art de penser [Logic or the Art of Thinking], also known as the "Port Royal Logic." It was a definite treatise on logic and scientific method in the 17th century.

Their map is introduced through the artifice of a ship that leaves port to sail away on an infinitely extended, flat sea. The position of the ship's bottom is viewed from shore. As the ship departs, its position rises in the observers field of view and the projection of it in a lens descends. (In the figure below, I have replaced the lens with a simple viewing screen.) As the ship moves away equal distances, the projection point descends toward an accumulation point. Each step takes less space, shrinking without limit. The infinitely extended sea is mapped onto a finite screen.

Sailboat image from Sailboat image from T. R Biddel, A Treatise on the Construction, Rigging, & Handling of Model Yachts, Ships and Steamers. London: Norrie & Wilson, 1883. p. 35.

Arnaud and Nicole's goal, however, was not to demonstrate the one-to-one correspondence of the points of the infinite sea and the points of the finite screen. That was only shown as an incidental result subordinate to their real goal. They wanted to argue for the infinite divisibility of space. They took as an acceptable premise that a flat sea could in principle always be extended by some fixed distance. The projection points of the newly acquired positions at sea would then correspond to ever smaller displacements on the viewing screen. Since these displacements could become arbitrarily small, the construction shows the infinite divisibility of space.

Antoine Arnauld and Pierre Nicole, Logic or the Art of Thinking. Trans. and ed. Jill Vance Buroker. Cambridge: Cambridge Univ. Press, 1996. p. 232

"Certainly, while we might doubt whether extension can be infinitely divided, at least we cannot doubt that it can be increased to infinity, and that we can join to a surface of a hundred thousand leagues another surface of a hundred thousand leagues, and so on to infinity.[footnote: Descartes, Principles of Philosophy, Pt. II, art. 21, Philosophical Writings, vol. I, p. 232.] Now this infinite increase in extension proves its infinite divisibility. To understand this we only have to imagine a flat sea that is increased infinitely in extent, and a vessel at the shore that leaves the port in a straight line. It is certain that when the bottom of this vessel is viewed from the port through a lens or some other diaphanous body, the ray that ends at the bottom of this vessel will pass through a certain point of the lens, and the horizontal ray will pass through another point of the lens higher than the first. Now as the vessel goes further away, the point of intersection with the lens of the ray ending at the bottom of the vessel will continue to rise, and will divide space infinitely between these two points. The further away the vessel goes, the more slowly it will rise, without ever ceasing to rise. Nor can it reach the point of the horizontal ray, because these two lines that intersect at the eye will never be parallel nor the same line. Thus this example provides at the same time proofs of the infinite divisibility of extension and of an infinite decrease in motion."

Higher Order Infinities

The Biggest Set?

At first, it seemed that all infinite sets are the same size. The natural numbers are the same size in cardinality as the integers, which is the same size in cardinality as the rational numbers. Just when it seems that every infinite set might be the same size, it turned out the real numbers form a set that is larger in cardinality that the natural numbers.

Have we now found in the real numbers the biggest set? Are there sets still bigger in cardinality than the real numbers? If not the reals, might there be a biggest set larger than it? It would seem a simple matter to form such a set. Just to collect together all the sets and form their union. Call it the universal set. It has the property that every set would be a subset of this universal set.

Here is one way to get a sense of why there might be a problem with this universal set. If the universal set has all sets among its subsets, then it is both a member of itself and a subset of itself. That sounds odd. One might be tempted dismiss the oddity as yet another peculiar but ultimately benign result concerning infinite sets. This one, however, turns out not to be benign.

Simple as this idea sounds, it turns out that there can be no set that is biggest in cardinality. For any set we are given, we can always find one that is larger than it in cardinality. This curious circumstance is the starting point of foundational work in set theory that came to dominate the 20th century. We shall see more of it in a chapter to come.

The Power of Power Sets.

Cantor found a simple means of ascending to higher infinities. It will enable us to see that there is no biggest set.

The means is through the notion of a power set. Consider some set, say S = {a, b, c}. Its power set is the set of all its subsets

P(S) = {{a}, {b}, {c}, {a, b}, {a, c}, {b, c}, {a, b, c}, {}}.

This set S has 3 elements. The power set has 2³ = 8 elements. We arrive quickly at this formula once we realize that we can identify each subset of S by answering 3 questions:

Is the first element a in the set? (yes/no)
Is the second element b in the set? (yes/no)
Is the third element c in the set? (yes/no)

Each question can be answered in two ways (yes/no). Since there are 3 questions, the total number of answers is:

The general result is that a set with n elements has a power set with 2ⁿ subsets. We can identify each element of the power set by answering n of these yes/no questions.

The jump from n to 2ⁿ is a big jump. If we now make the jump in the case of infinite sets, we are jumping from one cardinal number to a bigger one.

We can see that this happened in the transition from the natural numbers to real numbers in the following way. Each subset of natural numbers in the power set of the natural numbers can be identified by answering infinitely many questions of the form:

Is 1 in the set? (yes/no)
Is 2 in the set? (yes/no)
Is 3 in the set? (yes/no)
...

What results is an infinite string of yes-no-no-yes-yes-yes-no-yes-... with each string identifying a unique subset of the natural numbers. If we copy the way that we treated the power set of a three-membered set above, we can represent the size of the power set of the natural numbers by multiplying a countable infinite of 2's together:

The resulting formula, "2^ℵ₀," is "two to the power of aleph-null." It is the cardinal number that represents the size of the power set of the natural numbers.

Self-test puzzle: apply this construction to the three-membered set (a, b, c}. Show that eight binary fractions are constructed. Which are they?

We can take this infinite string of yes-no answers and replace each yes by a 1 and each no by a 0. We can use the resulting string of 1's and 0's to form a real number such as:

If we read this as a base-2 binary number, it is a real number between 0 and 1. All the possible encodings of the answers exhaust the real numbers between 0 and 1. The overall effect is that we have shown that all the subsets of the natural numbers can be mapped one-to-one to the real numbers between 0 and 1. This set, we saw above, is larger than the set of natural numbers and is of continuum size. That is, the cardinality of the set of real numbers is

Cantor's Theorem

We have seen examples above of how taking a power set carries us to a set of higher cardinality. Cantor's theorem tells us that this is a quite general result. Take the power set of some set and always end up with a set of higher cardinality:

Cantor's Theorem: The power set of any set is strictly larger than the set and cannot be placed in a one-to-one correspondence with the set.

This assertion is of foundational importance in investigations of infinity. It tells us that we can always find a larger infinity by taking the power set of the set presently under consideration. What results is an infinity of infinities, each larger in cardinality than the one before. If the infinite set is S, then the ascent arises as:

The theorem turns out to be rather simple to prove. The proof is another diagonalization. We proceed with an assumption for reductio. Let us say that we have found a one-to-one correspondence "f" between some set S = {a, b, c, ...} and its power set P(S) = { {}, {a}, {a b}, ...} There is no presumption on the size of S. It can be infinite of any order. The one-to-one correspondence might look something like this:

Listing the elements like this sequentially will only be possible if the original set S is countable. This listing is only an expository convenience. The proof does not require that S be countable.

a ↔ f(a) = {a, b, c}
b ↔ f(b) = {a, c, d, e, ...}
c ↔ f(c) = {e, f, g, ...}
and so on for all elements of S.

a is an element of Diag, exactly in case it is not an element f(a).
b is an element of Diag, exactly in case it is not an element f(b).
and so on for all elements of S.

f(a) = {a, b, c}                     so a is NOT in Diag
f(b) = {a, c, d, e, ...}        so b IS in Diag
f(c) = {e, f, g, ...}              so c IS in diag
        and so on.

With this diagonal set defined, we quickly arrive at a contradiction. The set Diag is a subset of S and thus should be an element of the power set P(S). Therefore it should appear somewhere in the one-to-one correspondence. Yet by its construction it cannot be the set to which any element of S is mapped.

It cannot be f(a), since by construction Diag has a as an element exactly if f(a) does not.
It cannot be f(b), since by construction Diag has b as an element exactly if f(b) does not.
and so on for all the remaining elements of S.

Self-test puzzle: the argument is a reductio ad absurdum. Exactly which hypothesis is set up for refutation? A reductio argument only shows that something in the assumptions is wrong. Is it possible to use the reductio as a refutation of a different hypothesis?

The reductio is complete. The assumption made for reductio is refuted. The elements of any set S cannot be mapped one-to-one onto its power set P(S). The power set is an order of infinity larger.

The Continuum Hypothesis

Cantor's transfinite arithmetic supposes the existence of cardinal numbers of ever increasing size. We might write them as a sequence:

There will be no largest cardinal number. For any set, Cantor's theorem assures us that there is always a larger set, its power set, and that larger set will have a larger cardinal number.

It would be natural to assume that the cardinal number of the set of real numbers, 2^ℵ₀, is the the next highest cardinal number after the cardinal numbers of the set of natural numbers, ℵ₀. It seems quite plausible that this is so. This plausible supposition has a name, "the continuum hypothesis." It asserts that the next highest cardinal number after the ℵ₀ of the natural numbers is the cardinal number of the continuum, 2^ℵ₀. We can write the hypothesis symbolically as:

The hypothesis would be false if it turned out that the cardinal number 2^ℵ₀ is actually equal to a larger cardinal number such as ℵ₂ or even ℵ₃. It seems a simple matter to check the hypothesis. We would look for a set larger than the set of natural numbers but smaller than the set of real numbers, where that set is larger in cardinality than the natural numbers and smaller in cardinality than the set of real numbers. This check is simple enough to state and the outcome known. No one has been able to find a set of intermediate cardinality.

That, alas, does not decide the matter. That no one can find such a set does not mean that such a set does not exist. Perhaps we just have not been diligent enough in our search. Or, more plausibly, we finite humans have limited methods of constructing sets through manipulation of finitely many symbols. Once we are in the world of infinite sets, might the set we seek simply be something that outstrips our meager powers of description?

The worst case for us would be that the truth of the continuum hypothesis just is something outside our demonstrative powers. It might be true; or it might be false. But our demonstrative powers are unable to decide. This worst case is the one we have. We can describe this worst case is more precise terms as follows. Take our best theory of sets and add either the continuum hypothesis or its negation to its axioms. Either case proves to be consistent. That is, we can tell a consistent story of infinite sets in which the continuum hypothesis is true; and we can tell a consistent story of infinite sets in which it is false.

How we should think about this curious outcome goes well beyond what can be treated here. Perhaps we can say this much. If we think that infinite sets exist independently of our theories, then we have learned that our theories are too weak to grasp them fully. If we think that infinite sets are conjured into being by our theories, then once again those theories are unable to find a principled reason to choose a system in which the continuum hypothesis is true as opposed to one in which it is false. We can just choose which we want.

Ross-Littlewood Urn Supertask.

The Supertask Described

A now standard account of this supertask is in pp. 46-47 of Sheldon M. Ross, A First Course in Probability. 8th ed. Prentice-Hall.

This curious supertask combines issues to do with supertasks with issues to do with infinite sets. We are to imagine a countable infinity of balls numbered 1, 2, 3, ... and an urn of limitless capacity. In the span of time from one minute to midnight ( time "-1") to midnight (time "0") we undertake the following supertask:

At time -1, we place balls 1 to 10 in the urn and remove ball 1.
At time -1/2, we place balls 11 to 20 in the urn and remove ball 2.
At time -1/3, we place balls 21 to 30 in the urn and remove ball 3.
At time -1/4, we place balls 31 to 40 in the urn and remove ball 4.
and so on until time 0.

The supertask continues similarly with the next ten balls. Stage 2, initiated at time -1/2, begins with

The supertask continues through the remaining stages 3, 4, 5 and so on infinitely.

The Question

There is an almost irresistible answer: At each stage, we put 10 balls into the urn and removed one. So at each stage the urn gains a net of 9 balls. There are infinitely many stages at times -1, -1/2, -1/3, ... so the urn must have infinitely many balls in it at midnight.

Tempting as this answer is, it turns out to be wrong. What reveals the error is another question: Which balls are in the urn at midnight? Each ball has a number, so if there is a ball in the urn we must be able to identify its number. We quickly see that there are none:

At time -1, ball 1 was removed.
At time -1/2, ball 2 was removed.
At time -1/3, ball 3 was removed.
At time -1/4, ball 4 was removed.
and so on for all balls.

Thus, at midnight, there can be no numbered ball in the urn. Since every ball has a number, it follows that there can be no balls in the urn.

As the supertask progresses, we see that the set of balls in the urn is eaten away, one by one, starting at the smallest number and working up through the entirety of the numbers. At any finite stage prior to midnight, however, the urn will contain a large number of balls. As midnight approaches, the number of balls in the urn will exceed any fixed number we care to name.

More than 100? At stage -1/100, there are 9x10 = 900 balls in the urn.
More than 1,000? At state -1/1,000, there are 9x1,000 = 9,000 balls in the urn.
and so on for any number we can name.

Subtracting Infinity from Infinity

What this supertask illustrates is an oddity of infinite cardinal arithmetic. We are used to simple subtractions in arithmetic:

However if we are subtracting an infinity from an infinity, the result is underspecified:

If the infinity is the set of natural numbers, we can take away the infinite set of even numbers and be left with an infinity, the odd numbers:

Or we might just subtract all numbers greater than 10 and be left with a finite set of just ten numbers:

Self-test puzzle: Before you look at the later chapter, what are schedules of removals that might leave only odd numbered balls in the urn? Or just balls numbered 1 to 10? Or something else?

The particular schedule specified in the urn supertask determines which of these or many other subtractions are implemented. The schedule in the supertask above implements this last subtraction. In a later chapter, the urn supertask is investigated further. There we will see how other schedules of removals lead to different numbers of balls remaining in the urn at the conclusion of the supertask.

To Ponder

Can you show that all natural numbers {1, 2, 3, 4, 5, ...} can be placed in one-to-one correspondence with the set of all integers {... -2, -1, 0, 1, 2, 3, ...}?

Can you show that all positive rational numbers, {1, 1/2, 2, 1/3, 2/3, ...} can be placed in one-to-one correspondence with the set of all positive and negative rational numbers, {1, 1/2, 2, 1/3, 2/3, ... -1, -1/2, -2, -1/3, -2/3, ...}?

Can you show that the set of all pairs of natural numbers {<1,1>, <1,2>, <1,3>, ..., <2,1>, <2,2>, ...} can be placed in one-to-one correspondence with the natural numbers?

Can you show that the set of all finite subsets of natural numbers can be placed in one-to-one correspondence with the natural numbers?
(Hint: The prime numbers are 2, 3, 5, 7, ... and each composite number can be written uniquely as a product of prime numbers. e.g. 5,445,468 = 2² x 3⁴ x 7⁵.)

Can you show that the set of infinite subsets of the natural numbers can be placed in one-to-one correspondence with the natural numbers?
(Hint: diagonalization?)

Can you find variants of the Ross-Littlewood Urn Supertask that leaves the urn at the end of the supertask filled with infinitely many balls; or just filled with 10 balls.

June 24, September 18, 27, October 6, December 7, 2021. December 21, 2022. February 18, 2023.

1	2	3	4	5	6	7	8	9	...
↕	↕	↕	↕	↕	↕	↕	↕	↕
2	4	6	8	10	12	14	16	18	...
↕	↕	↕	↕	↕	↕	↕	↕	↕
1²=1	2²=4	3²=9	4²=16	5²=25	6²=36	7²=49	8²=64	9²=81	...

1	2	3	4	5	6	7	8	9	...
↕	↕	↕	↕	↕	↕	↕	↕	↕
0	-1	1	-2	2	-3	3	-4	4	...

Time	Balls in urn	Count of balls in urn	Balls removed
-1	1, 2, 3, 4, 5, 6, 7, 8, 9, 10	1x9 = 9	1
-1/2	1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15 16, 17, 18, 19, 20	2x9 = 18	1, 2
-1/3	1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, 28, 29, 30	3x9 = 27	1, 2, 3
...	...	...	...
0	1, 2, 3, 4, 5, 6, 7, 8, 9, 10, ...	0	1, 2, 3, 4, 5, 6, 7, 8, 9, 10, ...