We now turn our attention to a brief description of
periodically forced and coupled neural oscillators.
The behaviors generally involve issues that are very difficult to analyze
and we will only touch on them briefly.
Before treating a specific example, it is useful to discuss
certain important aspects of oscillators. We say that a periodic solution to an
autonomous (time does not explicitly appear in the right hand side) differential
equation is ( orbitally) asymptotically stable if perturbations from the
oscillation return to the oscillation as 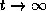 . The difference
between asymptotic stability of an oscillation and that of a steady state
solution is that for the oscillation the time course may exhibit a shift. That
is, we do not expect the solution of the perturbed oscillation to be the same as
the unperturbed, rather there will be a shift (see Figure 12A).
. The difference
between asymptotic stability of an oscillation and that of a steady state
solution is that for the oscillation the time course may exhibit a shift. That
is, we do not expect the solution of the perturbed oscillation to be the same as
the unperturbed, rather there will be a shift (see Figure 12A).
Figure 12a
Integrate the equation and freeze the curve so you have a reference
cycle. Use the (Graphic stuff) (Freeze) (Freeze) option to freeze
it. Now it is permanently in memory. To see the effect of a
perturbation, set s0=300 and reintegrate. Not much effect,
huh? The parameter ph determines where in the cycle the
perturbation occurs. Try ph=40 and integrate again. There is
a big phase shift.
This shift is
due to the time-translation invariance of the periodic solution. Indeed, in
phase-space, the periodic trajectory is unchanged by translation in time. This
shift that accompanies the perturbation of the limit cycle can be exploited in
order to understand the behavior of the oscillator under external forcing.
Suppose that an oscillator has a period, say T. We may let t=0 correspond
to the time of peak value of one of the oscillating variables, so that at t=T
we
are back to the peak. Given that we lie on the periodic solution, if
some t is
specified then we know precisely the state of each oscillating variable. This
allows us to introduce the notion of phase of the periodic solution. Let
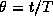 define the phase of the periodic solution so that
define the phase of the periodic solution so that 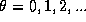 all define the same point on the periodic solution. For example, if
all define the same point on the periodic solution. For example, if
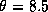 then we are halfway through the oscillator's ninth cycle.
then we are halfway through the oscillator's ninth cycle.


Next: Phase-response curves
Up: No Title
Previous: Parabolic Bursting: Two
Bard Ermentrout
Mon Jul 29 17:47:46 EDT 1996
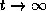 . The difference
between asymptotic stability of an oscillation and that of a steady state
solution is that for the oscillation the time course may exhibit a shift. That
is, we do not expect the solution of the perturbed oscillation to be the same as
the unperturbed, rather there will be a shift (see Figure 12A).
. The difference
between asymptotic stability of an oscillation and that of a steady state
solution is that for the oscillation the time course may exhibit a shift. That
is, we do not expect the solution of the perturbed oscillation to be the same as
the unperturbed, rather there will be a shift (see Figure 12A).
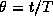 define the phase of the periodic solution so that
define the phase of the periodic solution so that 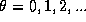 all define the same point on the periodic solution. For example, if
all define the same point on the periodic solution. For example, if
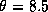 then we are halfway through the oscillator's ninth cycle.
then we are halfway through the oscillator's ninth cycle.