


Next: More Bistability
Up: Understanding Dynamics via
Previous: Oscillations emerging with
The Hopf bifurcation is one of a few generic mechanisms for the onset of
oscillations in nonlinear differential equation models.
In that case, the frequency at onset of
repetitive activity has a well-defined, non-zero minimum.
In contrast, some membranes and models (see, e.g. [4]) exhibit zero
(i.e., arbitrarily low) frequency as they
enter the oscillatory regime of behavior; Rall's model (see [14]) also
behaves this way. A basic feature in such systems is
that  versus V is N-shaped rather than monotonic as in the previous
section. For equations (4)-(6) this
occurs if the V-dependence of
versus V is N-shaped rather than monotonic as in the previous
section. For equations (4)-(6) this
occurs if the V-dependence of  -activation is translated rightward
(see Appendix A , and note value of
-activation is translated rightward
(see Appendix A , and note value of 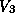 ) so
that the inward component of
) so
that the inward component of  dominates over an intermediate V-range.
Thus, for some values of I, below the repetitive firing range, there are
three singular points in the phase plane. We discuss this case, when the system
is excitable, first.
dominates over an intermediate V-range.
Thus, for some values of I, below the repetitive firing range, there are
three singular points in the phase plane. We discuss this case, when the system
is excitable, first.
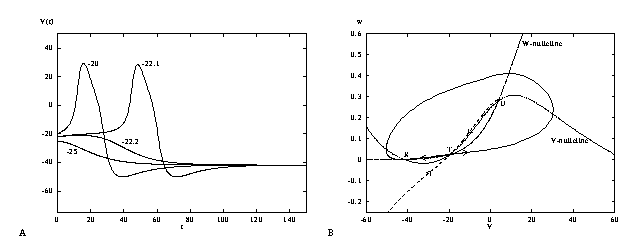
Figure 4
Try a few different initial voltages to find the threshold. Then set
everything to rest, (V,w) = (-41.84,0.002). Plot the nullclines and
use the (sing pt) command to find the unstable saddle point. Have XPP
plot the invariant manifolds to get figure 4B. Plot V vs t.
Try to find a current stimulus
like in Figure 1 that elicits a spike.
Since there is a bias current for this system it should be less than
in Fig 1.
In Figure 4B we see the nullclines intersecting three
times. As determined by linear stability theory, the singular points
are the stable rest state ( R), an unstable saddle-point
threshold ( T), and an unstable spiral ( U).
The system is excitable with the lower
state being a globally attracting rest state: initial conditions near
R lead
to a prompt decay to rest, while larger stimuli lead to an action potential - a
long trajectory about the phase plane. The phase plane portrait moreover
reveals that this case of excitability indeed has a distinct threshold which is
due to the presence of the saddle point, T.
To understand this we note that
associated with the saddle are a unique pair of incoming trajectories
(bold dashed lines) corresponding to the negative
eigenvalue of the Jacobian matrix;
together these represent the stable manifold. Corresponding to the
positive
eigenvalue are a pair of trajectories (bold lines) that enter the saddle
as  ; these are the unstable manifold.
XPP has
a command which generates these manifolds. The stable manifold defines a
separatrix curve in the phase plane which sharply distinguishes sub- from
super-threshold initial conditions. For initial conditions near the
threshold separatrix there is a long latency before firing or decaying as a
subthreshold response (see Figure 4A).
This is because the trajectory starts close to (but not exactly on) the stable
manifold and so the solution comes very near the saddle singular point (where it
moves very slowly) before taking off. If w is started at rest,
; these are the unstable manifold.
XPP has
a command which generates these manifolds. The stable manifold defines a
separatrix curve in the phase plane which sharply distinguishes sub- from
super-threshold initial conditions. For initial conditions near the
threshold separatrix there is a long latency before firing or decaying as a
subthreshold response (see Figure 4A).
This is because the trajectory starts close to (but not exactly on) the stable
manifold and so the solution comes very near the saddle singular point (where it
moves very slowly) before taking off. If w is started at rest,  , then
there is a unique value of
, then
there is a unique value of 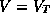 (between -22.1 and -22.2 mV in the present
example) where the stable manifold intersects the line
(between -22.1 and -22.2 mV in the present
example) where the stable manifold intersects the line  This is the voltage threshold.
This is the voltage threshold.
The action potential trajectory
follows along the unstable manifold (bold lines)
which passes around the unstable
spiral and eventually tends to the rest point.
Such a trajectory which joins two singular points is called a heteroclinic
orbit. The other branch of the unstable manifold is also a heteroclinic orbit
from the saddle to the rest point. This heteroclinic pair forces any trajectory
which begins outside it to remain outside it - thus preserving the amplitude of
the action potential. In this case we do not find graded responses for any
brief current pulses from the rest state.
This case also provides a counterexample to a common misconception in which it
is believed that if there are three steady states then the ``outer'' two are
stable while the ``middle'' one is unstable. In fact, in some parameter regimes
this model has three singular points, none of which is stable.
Next we tune up I and ask when repetitive firing occurs. Because 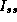 is
N-shaped we know that the lower and middle
is
N-shaped we know that the lower and middle 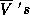 move toward each other as
I increases, and there is a critical value
move toward each other as
I increases, and there is a critical value 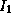 where they meet. In the
phase plane, this means that the rest point and the saddle coalesce and then
disappear; this is called a saddle-node bifurcation. Moreover, the
heteroclinic pair become a single closed loop, a limit cycle, which for I just
above
where they meet. In the
phase plane, this means that the rest point and the saddle coalesce and then
disappear; this is called a saddle-node bifurcation. Moreover, the
heteroclinic pair become a single closed loop, a limit cycle, which for I just
above 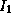 has very long period
(Figure 5).
has very long period
(Figure 5).
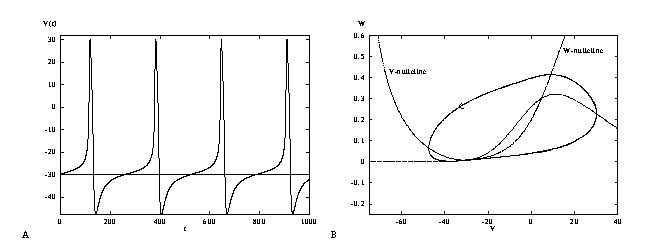
Figure 5
Change the current to 40.76 (just past criticality) and integrate the
equations for 1000 msec. Draw the nullclines and look at V vs t.
Thus, in this parameter regime,
the transition to repetitive firing is marked by arbitrarily low frequency
(Figure 6B). For I near the critical current, the frequency is proportional
to 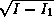 ([34]).
When
([34]).
When 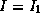 the limit cycle has infinite period; it
is called a saddle-node loop or SNIC
(saddle-node on an invariant circle).
Generally, an infinite period
limit cycle is called a
homoclinic orbit, one that begins and ends at a singular point.
The saddle-node loop is one type of homoclinic orbit; we will
encounter another type in the next section.
This type of zero-frequency onset is generic and occurs
over a range of parameters.
Changing another parameter will typically lead to a smooth change in
the limit cycle has infinite period; it
is called a saddle-node loop or SNIC
(saddle-node on an invariant circle).
Generally, an infinite period
limit cycle is called a
homoclinic orbit, one that begins and ends at a singular point.
The saddle-node loop is one type of homoclinic orbit; we will
encounter another type in the next section.
This type of zero-frequency onset is generic and occurs
over a range of parameters.
Changing another parameter will typically lead to a smooth change in 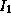 .
We emphasize that this mechanism allows arbitrarily low firing
rates without relying on channel gating kinetics which are necessarily slow.
Such low rates have been associated with the inactivating potassium
A-type current ([4]) although the underlying mathematical
structure of the saddle-node loop does not, of course, require an A-current
([32]). The fast spike dynamics in several recent models
(e.g., [35]) for
cortical pyramidal cells have this same zero-frequency onset of
repetitive firing (unpublished observations by the authors).
The value
.
We emphasize that this mechanism allows arbitrarily low firing
rates without relying on channel gating kinetics which are necessarily slow.
Such low rates have been associated with the inactivating potassium
A-type current ([4]) although the underlying mathematical
structure of the saddle-node loop does not, of course, require an A-current
([32]). The fast spike dynamics in several recent models
(e.g., [35]) for
cortical pyramidal cells have this same zero-frequency onset of
repetitive firing (unpublished observations by the authors).
The value 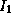 is determined by evaluating
is determined by evaluating 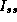 at the value of V for which
at the value of V for which
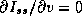 , and this latter condition is equivalent to
having the determinant ad-bc of the Jacobian matrix equal zero.
, and this latter condition is equivalent to
having the determinant ad-bc of the Jacobian matrix equal zero.
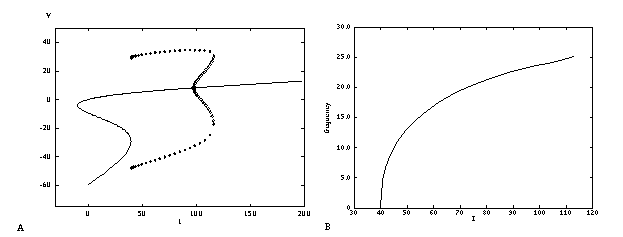
Figure 6
Change the current, I=0 and set the voltage and recovery to
rest, V=-59.474, w=0.00. Run AUTO and set the AUTO axes to run from
-30 to 200 along the x-axis and from -70 to 50 along the y-axis. In
the AUTO Numerics window make DSMAX=5, Par Min=-30, Par Max=200
and run from the steady state. Grab the Hopf bifurcation and
trace the periodic orbit. Plot frequency as a function of the
parameter. (Use the (Axes) (Frequency)
option followed by the (Axes) (Fit) )
Make sure you use the (File) (Reset Diagram) when done.
The global picture of repetitive firing is shown in the bifurcation diagram of
Figure 6A, with frequency versus I in Figure 6B. The branch of steady states
(unstable shown dashed) form the S-shaped curve, and the oscillatory solutions
are represented by the forked curve whose open end begins at 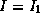 .
As I increases beyond
.
As I increases beyond 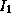 the peak-to-peak amplitude on the
stable (repetitive firing) branch decreases
and the frequency increases. The family of periodic solutions terminates
at
the peak-to-peak amplitude on the
stable (repetitive firing) branch decreases
and the frequency increases. The family of periodic solutions terminates
at 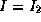 via a subcritical Hopf bifurcation. Except for I in a small
interval of this upper range, this system is monostable. Annihilation of
repetitive firing as in Figure 3 cannot be carried out for I near
via a subcritical Hopf bifurcation. Except for I in a small
interval of this upper range, this system is monostable. Annihilation of
repetitive firing as in Figure 3 cannot be carried out for I near  in
this case. (However, at the high-current end where there is
bistability, annihilition can occur.)
in
this case. (However, at the high-current end where there is
bistability, annihilition can occur.)


Next: More Bistability
Up: Understanding Dynamics via
Previous: Oscillations emerging with
Bard Ermentrout
Mon Jul 29 17:47:46 EDT 1996
 versus V is N-shaped rather than monotonic as in the previous
section. For equations (4)-(6) this
occurs if the V-dependence of
versus V is N-shaped rather than monotonic as in the previous
section. For equations (4)-(6) this
occurs if the V-dependence of  -activation is translated rightward
(see Appendix A , and note value of
-activation is translated rightward
(see Appendix A , and note value of 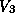 ) so
that the inward component of
) so
that the inward component of  dominates over an intermediate V-range.
Thus, for some values of I, below the repetitive firing range, there are
three singular points in the phase plane. We discuss this case, when the system
is excitable, first.
dominates over an intermediate V-range.
Thus, for some values of I, below the repetitive firing range, there are
three singular points in the phase plane. We discuss this case, when the system
is excitable, first.

 ; these are the unstable manifold.
XPP has
a command which generates these manifolds. The stable manifold defines a
separatrix curve in the phase plane which sharply distinguishes sub- from
super-threshold initial conditions. For initial conditions near the
threshold separatrix there is a long latency before firing or decaying as a
subthreshold response (see Figure 4A).
This is because the trajectory starts close to (but not exactly on) the stable
manifold and so the solution comes very near the saddle singular point (where it
moves very slowly) before taking off. If w is started at rest,
; these are the unstable manifold.
XPP has
a command which generates these manifolds. The stable manifold defines a
separatrix curve in the phase plane which sharply distinguishes sub- from
super-threshold initial conditions. For initial conditions near the
threshold separatrix there is a long latency before firing or decaying as a
subthreshold response (see Figure 4A).
This is because the trajectory starts close to (but not exactly on) the stable
manifold and so the solution comes very near the saddle singular point (where it
moves very slowly) before taking off. If w is started at rest, 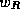 , then
there is a unique value of
, then
there is a unique value of 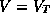 (between -22.1 and -22.2 mV in the present
example) where the stable manifold intersects the line
(between -22.1 and -22.2 mV in the present
example) where the stable manifold intersects the line  This is the voltage threshold.
This is the voltage threshold.
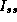 is
N-shaped we know that the lower and middle
is
N-shaped we know that the lower and middle 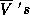 move toward each other as
I increases, and there is a critical value
move toward each other as
I increases, and there is a critical value 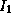 where they meet. In the
phase plane, this means that the rest point and the saddle coalesce and then
disappear; this is called a saddle-node bifurcation. Moreover, the
heteroclinic pair become a single closed loop, a limit cycle, which for I just
above
where they meet. In the
phase plane, this means that the rest point and the saddle coalesce and then
disappear; this is called a saddle-node bifurcation. Moreover, the
heteroclinic pair become a single closed loop, a limit cycle, which for I just
above 
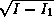 ([
([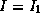 the limit cycle has infinite period; it
is called a saddle-node loop or SNIC
(saddle-node on an invariant circle).
Generally, an infinite period
limit cycle is called a
homoclinic orbit, one that begins and ends at a singular point.
The saddle-node loop is one type of homoclinic orbit; we will
encounter another type in the next section.
This type of zero-frequency onset is generic and occurs
over a range of parameters.
Changing another parameter will typically lead to a smooth change in
the limit cycle has infinite period; it
is called a saddle-node loop or SNIC
(saddle-node on an invariant circle).
Generally, an infinite period
limit cycle is called a
homoclinic orbit, one that begins and ends at a singular point.
The saddle-node loop is one type of homoclinic orbit; we will
encounter another type in the next section.
This type of zero-frequency onset is generic and occurs
over a range of parameters.
Changing another parameter will typically lead to a smooth change in 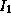 .
We emphasize that this mechanism allows arbitrarily low firing
rates without relying on channel gating kinetics which are necessarily slow.
Such low rates have been associated with the inactivating potassium
A-type current ([
.
We emphasize that this mechanism allows arbitrarily low firing
rates without relying on channel gating kinetics which are necessarily slow.
Such low rates have been associated with the inactivating potassium
A-type current ([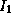 is determined by evaluating
is determined by evaluating 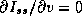 , and this latter condition is equivalent to
having the determinant ad-bc of the Jacobian matrix equal zero.
, and this latter condition is equivalent to
having the determinant ad-bc of the Jacobian matrix equal zero.

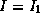 .
As I increases beyond
.
As I increases beyond 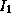 the peak-to-peak amplitude on the
stable (repetitive firing) branch decreases
and the frequency increases. The family of periodic solutions terminates
at
the peak-to-peak amplitude on the
stable (repetitive firing) branch decreases
and the frequency increases. The family of periodic solutions terminates
at 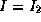 via a subcritical Hopf bifurcation. Except for I in a small
interval of this upper range, this system is monostable. Annihilation of
repetitive firing as in Figure 3 cannot be carried out for I near
via a subcritical Hopf bifurcation. Except for I in a small
interval of this upper range, this system is monostable. Annihilation of
repetitive firing as in Figure 3 cannot be carried out for I near  in
this case. (However, at the high-current end where there is
bistability, annihilition can occur.)
in
this case. (However, at the high-current end where there is
bistability, annihilition can occur.)