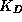for the calcium dependent potassium conductance, equivalent
to decreasing 
,
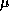can switch the membrane into a repetitively firing regime.
The transition between the bursting and repetitive regimes is very
complicated. For example, when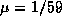 
the burst pattern
has period 4, that is every fourth burst is the same.
When 
, behavior is aperiodic
(time course not shown) whose dynamics can be described as follows.
Each time that V passes a given value, here
when V decreases through 0 mV,
we record the concentration of calcium, as well as the value of w.
For this particular model, the recorded values of w
are all about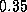 
.
However, the
value of calcium varies between 18.7 and 20.8. The solution
is approximately represented by the
time series of values for the calcium, 
Here's how we can generate the one-variable dynamic rule
whose solutions approximate these time series.
With initial conditions
V=0 and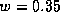 
, we specify a value for calcium, and then
integrate the full differential equations until V crosses 0 again
getting the next value of calcium. Thus we have a map taking a
value of calcium, Ca to a new value of calcium,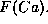 
This map
is called a Poincare Map. The entire dynamics of our burster
is captured by this simple map. For 
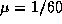this map is shown in
Figure 9c. From the figure, it is evident that there is an
intersection of the line y=x and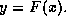 
That means that there is
a single concentration of calcium,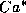 
, to which the trajectory
returns after one cycle. This corresponds to a periodic solution
to the model equations. If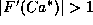 
(as is the case here) the
periodic solution is unstable. This type of map is characteristic of
dynamics that have chaotic behavior. That is, the successive
values of calcium appear to be random and aperiodic. By reducing the
three-dimensional differential equation to a simple one-dimensional
iteration, we can understand the essence of the transition from constant
repetitive firing to chaos as we vary a parameter, say 
.
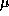More details on one-dimensional maps and chaos can be found in [13],
and for an application to a neuronal bursting system see
[17].